Inscribed Angles - PowerPoint PPT Presentation
1 / 17
Title:
Inscribed Angles
Description:
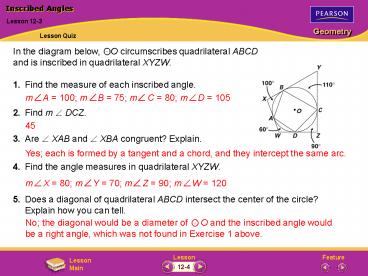
Inscribed Angles Lesson 12-3 Lesson Quiz In the diagram below, O circumscribes quadrilateral ABCD and is inscribed in quadrilateral XYZW. 1. Find the measure of each ... – PowerPoint PPT presentation
Number of Views:165
Avg rating:3.0/5.0
Title: Inscribed Angles
1
Inscribed Angles
Lesson 12-3
Lesson Quiz
In the diagram below, O circumscribes
quadrilateral ABCD and is inscribed in
quadrilateral XYZW. 1. Find the measure of each
inscribed angle. 2. Find m ? DCZ. 3. Are ? XAB
and ? XBA congruent? Explain. 4. Find the angle
measures in quadrilateral XYZW.
45
Yes each is formed by a tangent and a chord, and
they intercept the same arc.
5. Does a diagonal of quadrilateral ABCD
intersect the center of the circle? Explain how
you can tell.
12-4
2
Angle Measures and Segment Lengths
Lesson 12-4
Check Skills Youll Need
(For help, go to Lessons 12-1 and 12-3.)
Check Skills Youll Need
12-4
3
Angle Measures and Segment Lengths
Lesson 12-4
Check Skills Youll Need
Solutions
1. mDE m DCE 57 2. mAED m ACD
180 3. mEBD 360 m DCE 360 57
303 4. Since radii of the same circle are
congruent, ACE is isosceles and m ACE
180 57 123. The sum of the angles of a
triangle is 180 and the base angles of an
isosceles triangle are congruent, so 123 2m
EAD 180 2m EAD 180 123 57 m
EAD 57 2 28.5 5. From Exercise 4, m
AEC m EAD 28.5 6. Since all radii of the
same circle are congruent, CE CD 4.
12-4
4
Angle Measures and Segment Lengths
Lesson 12-4
Check Skills Youll Need
Solutions (continued)
7. By Theorem 11-3, two tangents tangent to a
circle from a point outside the circle are
congruent, so DF EF 2. 8. Draw CF. Since FE
is tangent to the circle, FE CE . By def. of
, CEF is a right angle. By def. of right
angle, m CEF 90, so CEF is a right
triangle. From Exercise 6, CE 4. Also, EF
2. Use the Pythagorean Theorem a2 b2 c2
CE 2 EF 2 CF 2 42 22 CF 2
16 4 CF 2 20 CF 2 CF 20 2 5
4.5 9. CEFD is a quadrilateral, so the sum
of its angles is 360. From Exercise 8, m ACE
90. Similarly, m CDF 90. Also, m DCE
57. So, m EFD m FEC m DCE m
CDF 360 m EFD 90 57 90 360 m
EFD 237 360 m EFD 360 237 123
12-4
5
Angle Measures and Segment Lengths
Lesson 12-4
Notes
A secant is a line that intersects a circle at
two points.
12-4
6
Angle Measures and Segment Lengths
Lesson 12-4
Notes
12-4
7
Angle Measures and Segment Lengths
Lesson 12-4
Notes
12-4
8
Angle Measures and Segment Lengths
Lesson 12-4
Notes
12-4
9
Angle Measures and Segment Lengths
Lesson 12-4
Notes
12-4
10
Angle Measures and Segment Lengths
Lesson 12-4
Notes
12-4
11
Angle Measures and Segment Lengths
Lesson 12-4
Additional Examples
Finding Angle Measures
Find the value of the variable.
a.
x 88 Simplify.
12-4
12
Angle Measures and Segment Lengths
Lesson 12-4
Additional Examples
(continued)
Quick Check
76 x Multiply each side by 2.
12-4
13
Angle Measures and Segment Lengths
Lesson 12-4
Additional Examples
Real-World Connection
12-4
14
Angle Measures and Segment Lengths
Lesson 12-4
Additional Examples
72 180 x Distributive Property
x 72 180 Solve for x.
x 108
Quick Check
A 108 arc will be in the advertising agencys
photo.
12-4
15
Angle Measures and Segment Lengths
Lesson 12-4
Additional Examples
Finding Segment Lengths
5 x 3 7 Along a line, the product of the
lengths of two segments from a point to a
circle is constant (Theorem 12-12 (1)).
5x 21 Solve for x.
x 4.2
8(y 8) 152 Along a line, the product of the
lengths of two segments from a point to a
circle is constant (Theorem 12-12 (3)).
8y 64 225 Solve for y.
8y 161
Quick Check
y 20.125
12-4
16
Angle Measures and Segment Lengths
Lesson 12-4
Additional Examples
Real-World Connection
Quick Check
Because the radius is 125 ft, the diameter is 2
125 250 ft.
The length of the other segment along the
diameter is 250 ft 50 ft, or 200 ft.
x x 50 200 Along a line, the product of the
lengths of the two segments from a point to a
circle is constant (Theorem 12-12 (1)).
x2 10,000 Solve for x.
x 100
The shortest distance from point A to point B is
200 ft.
12-4
17
Angle Measures and Segment Lengths
Lesson 12-4
Lesson Quiz
a 60 b 28
82
15.5
24
22
12-4