Uniform Circular Motion, Acceleration

1
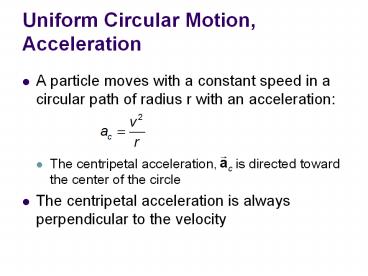
Uniform Circular Motion, Acceleration

• A particle moves with a constant speed in a
  circular path of radius r with an acceleration
• The centripetal acceleration, is directed
  toward the center of the circle
• The centripetal acceleration is always
  perpendicular to the velocity

2
Uniform Circular Motion, Force

• A force, , is associated with the centripetal
  acceleration
• The force is also directed toward the center of
  the circle
• Applying Newtons Second Law along the radial
  direction gives

3
Uniform Circular Motion, cont

• A force causing a centripetal acceleration acts
  toward the center of the circle
• It causes a change in the direction of the
  velocity vector
• If the force vanishes, the object would move in a
  straight-line path tangent to the circle
• See various release points in the active figure

4
Motion in a Horizontal Circle

• The speed at which the object moves depends on
  the mass of the object and the tension in the
  cord
• The centripetal force is supplied by the tension
• Tmv2/r hence

5
Motion in Accelerated Frames

• A fictitious force results from an accelerated
  frame of reference
• A fictitious force appears to act on an object in
  the same way as a real force, but you cannot
  identify a second object for the fictitious force
• Remember that real forces are always interactions
  between two objects

6
Centrifugal Force

• From the frame of the passenger (b), a force
  appears to push her toward the door
• From the frame of the Earth, the car applies a
  leftward force on the passenger
• The outward force is often called a centrifugal
  force
• It is a fictitious force due to the centripetal
  acceleration associated with the cars change in
  direction
• In actuality, friction supplies the force to
  allow the passenger to move with the car
• If the frictional force is not large enough, the
  passenger continues on her initial path according
  to Newtons First Law

7
Coriolis Force

• This is an apparent force caused by changing the
  radial position of an object in a rotating
  coordinate system

The result of the rotation is the curved path of
object Ball in figure to the right, winds, rivers
and currents on earth. For winds we get the
prevailing wind pattern below.
8
Fictitious Forces, examples

• Although fictitious forces are not real forces,
  they can have real effects
• Examples
• Objects in the car do slide
• You feel pushed to the outside of a rotating
  platform
• The Coriolis force is responsible for the
  rotation of weather systems, including
  hurricanes, and ocean currents

9
Introduction to Energy

• The concept of energy is one of the most
  important topics in science and engineering
• Every physical process that occurs in the
  Universe involves energy and energy transfers or
  transformations
• Energy is not easily defined

10
Work

• The work, W, done on a system by an agent
  exerting a constant force on the system is the
  product of the magnitude F of the force, the
  magnitude Dr of the displacement of the point of
  application of the force, and cos q, where q is
  the angle between the force and the displacement
  vectors

11
Work, cont.

• W F Dr cos q F. Dr
• The displacement is that of the point of
  application of the force
• A force does no work on the object if the force
  does not move through a displacement
• The work done by a force on a moving object is
  zero when the force applied is perpendicular to
  the displacement of its point of application

12
Work Example

• The normal force and the gravitational force do
  no work on the object
• cos q cos 90 0
• The force is the only force that does work on
  the object

13
Units of Work

• Work is a scalar quantity
• The unit of work is a joule (J)
• 1 joule 1 newton . 1 meter
• J N m ( Fr)
• The sign of the work depends on the direction of
  the force relative to the displacement
• Work is positive when projection of onto
  is in the same direction as the displacement
• Work is negative when the projection is in the
  opposite direction

14
Work Done by a Varying Force

• Assume that during a very small displacement, Dx,
  F is constant
• For that displacement, W F Dx
• For all of the intervals,

15
Work Done by a Varying Force, cont

• Therefore,
• The work done is equal to the area under the
  curve between xi and xf

16
Work Done By A Spring

• A model of a common physical system for which the
  force varies with position
• The block is on a horizontal, frictionless
  surface
• Observe the motion of the block with various
  values of the spring constant

17
Hookes Law

• The force exerted by the spring is
• Fs - kx
• x is the position of the block with respect to
  the equilibrium position (x 0)
• k is called the spring constant or force constant
  and measures the stiffness of the spring
• This is called Hookes Law

18
Hookes Law, cont.

• When x is positive (spring is stretched), F is
  negative
• When x is 0 (at the equilibrium position), F is 0
• When x is negative (spring is compressed), F is
  positive

19
Hookes Law, final

• The force exerted by the spring is always
  directed opposite to the displacement from
  equilibrium
• The spring force is sometimes called the
  restoring force
• If the block is released it will oscillate back
  and forth between x and x

20
Hookes Law consider the spring

• When x is positive (spring is stretched), Fs is
  negative
• When x is 0 (at the equilibrium position), Fs is
  0
• When x is negative (spring is compressed), Fs is
  positive
• Hence the restoring force
• Fs Fs -kx

21
Work Done by a Spring

• Identify the block as the system and see figure
  below
• The work as the block moves from xi - xmax to
  xf 0 is ½ kx2
• Note The total work done by the spring as the
  block moves from xmax to xmax is zero see
  figure also
• Ie. From the General definition
• Or

22
Work Done by a Spring,in general

• Assume the block undergoes an arbitrary
  displacement from x xi to x xf
• The work done by the spring on the block is
• If the motion ends where it begins, W 0
• NOTE the work is a change in the expression
• 1/2kx2 We say a change in elastic potential
  energy..in general a energy expression is defined
  for various forces and the work done changes that
  energy.

23
Kinetic Energy and Work-Kinetic Energy Theorem

• Kinetic Energy is the energy of a particle due to
  its motion
• K ½ mv2
• K is the kinetic energy
• m is the mass of the particle
• v is the speed of the particle
• A change in kinetic energy is one possible result
  of doing work to transfer energy into a system

24
Kinetic Energy

• Calculating the work

IE. adv/dt adxdv/dt dx dv dx/dtvdv

• The Work-Kinetic Energy Theorem states SW Kf
  Ki DK

• Hence K1/2 mv2 is a a natural for energy
  expression..
• And the last equation is called the Work-Kinetic
  Energy Theorem
• Again we note that the work done changes an
  energy expression
• in this case a change in Kinetic energy
• The speed of the system increases if the work
  done on it is positive
• The speed of the system decreases if the net work
  is negative
• Also valid for changes in rotational speed

25
Potential Energy in general

• Potential energy is energy related to the
  configuration of a system in which the components
  of the system interact by forces
• The forces are internal to the system
• Can be associated with only specific types of
  forces acting between members of a system

26
Gravitational Potential EnergyNEAR SURFACE OF
EARTH ONLY

• The system is the Earth and the book
• Do work on the book by lifting it slowly through
  a vertical displacement
• The work done on the system must appear as an
  increase in the energy of the system

27
Gravitational Potential Energy, cont

• There is no change in kinetic energy since the
  book starts and ends at rest
• Gravitational potential energy is the energy
  associated with an object at a given location
  above the surface of the Earth

28
Gravitational Potential Energy, final

• The quantity mgy is identified as the
  gravitational potential energy, Ug
• Ug mgy
• THIS IS ONLY NEAR THE EARTHs surface
  WHY???????
• Units are joules (J)
• Is a scalar
• Work may change the gravitational potential
  energy of the system
• Wnet DUg

29
Conservative Forces and Potential Energy

• Define a potential energy function, U, such that
  the work done by a conservative force equals the
  decrease in the potential energy of the system
• The work done by such a force, F, is
• DU is negative when F and x are in the same
  direction

30
Conservative Forces and Potential Energy

• The conservative force is related to the
  potential energy function through
• The x component of a conservative force acting on
  an object within a system equals the negative of
  the potential energy of the system with respect
  to x
• Can be extended to three dimensions

31
Conservative Forces and Potential Energy Check

• Look at the case of a deformed spring
• This is Hookes Law and confirms the equation for
  U
• U is an important function because a conservative
  force can be derived from it

32
Energy Diagrams and Equilibrium

• Motion in a system can be observed in terms of a
  graph of its position and energy
• In a spring-mass system example, the block
  oscillates between the turning points, x xmax
• The block will always accelerate back toward x
  0

33
Energy Diagrams and Stable Equilibrium

• The x 0 position is one of stable equilibrium
• Configurations of stable equilibrium correspond
  to those for which U(x) is a minimum
• x xmax and x -xmax are called the turning
  points

34
Energy Diagrams and Unstable Equilibrium

• Fx 0 at x 0, so the particle is in
  equilibrium
• For any other value of x, the particle moves away
  from the equilibrium position
• This is an example of unstable equilibrium
• Configurations of unstable equilibrium correspond
  to those for which U(x) is a maximum

35
Neutral Equilibrium

• Neutral equilibrium occurs in a configuration
  when U is constant over some region
• A small displacement from a position in this
  region will produce neither restoring nor
  disrupting forces

36
Ways to Transfer Energy Into or Out of A System

• Work transfers by applying a force and causing
  a displacement of the point of application of the
  force
• Mechanical Waves allow a disturbance to
  propagate through a medium
• Heat is driven by a temperature difference
  between two regions in space

A word from our sponsors CONDUCTION,
CONVECTION, RADIATION
37
More Ways to Transfer Energy Into or Out of A
System

• Matter Transfer matter physically crosses the
  boundary of the system, carrying energy with it
• Electrical Transmission transfer is by electric
  current
• Electromagnetic Radiation energy is transferred
  by electromagnetic waves

38
Two New important Potential Energies

• In the universe at large Gravitational force as
  defined by Newton prevails
• Ie.. F -Gm1m2 /r2 m the masses G a universal
  constant and r distance between the masses
  (negative is attractive force)
• In the atomic world the electric force dominates
  defined as Fkq1q2 /r2 here r is the distance
  between the electric charges represented by q and
  k a universal constant
• Charges can be or -
• The Constant values.G,k depend upon units used

39
Gravitational and Electric Potential energies (3D)
With r replacing x we get and using the
gravitational and electric forces equations and S
for integration from point initial to final W S
FGdr - Gm1m2 S 1/r2 dr -Gm1m2 (1/rf
-1/ri) W S Fedr kq1q2 S 1/r2 dr kq1q2
(1/rf -1/ri) Or potential energies for these
forces go as 1/r Note from above that F -dU/dr
with UG Gm1m2 /r Ue kq1q2 /r we get back
the 1/r2 forces
40
Conservation of Energy

• Energy is conserved
• This means that energy cannot be created nor
  destroyed
• If the total amount of energy in a system
  changes, it can only be due to the fact that
  energy has crossed the boundary of the system by
  some method of energy transfer!

41
Isolated System

• For an isolated system, DEmech 0
• Remember Emech K U
• This is conservation of energy for an isolated
  system with no nonconservative forces acting
• If nonconservative forces are acting, some energy
  is transformed into internal energy
• Conservation of Energy becomes DEsystem 0
• Esystem is all kinetic, potential, and internal
  energies
• This is the most general statement of the
  isolated system model

42
Isolated System, cont

• (example book falling)
• The changes in energy DEsystem 0
• Or DK DU0
• DK-DU
• Ie. Kf - Ki -(Uf Ui)
• can be written out and rearranged
• Kf Uf Ki Ui Remember, this applies only
  to a system in which conservative forces act
• Or 1/2mvf2 mghf 1/2mgvi2mghi

43
Example Free Fallexample 8-1

• Determine the speed of the ball at y above the
  ground
• Conceptualize
• Use energy instead of motion
• Categorize
• System is isolated
• Only force is gravitational which is conservative

44
Example Free Fall, cont

• Analyze
• Apply Conservation of Energy
• Kf Ugf Ki Ugi
• Ki 0, the ball is dropped
• Solving for vf
• Finalize
• The equation for vf is consistent with the
  results obtained from kinematics

45
For the electric force

• Total energy
• Is KU1/2mv2 kq1q2 /r
• Specifically in a hydrogen atom using charge
  units e (CALLED ESU we get rid of K) and the
  proton and electron both have the same charge e
• Or total energy for electron in orbit
• 1/2mv2 e2 /r we will use this in chapter 3

46
Instantaneous Power

• Power is the time rate of energy transfer
• The instantaneous power is defined as
• Using work as the energy transfer method, this
  can also be written as

47
Power

• The time rate of energy transfer is called power
• The average power is given by
• when the method of energy transfer is work
• Units of power
• what is a Joule/sec called ?
• Answer WATT! 1 watt1joule/sec

48
Instantaneous Power and Average Power

• The instantaneous power is the limiting value of
  the average power as Dt approaches zero
• The power is valid for any means of energy
  transfer
• NOTE only part of F adds to power ?

49
Units of Power

• The SI unit of power is called the watt
• 1 watt 1 joule / second 1 kg . m2 / s2
• A unit of power in the US Customary system is
  horsepower
• 1 hp 746 W
• Units of power can also be used to express units
  of work or energy
• 1 kWh (1000 W)(3600 s) 3.6 x106 J

50
Example 8.10 melev 1600kg passengers 200kg A
constant retarding force 4000 N How much power
to lift at constant rate of 3m/s How much power
to lift at speed v with a1.00 m/ss
T
f
USE SF 0 in first part and ma in second then
use Next equation
W