Factoring - PowerPoint PPT Presentation
Title:
Factoring
Description:
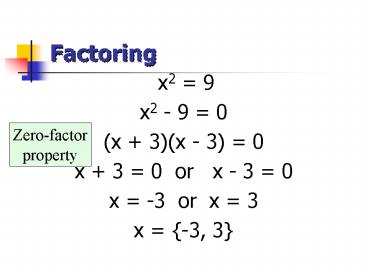
Factoring x2 = 9 x2 - 9 = 0 (x + 3)(x - 3) = 0 x + 3 = 0 or x - 3 = 0 x = -3 or x = 3 x = {-3, 3} Zero-factor property – PowerPoint PPT presentation
Number of Views:170
Avg rating:3.0/5.0
Title: Factoring
1
Factoring
- x2 9
- x2 - 9 0
- (x 3)(x - 3) 0
- x 3 0 or x - 3 0
- x -3 or x 3
- x -3, 3
Zero-factor property
2
Another Way to Solve QuadraticsSquare Root
Property
Recall that we know the solution set is x -3,
3
When you introduce the radical you must use and
- signs.
3
Solving Quadratic Equations by Completing the
Square
- Solve the following equation by completing the
square - Step 1 Move quadratic term, and linear term to
left side of the equation
4
Perfect Square Trinomials
- Create perfect square trinomials.
- x2 20x ___
- x2 - 4x ___
- x2 5x ___
100 4 25/4
5
Creating a Perfect Square Trinomial
- In the following perfect square trinomial, the
constant term is missing.
X2 14x ____
- Find the constant term by squaring half the
coefficient of the linear term. - (14/2)2
X2 14x 49
6
Solving Quadratic Equations by Completing the
Square
- Step 2 Find the term that completes the square
on the left side of the equation. Add that term
to both sides.
7
Solving Quadratic Equations by Completing the
Square
- Step 4 Take the square root of each side
8
Solving Quadratic Equations by Completing the
Square
- Step 5 Set up the two possibilities and solve
9
- AAT-A Date 2/3/14 SWBAT complete the square to
solve factoring problems - Do Now Go over Semester 1 Exam
- HW Requests pg 303 35-41 odds 42-49
- HW Pg 310 15-37 odds Read Section 6.4
- Begin Section 6.5
- Announcements
- Chapt. 5 Vocab Sheet due Tues.
- Tutoring Tues. and Thurs. 3-4
- Bring Graphing Calculator to
- Class for Thursday
- Quiz Friday w/HW Quiz before
- Complete presentations Tues.
- Bring your presentation on a
- Flash drive.
Life Is Just A MinuteLife is just a minuteonly
sixty seconds in it.Forced upon youcan't refuse
it.Didn't seek itdidn't choose it.But it's up
to you to use it.You must suffer if you lose
it.Give an account if you abuse it.Just a tiny,
little minute,But eternity is in it!By Dr.
Benjamin Elijah Mays, Past President of
Morehouse College
10
Solving Quadratic Equations by Completing the
Square
11
Section 8.1
- Completing the Square
12
Factoring
- Before today the only way we had for solving
quadratics was to factor. - x2 - 2x - 15 0
- (x 3)(x - 5) 0
- x 3 0 or x - 5 0
- x -3 or x 5
- x -3, 5
Zero-factor property
13
Square Root Property
- If x and b are complex numbers and if x 2 b,
then
OR
14
Solve each equation. Write radicals in
simplified form.
Square Root Property
15
Solve each equation. Write radicals in
simplified form.
Square Root Property
Radical will not simplify.
16
Solve each equation. Write radicals in
simplified form.
Square Root Property
Solution Set
17
Solve each equation. Write radicals in
simplified form.
18
Solve each equation. Write radicals in
simplified form.
19
Perfect Square Trinomials
- Examples
- x2 6x 9
- x2 - 10x 25
- x2 12x 36
20
Completing the Square
- 1. Divide by the coefficient of the squared term.
Make the coefficient of the squared term 1. - 2. Move all variables to one side and constants
to the other. - 3. Take half of the coefficient of the x term and
square it. Then add to both sides of the
equation. - 4. Factor the left hand side and simplify the
right. - 5. Root and solve.
21
Completing the Square
- 1.Divide by the coefficient of the squared term.
Make the coefficient of the squared term 1. - 2. Move all variables to one side and constants
to the other. - 3. Take half of the coefficient of the x term and
square it. Then add to both sides of the
equation. - 4. Factor the left hand side and simplify the
right. - 5. Root and solve.
22
Completing the Square
- 1. Make the coefficient of the squared term 1.
- 2. Move all variables to one side and constants
to the other. - 3. Take half of the coefficient of the x term and
square it. Then add to both sides of the
equation. - 4. Factor the left hand side and simplify the
right. - 5. Root and solve.
23
Completing the Square
- 1.Divide by the coefficient of the squared term.
Make the coefficient of the squared term 1. - 2. Move all variables to one side and constants
to the other. - 3. Take half of the coefficient of the x term and
square it. Then add to both sides of the
equation. - 4. Factor the left hand side and simplify the
right. - 5. Root and solve.
24
- 1. Make the coefficient of the squared term 1.
- 2. Move all variables to one side and constants
to the other. - 3. Take half of the coefficient of the x term and
square it. Then add to both sides of the
equation. - 4. Factor the left hand side and simplify the
right. - 5. Root and solve.
25
Solving Quadratic Equations by Completing the
Square
- x2 - 2x - 15 0
- (x 3)(x - 5) 0
- x 3 0
- or x - 5 0
- x -3 or x 5
- x -3, 5
Now take 1/2 of the coefficient of x. Square
it. Add the result to both sides.
Factor the left. Simplify the right.
Square Root Property
26
Solving Quadratic Equations by Completing the
Square
Try the following examples. Do your work on your
paper and then check your answers.
27
Solving Quadratic Equations by Completing the
Square
Step 3 Factor the perfect square trinomial on
the left side of the equation. Simplify the
right side of the equation.
28
Deriving The Quadratic Formula
Divide both sides by a
Complete the square by adding (b/2a)2 to both
sides
Factor (left) and find LCD (right)
Combine fractions and take the square root of
both sides
Subtract b/2a and simplify
29
Completing the Square-Example 2
- Solve the following equation by completing the
square - Step 1 Move quadratic term, and linear term to
left side of the equation, the constant to the
right side of the equation.
30
Solving Quadratic Equations by Completing the
Square
Step 2 Find the term that completes the square
on the left side of the equation. Add that term
to both sides. The quadratic coefficient must be
equal to 1 before you complete the square, so you
must divide all terms by the quadratic
coefficient first.
31
Solving Quadratic Equations by Completing the
Square
Step 3 Factor the perfect square trinomial on
the left side of the equation. Simplify the
right side of the equation.
32
Solving Quadratic Equations by Completing the
Square
Step 4 Take the square root of each side