Linear Equations - PowerPoint PPT Presentation
Title:
Linear Equations
Description:
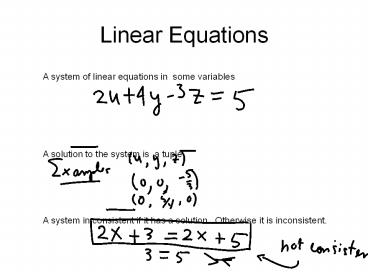
Linear Equations A system of linear equations in some variables A solution to the system is a tuple A system in consistent if it has a solution. – PowerPoint PPT presentation
Number of Views:746
Avg rating:3.0/5.0
Title: Linear Equations
1
Linear Equations
A system of linear equations in some
variables A solution to the system is a
tuple A system in consistent if it has a
solution. Otherwise it is inconsistent.
2
Methods for solving a system of linear
equations i.e. finding all solutions 1
equation in one or more variables. Find 3
solutions to 2y 4u 5v 3 Common
2 Select all solutions to 10y 9u 3x
21 0 from the given list. (24/5,-2,-3)
(6,3,4) (1,1,1) (6,-3,-4)
(0,0,0) (-6,-3,-4)
3
Very often you cannot if a system is consistent
or inconsistent without solving it. Common 3
The set of equations -2t8 -6t 9, -12t
7 -2t 2 Is consistent/ inconsistent?
Common 12 The equation (x5) (-9x -2)
(-7x 16) (-17x 9) is Consistent/incosistent?
4
Why worry about solving linear equations?
Because many questions can be answered by
setting up and solving a system of linear
equations Common 1 The product of 7 and an
unknown number is added to 6. If the reciprocal
of the resulting number is multiplied by 5, the
result is 7. The unknown number must be
______
5
General The product of a number A and an
unknown number is added to a number B. If the
reciprocal of the resulting number is multipled
by a number C, the resulting number is D. The
unknown number must be________
6
Another example By itself the cold water
faucet can fill a tub in 12 minutes while by
itself the hot water faucet can fill the tub in 6
minutes. Together the two faucets can fill the
tub in ______ minutes. Solution
7
Systems of linear equations in more than one
variable can be Inconsistent no
solution Consistent many
solutions Consistent 1 solution
8
- Systems of two linear equations in two variables
Methods of solving - Substitution Solve the first equation for one
of the variables in terms of the - other. Substitute that value into the second
equation and solve the resulting equation - in 1 variable for that variable. Substitute that
value back into the first equation to - get the value of the first variable.
9
2 Elimination Add a multiple of the first
equation to the second equation so that one of
the variables is eliminated. Solve the resulting
equation for the remaining variable. Substitute
that value back into the first equation and solve
for the first variable.
10
Cramers rule is what you get when you solve
the general system of two linear equations in two
variables by elimination. It is convenient to
use if the system has a unique solution.
11
Why worry about all this? Because lots of
questions can be answered by setting up and
solving a system of two equations in two
variables. Common 10 A grocery shelf has cans
of red beans and black beans If 4 cans of
red And 9 cans of black beans add to 30 pounds,
and 6 cans of red beans and 8 cans of black beans
add to 34 pounds, how much does each can of
each color bean weigh? Red _____ Black
_______
12
Assignment Make a variation on the bean problem
and solve it.