Multiply polynomials. - PowerPoint PPT Presentation
Title:
Multiply polynomials.
Description:
Title: Slide 1 Author: HRW Last modified by: j.bolla Created Date: 10/14/2002 6:20:28 PM Document presentation format: On-screen Show (4:3) Company – PowerPoint PPT presentation
Number of Views:108
Avg rating:3.0/5.0
Title: Multiply polynomials.
1
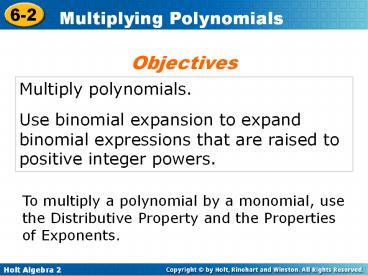
Objectives
Multiply polynomials. Use binomial expansion to
expand binomial expressions that are raised to
positive integer powers.
To multiply a polynomial by a monomial, use the
Distributive Property and the Properties of
Exponents.
2
Example 1 Multiplying a Monomial and a Polynomial
Find each product.
A. 4y2(y2 3)
4y2(y2 3)
4y2 ? y2 4y2 ? 3
4y4 12y2
B. fg(f4 2f3g 3f2g2 fg3)
fg(f4 2f3g 3f2g2 fg3)
fg ? f4 fg ? 2f3g fg ? 3f2g2 fg ? fg3
f5g 2f4g2 3f3g3 f2g4
3
Check It Out! Example 1
Find each product.
a. 3cd2(4c2d 6cd 14cd2)
b. x2y(6y3 y2 28y 30)
4
To multiply any two polynomials, use the
Distributive Property and multiply each term in
the second polynomial by each term in the first.
Keep in mind that if one polynomial has m terms
and the other has n terms, then the product has
mn terms before it is simplified.
5
Example 2A Multiplying Polynomials
Find the product.
(a 3)(2 5a a2)
Method 1 Multiply horizontally.
(a 3)(a2 5a 2)
a(a2) a(5a) a(2) 3(a2) 3(5a) 3(2)
a3 5a2 2a 3a2 15a 6
a3 8a2 17a 6
6
Example 2A Multiplying Polynomials
Find the product.
(a 3)(2 5a a2)
Method 2 Multiply vertically.
3a2 15a 6
a3 5a2 2a
a3 8a2 17a 6
7
Check It Out! Example 2a
Find the product.
Multiply horizontally.
(3b 2c)(3b2 bc 2c2)
8
Check It Out! Example 2b
Find the product.
Multiply vertically
(x2 4x 1)(x2 5x 2)
9
Find the product.
(a 2b)3
10
Find the product.
(x 4)4
11
Find the product.
(2x 1)3
12
Lesson Quiz
Find each product.
1. 5jk(k 2j)
5jk2 10j2k
2. (2a3 a 3)(a2 3a 5)
2a5 6a4 11a3 14a 15
3. (3a b)3
27a3 27a2b 9ab2 b3