Warm Up - PowerPoint PPT Presentation
1 / 23
Title: Warm Up
1
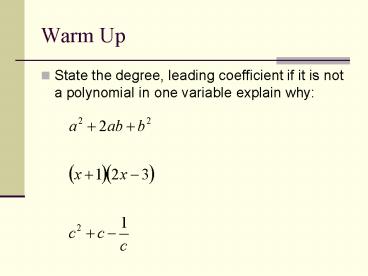
Warm Up
- State the degree, leading coefficient if it is
not a polynomial in one variable explain why
2
HW
3
7-2. Graphing Polynomial Functions
- Objective
- Graph polynomial functions locate their real
zeros - Find the maxima minima of polynomial functions
4
Graph Polynomial Functions
- To graph a polynomial functions, make a table of
values to find several points and then connect
them to make a smooth curve. - Knowing the end behavior of the graph will assist
you in completing the sketch of the graph.
5
So Lets Graph!
x f(x) x f(x)
-2.5 0.0
-2.0 0.5
-1.5 1.0
-1.0 1.5
-0.5 2.0
- by making a table
of values - Even-degree polynomial with a positive leading
coefficient, so - How many zeros?
6
Completed Table
x f(x) x f(x)
-2.5 8.4 0.0 0
-2.0 0.0 0.5 -2.8
-1.5 -1.3 1.0 -6.0
-1.0 0.0 1.5 -6.6
-0.5 0.9 2.0 0.0
7
(No Transcript)
8
Location Principle
- In our first example, notice that the values of
the function before and after each zero are
different in sign. - In general, the solutions of a polynomial occur
somewhere between pairs of x values _at_ which the
corresponding y values change signs. - This property for locating zeroes is called the
Location Principle
9
Key Concept!
- Suppose yf(x) represents a polynomial function
and a and b are two numbers such that f(a)
lt 0 and f(b) gt 0. Then the function has at least
one real zero between a and b.
10
Key Concept
- If you have a polynomial f(x) and you have two
points a and b and f(a) is positive and
f(b) is negative, there is a solution in
between a and b
11
Locate Zeros of a Function
- Determine consecutive values of x between which
each real zero of the function f(x)x³-5x²3x2
is located. - Then draw the graph.
- Note Since f(x) is a third-degree polynomial
function, it will have either ___, ___, or ___
zeros.
12
f(x)x³-5x²3x2
X f(x)
-2
-1
0
1
2
3
4
5
13
f(x)x³-5x²3x2
X f(x)
-2 -32
-1 -7
0 2
1 1
2 -4
3 -7
4 -2
5 17
- gt CHANGE IN SIGNS
- gt CHANGE IN SIGNS
- gt CHANGE IN SIGNS
14
From the previous example
- NOTE The changes in sign indicate that there
are zeros between x-1 and x0, between x1 and
x2, and between x4 and x5.
15
Maximum Minimum
16
HW Page 356 (13-18) a b ONLY Sketch graph
MAKE A TABLE!!!!
- 13-16
- 17-18
17
Relative Maximum Minimum
- Point A on graph is relative maximum of the cubic
function since no other nearby points have a
greater y-coordinate. - Likewise, point B is a relative minimum since no
other nearby points have a lesser y-coordinate. - These points are often referred to as turning
points
18
Key Points
- The graph of a polynomial function of degree n
has at most n-1 turning points. - The plurals of maximum minimum are maxima
minima
19
One more example!
X f(x)
-2
-1
0
1
2
3
- Graph f(x)x³-3x²5. Estimate the
x-coordinates at which the relative maxima
relative minima occur.
20
Answer
- The values of f(x) change signs between x-2 and
x-1, indicating a zero of the function. - The value of f(x) at x0 is greater than the
surrounding points, so it is a relative maximum. - The value of f(x) at x2 is less than the
surrounding points, so it is a relative minimum.
21
HW
- Page 356 (13-18) You will need to sketch the
graph of polynomial functions and may want to use
graph paper. - If you Google printable graph paper several
sites will come up that allow you to print graph
paper out.
22
CALCULATOR!!!
- You can use your graphing calculator to find the
coordinates of relative maxima and relative
minima. Enter the polynomial function in the Y
list and graph the function. Make sure that all
the turning points are visible in the viewing
window. Find the coordinates of the minimum and
maximum points respectively
23
Graphing Calculator (Maxima Minima)
- Step 1 Graph the function so that the vertex of
the parabola is visible. - Step 2 Select 3minimum or 4maximum from the
CALC menu. - Step 3 Using the arrow keys, locate a left bound
and press ENTER. - Step 4 Locate a right bound and press ENTER
twice. The cursor appears on the maximum or
minimum point of the function. The maximum or
minimum value is the y-coordinate of that point.