Momentum Integral Equation

1
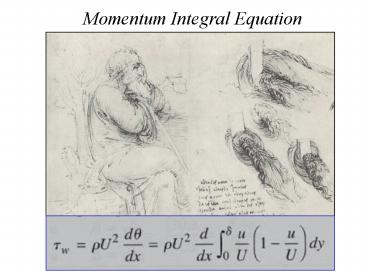
Momentum Integral Equation
2
Von Karman and Poulhausen derived momentum
integral equation (approximation) which can be
used for both laminar and turbulent flow (with
and without pressure gradient)
3
Von Karman and Polhausen devised a simplified
method by satisfying only the boundary conditions
of the boundary layer flow rather than
satisfying Prandtls differential equations for
each and every particle within the boundary layer.
4
(No Transcript)
5
Want to solve for ? in Laminar Flow

• assume velocity profile,
• u/U f(y/??), similar profiles
• u ? Ue at y ?
• ?u/?y ? 0 at y ?
• u 0 at y 0
• ?w ??u/?y ?U/?d(u/U)/d?

6
LAMINAR FLOW
7
(plate is 2 thick, RexL 10,000 air bubbles
in water)
For flat plate with dp/dx 0, dU/dx 0
8

• Realize (like Blasius) that u/U
• similar for all x when plotted
• as a function of y/?.
• Substitutions ? y/? so dy ?d?
• 0 when y0 ?1 when y ?

Not f(x)
9
Strategy assume velocity profile u/Uo f(?),
obtain an expression for ?w as a function of ?,
and solve for ?
f (?)
10
Laminar Flow Over a Flat Plate, dp/dx 0
Want to know ?w(x)
Assume velocity profile u a by cy2 B.C. at
y 0 u 0 so a 0 at y
? u U so U b? c?2 at y ?
?u/?y 0 b 2c? so b -2c? U
-2c?2 c?2 -c?2 so c -U/?2 b 2U/?
u a by cy2 0 2Uy/? Uy2/?2
u/U 2? -?2
11
Laminar Flow Over a Flat Plate, dp/dx 0
Want to know ?w(x)
Assume velocity profile u a by cy2 u a
by cy2 0 2Uy/? Uy2/?2 u/U 2(y/?)
(y/?)2 Let y/? ? u/U 2? -?2
12
Laminar Flow Over a Flat Plate, dp/dx 0
u/U 2? -?2
Strategy obtain an expression for ?w as a
function of ?, and solve for ?(x)
13
?w 2?U/? u/U 2? -?2
2? - 4?2 2?3 - ?2 2?3 - ?4
Strategy obtain an expression for ?w as a
function of ?, and solve for ?(x)
14
2?U/(??U2) (d?/dx) (?2 (5/3)?3 ?4
(1/5)?5)01 2?U/(??U2) (d?/dx) (1 5/3 1
1/5) (d?/dx) (2/15) 15?dx ??U(d?)
Assuming ? 0 at x 0, then c 0
?2/2 15?x/(?U)
Strategy obtain an expression for ?w as a
function of ?, and solve for ?(x)
15
?2/2 15?x/(?U) ?2/x2 30?/(?Ux) 30
Rex ?/x 5.48 (Rex)-1/2 Exact Solution ?/x
5(Rex)-1/2
Can also calculate drag on plate by integrating
over ?w since know ?w 2?U/? Since know ? and
u(x,y) can also calculate ?.
16
TURBULENT FLOW
17
BREATH
18
Want to solve for ? in Laminar Flow

• u/Uo (y/?)1/n (from pipe)
• u/Uo ?1/n similar profiles
• 2. ?w ??u/?y BLOWS UP at y 0
• ?w 0.0332? (V)2?/(RV)1/4

19
Calculating drag on a flat plate, zero pressure
gradient turbulent flow u/Uo (y/?)1/8

Cant use ?wall ?du/dy? y0
?u/?y blows up at y 0
20
Calculating drag on a flat plate, zero pressure
gradient turbulent flow
u/Uo (y/?)1/8 Uo Uc/l R ?
21
?w 0.0332 ? V2 ?/(RV)1/4
PIPE
TO USE FOR FLAT PLATE need to Uavg to Uc/l
and R to ?
22
?w 0.0332 ? V2 ?/(RV)1/4 V 0.837 Uc/l R
? ?w 0.0243 ? Uc/l2 ?/(Uc/l ?)1/4
u/Uo (y/?)1/8 ?1/8
23
u/Uo (y/?)1/8 ?1/8
24
?w 0.0243 ? Uo2 ?/(Uo ?)1/4 ?w (8/90) ?
Uo2d?/dx ?1/4d? 0.274(?/U)1/4dx (4/5)?5/4
0.274 (?/U)1/4x c
25
Turbulent Flow
Assume tripped at leading edge so turbulent flow
everywhere on plate
(4/5)?5/4 0.274 (?/U)1/4x c
Assume ? 0 at x 0, so c 0

• (5/4) 0.274 (?/U)1/4x4/5
• 0.424(?/U)1/5x4/5

? 0.424 Rex-1/5x
26
? 0.424 Rex-1/5x
?w 0.0243 ? U2 (?/(U?))1/4 ?w 0.0243 ? U2
(?/(U 0.424 Rex-1/5x ))1/4
?w 0.0301 ? U2 Rex-1/5
27
(No Transcript)
28
Re increases, n increases, wall shear stress
increases, boundary layer increases, viscous
sublayer decrease
u/U
u/U (y / ?)1/6 u/U (y / ?)1/7 u/U (y / ?)1/8
y/?
29
LAMINAR BOUNDARY LAYER AT SEPARATION
Given u/U a b? c?2 d ?3 What are
boundary conditions?
30
Given u/U a b? c?2 d ?3
? y/?
Separating ?u/?y 0

• 0 u 0 a 0
• 0 ?u/?y 0 b 0
• ? u U 1 c d
• ? ?u/?y 0 2c 3d 0
• 2(1-d) 3d 2 d 0
• so d -2 and c 3

u/U 3 ?2 -2 ?3
31
Separating Flow u/U 3 ?2 -2 ?3
dp/dx gt 0
dp/dx 0
32
QUESTIONS
33
Which way is flow moving?
34

• ? In laminar flow along a plate, ?(x), ?(x),
  ?(x) and?w(x)
• Continually decreases
• Continually increases
• Stays the same
• ? In turbulent flow along a plate, ?(x), ?(x),
  ?(x) and?w(x)
• Continually decreases
• Continually increases
• Stays the same
• ?At transition from laminar to turbulent flow,
  ?(x), ?(x), ?(x)
• and?w(x)
• Abruptly decreases
• Abruptly increases
• Stays the same

35
61 ellipsoid
?wall
Turbulent Laminar
forced
natural
Turbulent Laminar
?
36
What is wrong with this figure?
37
What is wrong with this figure?
38
80 of fresh water found in world Antarctic ice
Are Antarctic Icebergs Towable Arctic News Record
Summer 1984 36
Cf 0.074/Re1/5 (FoxCf 0.0594/Re1/5) Area
1 km long x 0.5 km wide ?sea water 1030 kg/m3
?sea water 1.5 x 10-6 m2/sec Power available
10 kW Maximum speed ?
39
P UD 104 U Cf½ ? U2A U 0.074
?1/5/(U1/5L1/5)( ½ ? U2A) 104 U14/5
0.074(1.5x10-6)1/5/10001/5 x ½
(1030)(1000)(500) U14/5 0.03054 U 0.288
m/s Rex 3x108