Vibrations of Continuous Systems

1
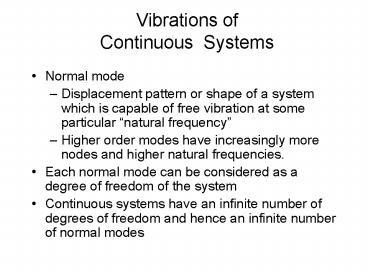
Vibrations of Continuous Systems

• Normal mode
• Displacement pattern or shape of a system which
  is capable of free vibration at some particular
  natural frequency
• Higher order modes have increasingly more nodes
  and higher natural frequencies.
• Each normal mode can be considered as a degree of
  freedom of the system
• Continuous systems have an infinite number of
  degrees of freedom and hence an infinite number
  of normal modes

2
Cool!
Guitar String
y (x,t)
y
x
L

• Length L
• Mass / Length
• Tension T

• Equilibrium position is x axis,
• displacement is in y direction
• y is a continuous function of x and t

3
y (x,t)
Fy T?R -??T?L
?R ?(x dx,t) ?L ?(x,t)
Fy T ?(xdx,t)???(x,t)?
4
T
dx
?(xdx)
?(x)
T
xdx
For small displacements
x
y force on element
5
y-force on element
Fy
m
Mass of element
dx
Newtons Law
xdx
x
Equation of Motion
6
Wave Equation
Equation of motion
Define the wave speed
Wave Equation
7
One Solution
wave going to the right
wave going to the left
where f and g are completely arbitrary functions
Most general solution
Not the kind of solution were looking for
8
Normal Modes
y (x,t)
y
x
L
wave equation
(1)
look for solutions in the form
(2)
substitute (2) in (1)
9
Normal Modes
y
x
L
mode shape satisfies Helmholtz equation
solution
B 0
boundary conditions
10
Normal Modes
Natural frequencies
Normal modes
Note Natural frequencies are harmonics of f1
11
Normal Modes
1
T
n 1
f
?
1
2L
?

L
1
T
f
n 2
?
2
L
?
L
n 3
3
T
f
?
3
2L
?
L
2
T
f
?
n 4
4
L
?
L
12
Initial Condition (Plucked String)
x
13
Initial Condition (Plucked String)
x
14
Subsequent motion?
x
15
Subsequent motion?
x
16
Subsequent motion?
x
17
Subsequent motion?
x
18
Subsequent motion?
x
19
Subsequent motion?
x
20
Subsequent motion?
x
21
Subsequent motion?
x
22
Subsequent motion?
x
23
Subsequent motion?
x
24
Subsequent motion?
x
25
Normal Mode Motion
y
x
26
Normal Mode Motion
y
x
27
Normal Mode Motion
y
x
28
Normal Mode Motion
y
x
29
Normal Mode Motion
y
x
30
Normal Mode Motion
y
x
31
Normal Mode Motion
y
x
32
Normal Mode Motion
y
x
33
Normal Mode Motion
y
x
34
Normal Mode Motion
y
x
35
Normal Mode Motion
y
x
36
Normal Mode Motion
y
x
37
Normal Mode Motion
y
x
38
Actual Motion
x
39
Actual Motion
x
40
Actual Motion
x
41
Actual Motion
x
42
Actual Motion
x
43
Actual Motion
x
44
Actual Motion
x
45
Actual Motion
x
46
Actual Motion
x
47
Actual Motion
A
x
L
48
Actual Motion
A
x
49
Actual Motion
A
x
50
Actual Motion
A
x
51
Actual Motion
A
x
52
Normal Modes
1
T
n 1
f
?
1
2L
?

L
1
T
f
n 2
?
2
L
?
L
n 3
3
T
f
?
3
2L
?
L
2
T
f
?
n 4
4
L
?
L
53
Fundamental Frequency of a Guitar String
Pitch Frequency

• Depends on
• length
• density
• tension

54

• Tune guitar by adjusting tension T
• Play guitar by changing length L
• Use higher density strings for low notes

55
Free-Free Beam
y
w(x,t)
x
b
L
Euler equation of motion for thin beam
Look for modal solution
56
Free-Free Beam
y
w(x,t)
x
b
L
Equation for wn(x)
General solution
57
Boundary Conditions

• Four boundary conditions
• Zero shear and zero moment at x 0 and x L

Moment
Shear
Boundary Condition Free ends
58
Other Possible Boundary Conditions
Clamped
Zero deflection and zero slope at x 0 and x L
Pinned
Zero deflection and zero moment at x 0 and x L
Shear
59
Boundary Conditions

• Four boundary conditions
• Zero shear and zero moment at x 0 and x L

Moment
Shear
Boundary Condition Free ends
60
y
w(x,t)
x
b
L
Characteristic equation
Characteristic equation
wn
Normal modes
61
Free-Free Natural Frequencies
Characteristic Equation cosx1/cosh(x)
62
y
w(x,t)
x
b
L
Characteristic equation
Characteristic equation
wn
Normal modes
63
Modes 1, 2 and 3
Normalized Displacement
x/L
64
Example
For first mode
hence
65
What about the Glockenspiel?
Are the overtones harmonic?
No, but overtones are not strongly excited and
decay quickly
66
(No Transcript)
67
Forced Drive
Sinusoidal force applied at xa

F
1
å
-
W

f
t
x
w
a
w
D
t
x
w
)
cos(
)
(
)
(
)
,
(
n
n
n
n
2
w
m

n
1
n
ö
æ
x
W
r
2
1

ç

F


n
arctan
r
D
n
,
,

ç
n
n
n
2
-
w
1
r
2
ø

-
x
2
2
è
r
r
)
2
(
)
1
(
n
n
n
n
n
-
W

f
x
t
x
w
t
x
w
))
(
cos(
)
(
)
,
(
A
A
x
w
)
,
(
W
Force -Displacement Transfer Function

W
A
T
)
(
F
68
Reciprocity
Reciprocity
xb
xb
xa
xa

Accelerometer
Accelerometer
Shaker
Shaker
69
Force-Displacement Transfer Function (Method 1 -
Random Excitation)
70
Force-Displacement Transfer Function (Method 2 -
Sinusoidal Excitation)
71
Force-Displacement Transfer Function
X/F Transfer Function (dB)
Frequency (Hz)