ENGINEERING MATHEMATICS II - PowerPoint PPT Presentation
1 / 97
Title:
ENGINEERING MATHEMATICS II
Description:
Cauchy's Mean value theorem, Taylor's theorem, Taylor's and Maclaurin series ... Verify Cauchy's Mean value Theorem for the following function ... – PowerPoint PPT presentation
Number of Views:1417
Avg rating:3.0/5.0
Title: ENGINEERING MATHEMATICS II
1
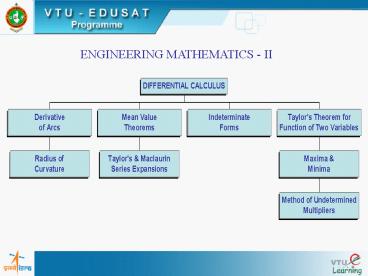
ENGINEERING MATHEMATICS - II
2
Differential Calculus
Introduction
We have already studied the Cartesian and polar
curves. In this chapter we will learn about
derivative of arcs and radius of curvature in
Cartesian, parametric and polar forms. In this
chapter we shall discuss, Rolle's theorem,
Lagrange's Mean value theorem. Cauchy's Mean
value theorem, Taylor's theorem, Taylor's and
Maclaurin series expansions of functions both in
single and two variables. Also we shall discuss
the application of differentiation to
indeterminate forms using L'Hospital rule, and
application of differential calculus to the
determination of a function which are greatest or
least in their neighbour hoods.
3
Derivatives of Arc
Derivative of the length of the arc for the
Cartesian curve y f(x). Let y f(x) be the
equation of the curve. A be a fixed point on the
curve. Let P(x, y) and
be two neighbouring points on the
curve such that arc AP s, arc PQ ds and
chord PQ dc. As on the curve
4
We have
5
- If the equation of the curve is x f(y) then
- If the equation of the curve is in parametric
form x x(t) and y y(t) - where t is the parameter then
6
Additional Results
We know that
again
7
Derivative of Arc Length in Polar Form
Let
be two neighbouring points on the curve
Let arc and chord
. As Q approaches P on the curve
8
From DONP since dq is very small,
?
?
Since dq is small
9
We have NQ OQ - ON
PQ2 PN2 NQ2
10
11
- we have
12
?
?
13
Radius of Curvature
14
Formula for radius curvature in Cartesian form
We have
Differentiate with respect to x.
15
Formula for radius of curvature in parametric
form
Let x x(t) and y y(t)
16
We have
17
Radius of curvature in pedal form
By definition
18
We have p r sin f
Differentiating with respect to r,
Comparing (1) and (2)
19
Radius of curvature in polar form
20
differentiating with respect to q
Dividing by -2r1
21
(No Transcript)
22
Problem 01
In the ellipse , show
that the radius of curvature at an end of the
major axis is equal to the semi latus rectum.
Solution
,
One end of major axis is (a, 0) in
Differentiating w.r.t x, we get, We have
23
24
Problem 02
Find the radius of curvature to the curve x a
(t - sin t), y a (1 - cos t) at any point t.
Solution
Let x a (t - sin t), y a (1 - cos t)
We have
25
(No Transcript)
26
Problem 03
Find the radius of curvature for the curve whose
pedal equation is given by pa2 r3.
Solution
Let pa2 r3 .....(1) Differentiate with
respect to p (1)
We have
27
Problem 04
Find the radius of curvature for the curve r
aeq cot a
Solution
Let r aeqcot a
We have
28
We get, p r sin a as the p-r equation
Differentiate with respect to p
29
Problem 05
With usual notations, prove that
Solution
30
From (1) and (2)
Differentiate with respect to y
31
Rolle's theorem
Statement If a function f(x) is (i) Continuous
in a, b (ii) Differentiable in (a, b)
and (iii) f(a) f(b)
32
Geometrical Interpretation
If the graph of f(x) be drawn between x a and x
b having a unique tangent at all points in
the above interval and f(a) f(b), then there
exits at least one point C on the curve
(corresponding to x c between x a and x b),
such that the tangent at C is parallel to x-axis.
33
Note 1 There may exists more than one at which
f'(x) vanishes.
34
Note 2 The three conditions of Rolle's theorem
are the sufficient conditions (but not
necessary) for f'(x) 0 for some
Note 3 Conclusion of Rolle's theorem does not
hold good for a function which does not satisfy
any of its conditions. Example consider the
function f(x) x in -1, 1
Observe that i) f(x) is continuous in -1,
1 ii) f(-1) 1 f(1)
35
But f(x) is not differentiable in (-1, 1) because
Since all the three conditions of Rolle's theorem
are not satisfied. Hence the conclusion is not
valid in -1, 1.
36
Problem 06
Verify Rolles' Theorem for the function f (x) x
(x 3) e-x/2 in -3, 0.
Solution
(i) f (x) is a product of 2 continuous functions.
\f (x) is a continuous function in -3, 0
is defined for all x in (-3, 0)
37
(iii) f (-3) 0 f (0) 0 i.e., f
(-3) f (0) All conditions of Rolles' Theorem
are satisfied. Solve f '(c) 0
38
Since
\ Rolles' Theorem is verified.
39
Lagrange's Mean value theorem (LMVT)
Let f(x) be a function such that i) Continuous
in a, b ii) Differentiable in (a, b)
40
Proof Construct a function F(x) such
that F(x) f(x) - Ax, where A is a constant
such that F(a) F(b) i.e,. f(a) - Aa f(b)
- Ab
i) Now F(x) is continuous in a,b
f(x), x is continuous ii) Since f(x), x is
derivable, F(x) is also derivable in (a,
b) (iii) Also F(a) F(b)
41
Hence F (x) satisfies all the conditions of the
Rolle's theorem.
Which proves the Lagrange's Mean Value Theorem.
42
Another form of LMVT
Let b - a h We have a lt c lt b
Note Rolle's theorem is a special case of LMVT.
43
Geometrical Interpretation of LMVT
Let the graph of f(x) be continuous between A(a,
f(a)) and B(b, f(b)). Let the curve have
tangents at all points between A and B then there
exists C(c, f(c)) on the curve between A and B
such that the tangent at C is parallel to the
chord AB.
44
Note c is not unique
45
Cor. then
f (x) is strictly increasing Let f(x) satisfy
the conditions of LMVT in a, b. Let x1, x2 be
any two points of a, b such that
x1ltx2 Applying LMVT to x1, x2
Since f(c)gt0
\ f(x) is strictly increasing.
46
Problem 07
Verify Lagranges' Mean Value Theorem for the
function
Solution
(i) f (x) is a polynomial. \ It is continuous in
(ii) f '(x) (x - 1) (x - 2) x (x - 1) x (x
- 2) is defined for \ f (x) is differentiable
in (0, ½)
47
or
\ LMVT is verified.
48
Problem 08
Verify Lagranges' Mean Value Theorem for the
function f (x) log x in 1, e.
Solution
(i) f (x) is continuous in 1, e (as it is
defined for all x in 1, e)
\ f (x) is differentiable in (1, e),
49
\ LMVT is verified
50
Problem 09
If x gt 0, prove that
Solution
(I) Let f (x) log (1 x) Applying
LMVT in 0, x f (x) f (0) x f '(qx)
51
We have, 0 lt q lt 1
52
(No Transcript)
53
Problem 10
If x gt 0, prove that
Solution
\ f (x) is an increasing function
54
(No Transcript)
55
\ F (x) is an increasing function
From (1) and (2), we get
56
Cauchy's Mean Value theorem
Statement If f(x) and g(x) are any two
functions such that (i) f(x) and g(x) are both
continuous in a, b (ii) f(x) and g(x) are both
differentiable in (a,b) and
57
Proof Consider F(x) f(x) - Ag(x) where A is a
constant to be determined such that F(a)
F(b) (i) Since f(x) and g(x) is continuous in
a, b F(x) is also continuous (ii) Since f(x)
and g(x) are derivable in (a, b) F(x) is
also derivable in (a, b) (iii) F(a) F(b)
58
F(x) satisfies all the conditions of Rolle's
theorem
59
Physical Interpretation of CMVT
CMVT interprets that the ratio of actual rates of
increase of f(x) and g(x) at x c is equal to
the ratio of their average rate of increase of
the functions in the interval (a, b).
60
Problem 11
Verify Cauchy's Mean value Theorem for the
following function
Solution
- f (x) and g (x) are both continuous in a, b
- (ii) f '(x) ex g '(x) -e-x are defined
- \ f (x) and g (x) are both differentiable in (a,
b)
61
?
e2c ea b
or
? 2c a b
\ CMVT is verified
62
Problem 12
Verify Cauchy's Mean value Theorem for the
following function
Solution
(i) f (x) and g (x) are both continuous in 1, e
\ f (x) and g (x) are differentiable in (1, e)
63
All conditions of CMVT are satisfied
we get
\ CMVT is verified
64
Taylor's Theorem (Statement Only)
Let f(x) be a function such that (i) f, f', f'',
,f(n-1) are continuous in a, b (ii) f(n-1)
is differentiable in (a, b) then
65
Another from of Taylor's
Put a h x then (2) becomes
66
67
Note 1
If f has continuous derivatives
is called the taylor series expansion of f(x)
about x a.
68
Note 2 Suppose x 0
is called the maclaurin series expansion of
f(x). Note 3 If n 1, Taylor's theorem
reduces to LMVT.
69
Problem 13
Hence show that
Solution
f (x) log x has continuous derivatives in 0,
2 We will Expand f (x) about the point x
1 We have f(x) log x f(1) 0
70
Using the above in the Taylors series
71
Put x 1.1
log (1.1) ? 0.0953
72
Problem 14
Expand sin x in powers of upto the
term containing
Solution
73
(No Transcript)
74
Problem 15
Obtain the Maclaurin series of expansion for the
function log (1 tan x) upto term containing
x3.
Solution
Let y log (1 tan x) y (0) 0
75
Differentiating (1), we get
76
Problem 16
Obtain the Maclaurin series of expansion for the
function sin-1 x.
Solution
Differentiate w.r.t x (1)
77
Differentiate w.r.t x (2)
Applying Leibnizs' Rule
Put x 0
78
(No Transcript)
79
(No Transcript)
80
Problem 17
Obtain the Maclaurin series of expansion for the
function log (1 sin x).
Solution
Let y log (1 sin x)
81
(No Transcript)
82
(No Transcript)
83
Problem 18
Obtain the Maclaurin series of expansion for the
function log (sec x).
Solution
y (0) 0
84
(No Transcript)
85
Problem 19
Obtain the Maclaurin series of expansion for the
function log (1 ex).
Solution
86
(No Transcript)
87
Indeterminate Forms
Forms like
are called in determinate forms
88
Indeterminate form (L'Hospital's Rule)
Let f(x) and g(x) be functions such that f(a) 0
and g(a) 0 or
Note If f(a), f"(a),..f(n-1)(a) and g'(a),
g"(a),,g(n-1)(a) are all zero but
89
Working Rule
1. Differentiate Numerator and Denominator
separately and put x a. If this reduces
to indeterminate form continue the above
procedure until a finite value is
obtained. 2. Incase where the expansions of
functions, involved in indeterminate form
are known, or some of the standard limits are
known, may be used to simplify the work.
90
Problem 20
Solution
Obtain the Maclaurin series of expansion for the
function log (1 ex).
91
Problem 21
Solution
92
Problem 22
Solution
93
Problem 23
Find the value of 'a' so that
Solution
94
Problem 24
Solution
which gives -3a b 0 ..(2). Solving (1)
and (2), we get a 1 and b 3.
95
Indeterminate form
This form can be converted to form and the
L'Hospital Rule applied.
Note L'Hospital Rule can be applied to the form
without adjusting to the form
96
Indeterminate form
In this case we can write f (x) . g (x) as
and the form reduces to where L'Hospital
Rule is applicable.
97
Indeterminate form
where L'Hospital Rule is applicable.
98
Problem 25
Solution
99
Indeterminate form 0o
Note Similar technique of taking logarithms is
followed in case of the indeterminate forms
100
Problem 26
Solution
log y 0
101
Problem 27
Solution
102
Problem 28
Solution
log a log e
log y log ae y ae
103
Problem 29
Solution
104
log y 0 y 1
105
Problem 30
Solution
106
Problem 31
Solution
log a log e
log y log ae y ae
107
Problem 32
Solution
log y 0 y 1
108
Taylor's Theorem for a Function of Two Variables
Let f(x, y) be a function of two variables
defined over a region R in the x - y plane. Let
P(a, b) and Q(ah, bk) be two neighbouring
points in the region R then
109
110
Cor Put a h x and b k y then
is called the taylor's series expansion of f (x,
y) about (a, b), (as, f (x, y)
possessing continuous partial derivatives of all
order).
111
Maclaurin's Expansion
For a 0 and b 0 we get from (2)
is called the Maclaurin's series expansion of
f(x, y).
112
Problem 33
Expand sin x cos y in powers of x and y as far as
terms of third degree.
Solution
We have f sinx cos y f (0, 0)
0 fx cosx cosy fx (0, 0 )
1 fy -sinx siny fy (0, 0)
0
113
(No Transcript)
114
Problem 34
Solution
115
(No Transcript)
116
Maxima and Minima
Definition A function f(x, y) of two independent
variables is said to have a minimum at (a, b),
if f(a, b) gt f(ah, bk) in the neighbourhood of
(a, b). Note 1 A maximum or minimum value of
a function is called its extreme value. Note
2 A function f(x, y) is said to be stationary
at (a, b) if fx(a, b) 0 and fy(a, b) 0.
117
118
Note 3 A sufficient condition for f(x, y) to
have a maximum at a critical point (a, b) is that
r lt 0 and rt - s2 gt 0 at (a, b). Note 4 A
sufficient condition for f(x, y) to have a
minimum at a critical point (a, b) is that r gt 0
and rt - s2 gt 0 at (a, b). Note 5 If rt - s2
lt 0 at (a, b) then (a, b) is called a saddle
point. Note 6 If rt - s2 0 and/or r 0
at (a, b) than no conclusion can be drawn about
the nature of (a, b).
119
Problem 35
Show that the function f (x, y) x3 y3 - 3xy
1 is minimum at (1, 1).
Solution
120
We have r 6 gt 0 \ f (x, y) is minimum at
(1, 1).
121
Problem 36
Show that the function f (x, y) x y (a - x -
y), a gt 0 is max. at the point
Solution
122
Now
123
Problem 37
Find the extreme values of f(x,y) sinx siny
sin()x y,
Solution
Let f(x, y) sinx siny sin (x y)
siny sin (2xy)
sinx sin (x 2y)
124
(No Transcript)
125
(No Transcript)
126
Problem 38
Examine the function f (x, y) 1 sin (x2 y2)
for extreme values.
Solution
127
(No Transcript)
128
Case (i) At (0, 0) p 0 r gt 0
s 0 t 2 Case (ii) At (a, b)
129
p 0
130
Since rt - s2 0, no conclusion can be made.
Further investigation is required. But
1 1 2 \ Maximum value of f(x,y)
is 2.
131
Problem 39
Find the extreme value of the function f(x, y)
x3 3xy2 - 3x2 - 3y2 4.
Solution
We get fx 3x2 3y2 - 6x fy 6xy -
6y fxx 6x - 6 fxy 6y s fyy 6x -
6 Solving fx 0 and fy 0
132
- i.e., 3x2 3y2 - 6x 0 (1) and 6xy - 6y 0
.(2) - from (2) we get ? x 1 or y 0
- y 0 in (1)
- ? 3x2 - 6x 0 ? 3x (x - 2) 0
- ? x 0 or x 2
- The critical points (0, 0) (2, 0)
- Put x 1 in (1)
- ? 3y2 - 3 0
- ? y ? 1
133
The other critical points are (1, 1), (1,
-1) To examine the nature of the critical points
observe the table.
\ Max. f (x, y) f (0, 0) 4 and Min. f
(x, y) f (2, 0) 0
134
Lagrange's Method of undetermined Multipliers
This method is used to find the stationary values
of a function of several variables subject to a
given condition. Note The disadvantage of
this method is we cannot determine the nature of
the stationary values.
135
Let f(x, y, z) be a function of the variables x,
y ,z subject to the condition. f(x,y, z)
c (1) Let F f lf (2) where l is a
constant
136
Let f(x, y, z) be a function of the variables x,
y ,z subject to the condition. f(x,y, z)
c (1) Let F f lf (2) where l is a
constant We form the auxillary equations Fx
0 (3) Fy 0 (4) Fz 0 (5) Using
(2), (3), (4) and (5) we obtain the stationary
point (x1, y1, z1) and f(x1, y1, z1) is the
stationary value.
137
Problem 40
The temperature T at any point (x, y, z) in space
is given by T 400 xyz2. Find the highest
temperature at the surface of a unit sphere x2
y2 z2 1.
Solution
138
(No Transcript)
139
(No Transcript)
140
Problem 41
Find the maximum and minimum distances of the
point (1, 2, 3) from the sphere (x2 y2 z2)
56.
Solution
Let A (1, 2, 3) Let P (x, y, z) be a point on
the sphere (x2 y2 z2) 56 .(1)
141
(No Transcript)
142
From (2) and (3) from (2) and (4)
Using in (1)
143
\ y 4, z 6 for x 2 y -4, z
-6 for x -2 The critical points are (2, 4,
6) and (-2, -4, -6). At (2, 4, 6), f 1 4
9 fmin 14 and the minimum distance is
. At (-2, -4, -6) f 9 36 81 \
fmin 14 and the minimum distance is
144
Problem 42
A rectangular box open at the top is to have a
volume of 32 cubit feet. Find its dimension if
the total surface area is minimum.
Solution
Let x, y and z be the dimensions of the
box. Given xyz 32 .(1) \ f(x, y,
z) xyz Let f xy 2yz 2xz (be the
surface area) Let F f lf xy
2yz 2xz l xyz
145
Solve Fx y 2z lyz 0 (2) Fy x 2z
lxy 0 (3) Fz 2y 2x lxy 0 (4)
146
From (2) and (3) From (2) and (4) x
y x 2z Using in (1)
x 4 y 4 z 2 units
147
Summary
Derivative of arc in Cartesian forms
Derivative of arc in parametric form
Derivative of arc in polar forms
148
Radius of curvature
Curvature
Formula for radius of curvature in cartesian form
Formula for radius of curvature in cartesian form
149
Formula for radius of curvature in pedal form
Formula for radius of curvature in polar form
150
Rolle's theorem If f(x) is i) Continuous in
a, b ii) Differentiable in (a, b) and iii)
f(a) f(b)
151
Lagranges Mean Value Theorem If f(x) is i)
Continuous in a, b and ii) Differentiable in
(a, b) then such that
152
Cauchy's Mean Value theorem If f(x) and g(x)
are two functions such that i) f(x) and g(x) are
both Continuous in a, b ii) f(x) and g(x) are
both differentiable in (a, b) and iii)
153
Taylor's Theorem Let f(x) be a function such
that i) f, f' , f'', ., f(n-1) are continuous
in a, b ii) f (n-1) is differentiable in (a,
b) then
154
Indeterminate form (L'Hospital Rule) If
f (x) and g (x) are such that
L'Hospital Rule can be applied to other
indeterminate forms by converting to the form
155
Taylor's Theorem for a function of two variables
156
Maclaurin's Expansion f(x, y) f(0, 0) x fx
(0, 0) yfy (0, 0)
157
Stationary point (a, b) is called a stationary
point if p fx(a, b) 0 and q fy(a, b) 0
If (a, b) is a stationary point. Then fx(a, b)
and fy(a, b) 0 and the nature of the
stationary point is representating the table.
158
Lagrange's Method of undetermined Multipliers
To find stationary values of f (x, y, z)
subject to f(x, y, z) c .(1) Step I F f
lf Step II Auxillary equations Fx 0, Fy
0, Fz 0 are formed. Step III Solve for x, y
and z using f(x, y, z) and auxillary equations.
159
- Conclusion
- Differential calculus is used in the studying
of curves and their properties - related to derivative of arcs and radius of
curvature. - We have studied Mean value theorems and their
geometrical interpretations. - Also we have learnt about the expansions of
functions using Taylor's series - expansion and Maclaurin series expansion.
- L'Hospital Rule is used to evaluating limits
in indeterminate forms. - Partial derivatives are used to obtain
stationary points and to examine their - nature.
- Lagrange's Method of Indetermined Multipliers
are used to obtain the - stationary values of a function of several
variable subject to a given - condition.
160
Problem 43
Solution
Similarly
161
Problem 44
Solution
162
Problem 45
Solution
163
Problem 46
Solution
164
(No Transcript)
165
Problem 47
Solution
166
Problem 48
Solution
167
Problem 49
Solution
168
(No Transcript)
169
Problem 50
Solution
We know that
170
Problem 51
Solution
171
Problem 52
at the point where it meets
Find the radius of curvature for
the line y x.
Solution
172
Problem 53
Show that the radius of curvature for the curve y
4 sin x sin 2x
Solution
173
Problem 54
Find the radius of curvature for xy2 a3 x3 at
(a, 0)
Solution
174
(No Transcript)
175
Problem 55
Find the radius of curvature for
at a point t
Solution
and
176
and
177
Problem 56
Find the radius of curvature for
at a point t.
Solution
178
(No Transcript)
179
Problem 57
Solution
Differentiating with respect to r we get
180
Problem 58
Solution
181
(No Transcript)
182
Problem 59
Solution
183
and also
184
Problem 60
Find the pedal equation of the polar curve
where k is a constant, and hence find the radius
of curvature at any point on the curve.
Solution
Differentiating the given equation with respect
to r we get
185
\The radius of curvature at any point on the
curve is
186
Problem 61
Verify Rolles Theorem for
in a, b where a gtbgt0.
Solution
f(x) is a product of elementary algebraic
functions which are continuous and hence it is
continuous in a, b
exists in
and also
187
Hence the three conditions of the Rolles Theorem
hold good.
Out of these values of c, since
the Rolles Theorem is verified.
188
Problem 62
Verify Rolles Theorem for
in a, b
Solution
is the sum of elementary
logarithmic functions which are continuous and
hence it is continuous in a, b
exists in
189
and similarly
Hence the three conditions of the Rolles Theorem
hold good.
Since
the Rolles Theorem is verified.
190
Problem 63
Verify LMVT for
in 0, 1
Solution
exists in (0,1) and hence f(x) is continuous in
(0, 1)
We find
i.e., both the conditions of LMVT hold good for
f(x) in 0, 1
Hence
Hence the LMVT is verified.
191
Problem 64
or
Find ? of LMVT for
Verify LMVT by finding ? for f(x) ax2 bx c
Solution
is an algebraic function hence continuous in a,
a h
exists in (a, a h)
i.e., both the conditions of LMVT hold good for
f(x) in a, a h
Then the second form of LMVT
192
Here
Using (2), (3) and (4) in (1) we have
Hence the LMVT is verified.
193
Problem 65
Apply Mean Value Theorem to show that
Solution
Consider f(x) sin-1 x in a, b
We see that
exists in (a, b)
Hence f(x) is differentiable in (a, b) and also
it is continuous in a, b.
194
Therefore, by LMVT
Since
From (1) and (2), we have
195
Problem 66
Verify the Cauchys MVT for f(x) x2 and g(x)
x4 in a, b
Solution
and
are algebraic polynomials hence continuous in a,
b
and
exist in (a, b)
also we see that
since 0 lt a lt b
i.e., the conditions of CMVT hold good for f(x)
and g(x) in a, b
196
Hence
c
i.e.,
Hence the CMVT is verified.
197
Problem 67
Verify the Cauchys MVT for f(x) ex and g(x)
e-x in a, b
Solution
f(x) ex and g(x) ex are elementary
exponential functions that
are continuous in a, b
f(x) ex and g(x) -e-x exist in (a, b)
also we see that
, since 0 lt a lt b
i.e., the conditions of CMVT hold good for
and g(x) in (a, b)
198
Hence
i.e.,
Hence the CMVT is verified.
199
Problem 68
Verify the Cauchys MVT for f(x) sinx and g(x)
cosx in
Solution
f(x) sinx and g(x) cosx in are elementary
trigonometric functions
that are continuous in
exist in
and
also we see that
200
i.e., the conditions of CMVT hold good for f(x)
and g(x) in
Hence
Hence the CMVT is verified.
201
Problem 69
Obtain a Taylors expansion for f(x) sinx in
the ascending powers of
up to the fourth degree term.
Solution
The Taylors expansion for f(x) about is
202
(No Transcript)
203
Problem 70
Substituting these in (1) we obtain the required
Taylors series in the form
Solution
Obtain a Taylors expansion for f(x) logex upto
the term containing (x-1)4
and hence find loge(1.1).
204
The Taylors series for f(x) about the point 1
is
Here
205
etc.,
Using all these values in (1) we get
Taking x1.1 in the above expansion we get
206
Problem 71
Using Taylors theorem Show that
Solution
Taking n3 in the statement of Taylors theorem,
we can write
Consider
Using these in (1), we can write,
207
For a1 in (2) we write,
Since
208
Problem 72
Obtain a Maclaurins series for f(x) sin x up
to the term containing x5
Solution
The Maclaurins series for f(x) is
Here
209
Substituting these values in (1), we get the
Maclaurins series for f(x) sinx as
Note As done in the above example, we can find
the Maclaurins series for various functions, for
ex
210
Taking m -1 in (iii)
we can get
Replacing x by (-x) in this we get
We use these expansions in the study of various
topics
211
Problem 73
Obtain a Maclaurins series for f(x) log (1
cos x) up to the term containing x4.
Solution
The Maclaurins series for f(x) is
Here
212
We may rewrite the above expression as (1 cosx)
f(x) sinx 0 (2)
Differentiating (2) we get
Differentiating (3) we get
(1 cos x) f (x) 2sinx f (x) cosx f (x)
sinx 0 . (4)
Again differentiating (4) We get
213
Substituting these in (1), we get the
Maclaurins series for
F(x) log (1 cos x) as
214
Problem 74
Obtain a Maclaurins series for f(x) log (1
ex) up to the term containing x4.
Solution
The Maclaurins series for f(x) is
Here
We may rewrite the above expression as
215
Differentiating (2) we get
Differentiating (3) we get
Again differentiating (4) We get
216
Substituting these in (1), we get the
Maclaurins series for
f(x) log (1 ex) as
217
Problem 75
Obtain a Maclaurins series for f(x) ex cos x
up to the term x4.
Solution
The Maclaurins series for f(x) is
Here
We may rewrite the above expression as
218
Differentiating (2) we get
Differentiating (3) we get
219
Again differentiating (4) We get
Substituting these in (1), we get the
Maclaurins series for
as
220
Problem 76
up to the term x4
Obtain a Maclaurins series for
Solution
The Maclaurins series for f(x) is
221
as
Substituting these in (1), we get the Maclaurins
series for
222
Problem 77
up to the term x5
Obtain a Maclaurins series for
Solution
The Maclaurins series for f(x) is
223
Substituting these values in (1) we get
224
Replacing x by x in the above we get
Using (3) and (4) in (2) we get
225
Problem 78
Obtain a Maclaurins series for f(x) x5 sinx up
to the term x10.
Solution
Hint First obtain the Maclaurins series
and hence write
226
Problem 79
Solution
227
Problem 80
Solution
228
Problem 81
Solution
229
Method 2
230
Problem 82
Solution
231
Problem 83
Solution
232
Problem 84
Solution
233
Problem 85
Solution
234
Problem 86
Solution
235
Problem 87
Solution
236
Problem 88
Solution
237
Problem 89
Solution
238
Find the value of a such that
Problem 90
is finite. Also find the value of the limit.
Solution
We can continue to apply the LHospitals Rule,
239
Problem 91
Solution
240
Problem 92
Solution
241
(No Transcript)
242
Problem 93
Problem 93
Solution
243
Problem 94
Solution
244
Obtain a Maclaurins expansion for
Problem 95
up to the 2nd degree terms.
Solution
We know that the Maclaurins series for f (x ,
y) is
245
(No Transcript)
246
Problem 96
Obtain a Maclaurins expansion for
up to the 3rd degree terms.
Solution
We know that the Maclaurins series for f (x ,
y) is
247
(No Transcript)
248
(No Transcript)
249
Problem 97
Find the extreme values of
Solution
250
(No Transcript)
251
Find the extreme values of
Problem 98
Solution
252
Find the extreme values of
Problem 99
Solution
253
254
Problem 100
Find the extreme values of
Solution
255
(No Transcript)
256
(No Transcript)