Differential Calculus - PowerPoint PPT Presentation
1 / 42
Title:
Differential Calculus
Description:
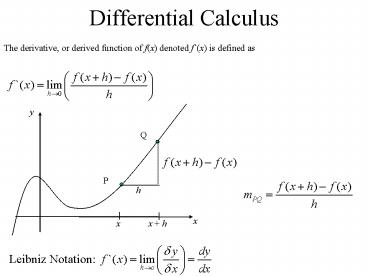
Differential Calculus The derivative, or derived function of f(x) denoted f`(x) is defined as y Q P h x x x + h The Product Rule The Quotient Rule Sec, Cosec, cot and ... – PowerPoint PPT presentation
Number of Views:2976
Avg rating:3.0/5.0
Title: Differential Calculus
1
Differential Calculus
The derivative, or derived function of f(x)
denoted f(x) is defined as
y
Q
P
h
x
x
x h
2
Differentiation from first principles
3
(No Transcript)
4
Further practice on page 29 Exercise 1A Questions
1, 4, 5 and 7 TJ Exercise 1 But not just Yet..
5
Not all functions are differentiable.
CAUTION
y tan(x).
Here, tan(x) has breaks in the graph where the
gradient is undefined
Although the graph is continuous, the derivative
at zero is undefined as the left derivative is
negative and the right derivative is positive.
For a function to be differentiable, it must be
continuous.
6
Further practice on page 29 Exercise 1A Questions
1, 4, 5 and 7 TJ Exercise 1
Differentiation reminder Page 32 Exercise 3A
Questions 1(a), (d), 2(a), (c), (d) 3(a), 4(a),
6(a) TJ Exercise 2 TJ Exercise 3
7
The Product Rule
Using Leibniz notation,
OR
8
(No Transcript)
9
(No Transcript)
10
(No Transcript)
11
Page 35 exercise 4A Questions 1, 2(b) and 3 Page
36 exercise 4B Questions 1(b), 3 and 4 TJ
Exercise 4
12
The Quotient Rule
Using Leibniz notation,
OR
13
Page 37 exercise 5A Questions 1 to 4 and 7 Page
38 Exercise 5B Questions 1 to 3 TJ Exercise 5
14
Sec, Cosec, cot and tan
Unlike the sine and cosine functions, the graphs
of sec and cosec functions have breaks in them.
The functions are otherwise continuous but for
certain values of x, are undefined.
15
(No Transcript)
16
undefined
17
(quotient rule)
Page 40 Exercise 7 questions 1, 2, 3(a), (c),
(e), (g). 4(a) TJ Exercise 6 Questions 1 to 4
18
(No Transcript)
19
Exponential and Logarithmic functions
20
Proof 1.
Let us examine this limit.
When a approaches e, the limit approaches 1.
21
Proof 2.
Differentiating both sides with respect to x.
Using the chain rule.
22
(No Transcript)
23
(No Transcript)
24
Higher derivatives
Function 1st derivative 2nd
Derivative..nth Derivative
etc. etc. etc.
25
Page 43 Exercise 8A Questions 1(b), (d), 2(b),
(c), (d), 3(a), (b),(c). 4(d), (e),
5(a), (c), (e), 6(b), (c),(e) TJ Exercise 6
Page 46 Exercise 9A Qu. 1 to 6 Review Chapter 2.1
26
Applications of Differential Calculus
Let displacement from an origin be a function of
time.
Velocity is a rate of change of displacement.
Acceleration is a rate of change of velocity.
Note The use of units MUST be consistent.
27
- A particle travels along the x axis such that
- x(t) 4t3 2t 5,
- where x represents its displacement in metres
from the origin t seconds after observation
began. - How far from the origin is the particle at the
start of observation? - Calculate the velocity and acceleration of the
particle after 3 seconds.
Hence the particle is 5m from the origin at the
start of the observation.
28
- A body travels along a straight line such that
- S t3 6t2 9t 1,
- where S represents its displacement in metres
from the origin after observation began. - Find when (i) the velocity and (ii) the
acceleration is zero. - When is the distance S increasing?
- When is the velocity of the body decreasing?
- Describe the motion of the particle during the
first 4 seconds of observation.
29
The velocity is zero when t 1 or 3 seconds.
The acceleration is zero when t 2 seconds.
30
(b) S is increasing when
Hence the distance S is increasing when t lt 1 and
when t gt 3 seconds.
(c) V is decreasing when
Hence the velocity is decreasing when t lt 2
seconds.
31
At t 0, the particle is 1m from the origin with
a velocity of 9ms-1 decelerating at a rate of
12ms-2.
At t 1, the particle is 5m from the origin at
rest decelerating at a rate of 6ms-2.
At t 2, the particle is 3m from the origin with
a velocity of -3ms-1 with zero acceleration.
At t 3, the particle is 1m from the origin at
rest accelerating at a rate of 3ms-2.
At t 4, the particle is 5m from the origin with
a velocity of 9ms-1 accelerating at a rate of
12ms-2.
32
Page 51 Exercise 1 Questions 1(a), (b), (d),
(f), 2(a), (c), (e), 3, 4, 6, 7, 8, 10, 12. TJ
Exercise 7.
33
Extreme Values of a Function(Extrema)
Critical Points
A critical point of a function is any point (a,
f(a)) where f (a) 0 or where f (a) does not
exist.
34
Consider the function
35
Critical points are
A (-2,4) f (-2) does not exist. (Right
differentiable at x -2)
A
E
B (0,0) f (0) 0. (Turning point)
D
C
C (1,1) f (1) does not exist. Left derivative
2, right derivative 1
B
D (2,2) f (2) does not exist. Left
derivative 1, right derivative 0.5
E is not a critical point as it is not in the
domain of f.
36
Local extrema
Local extreme values occur either at the end
points of the function, turning points or
critical points within the interval of the
domain.
Consider the function,
3 is the local maximum value
-1 is the local minimum value
If extrema occurs at end points then they are end
point maximums or end point minimums.
37
In short,
- Local maximum / minimum turning points
- End point values
- Critical points
The Nature of Stationary Points.
If f (a) 0 then a table of values over a
suitable interval centred at a provides evidence
of the nature of the stationary point that must
exist at a.
A simpler test does exist.
It is the second derivative test.
38
If the second derivative test is easier to
determine than making a table of signs then this
provides an efficient technique to finding the
nature of stationary points.
Page 56 Exercise 2 Questions 1, 3(a), (c), (e),
(g), (i) Page 60 Exercise 3 Questions 1(a), (c),
(e), 5(a) to (d) TJ Exercise 8
39
Optimisation Problems
Optimisation problems appear in many guises
often in the context in which they are set can be
somewhat misleading.
- Read the question at least TWICE.
- Draw a sketch where appropriate. This should
help you introduce any variables you are likely
to need. It may be that you come back to the
diagram to add in an extra x etc. - Try to translate any information in the question
into a mathematical statement. - Identify the variables to be optimised and then
express this variable as a function of one of the
other variables. - Find the critical numbers for the function
arrived at in step 4. - Determine the local extrema and if necessary the
global extrema.
40
1. An open box with a rectangular base is to be
constructed from a rectangular piece of card
measuring 16cm by 10cm. A square is to be cut
out from each corner and the sides folded up.
Find the size of the cut out squares so that the
resulting box has the largest possible volume.
x
16 2x
x
10 2x
41
Hence the cut out squares should be 2cm in
length.
42
Page 63 Exercise 4A. Note Question 8 cant be
done but try to prove me wrong!! Page 64
Exercise 4B is VERY Difficult. (VERY) TJ
Exercise 9