Boundary value problems:

1
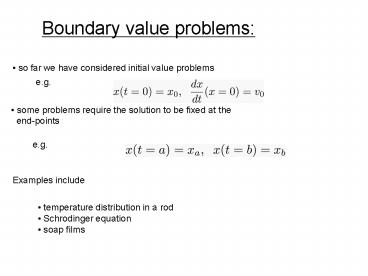
Boundary value problems

• so far we have considered initial value problems

e.g.

• some problems require the solution to be fixed
  at the
• end-points

e.g.
Examples include

• temperature distribution in a rod
• Schrodinger equation
• soap films

2
Shooting method
To solve
with boundary conditions

• assume an initial value for

• solve as an initial value problem

• vary until solution hits target

3
Time independent Schrodinger equation

• 2nd order ode

• solve for and E with boundary conditions

• normalization

• as the Schrodinger equation is linear in ,
  and homogeneous we may fix the normalization
  after finding a solution. i.e.

if is a solution, so is

• use the symmetry of V(x) to give another
  boundary condition

4
Symmetric potential

• if V(x)V(-x) then the wavefunction has definite
  parity

• even
• odd

• this fixes the boundary condition at the origin

even
odd

• due to the linearity of the Schrodinger equation
  we may always
• choose

• Use these as initial conditions in a shooting
  algorithm
• Only for certain values of E (the eigen values)
  will

5
Example simple harmonic oscillator

• introduce some rescaled variables

• then we have that

• solve as an initial value problem, using
  wavefunctions of
• either parity, to find the allowed values of

6
The potential is symmetric so look for even/odd
solutions.
shooting method
even,
7
The potential is symmetric so look for even/odd
solutions.
shooting method
odd,
8
Matching methods

• if the potential is not symmetric then the
  wavefunction does not have definite parity

• but we know that

• approximate these conditions by

For some far to the left, and far to
the right. (Must check that answers are not
sensitive to your choices.)

• pick another value such that

9
now solve from both directions

• find solution in the region
  , using initial conditions

• find solution in the region
  , using final conditions

(i.e. using the shooting method from to the
left, )
10

• rescale one of the solutions such that

• vary E until the solutions have the same slope at

11

• then the eigenfunction is given by

• the normalized wavefunction is then given by

12
Example simple harmonic oscillator

• pick an energy, En4.1

• pick co-ordinate range,

• integrate from the left and right using shooting

13
Example simple harmonic oscillator

• rescale one of the solutions,

• this is not smooth, it has a V kink, so choose
  another energy

14
Example simple harmonic oscillator

• try another energy, En6.1

• this is also not smooth, but has an inverted V
  kink.

• choose an energy between that with the V kink
  and that with the inverted V kink.

15
Example simple harmonic oscillator
After a bit of trial and error in choosing En we
get the final eigenfunction.
16
Homing in on En
In both the shooting method and the matching
method we find that the required solution is in
some region

• For the shooting method we see that picking the
  wrong En means the solution diverges to either
  or . This determines E1 and E2.
  

• for the matching method we see that picking the
  wrong En means the solution has either a V
  kink, or an inverted V kink this determines E1
  and E2.

By taking another trial energy in this range are
able to home in on the correct solution, up to
some desired accuracy. A natural choice is half
way between E1 and E2.