Building a Hypercube

1
Building a Hypercube
(tesseract)
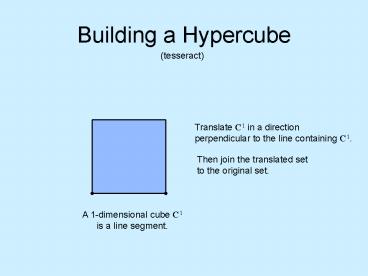
Translate C1 in a direction perpendicular to the
line containing C1.
Then join the translated set to the original set.
A 1-dimensional cube C1 is a line segment.
2
Note each edge of a 2-dimensional cube is a
1-dimensional cube.
There are 4 edges 1 from the original line
segment 1 from
the translated line segment 2 from joining
the original vertices to the translated ones.
There are 4 vertices 2 from the original line
segment 2 from the translated line segment
A 2-dimensional cube C2 is a square.
3
Translate the square in a direction perpendicular
to the plane containing the square.
Then join the translated set to the original set.
4
Note each face of a 3-dimensional cube is a
2-dimensional cube (square).
There are 6 faces 1 from the original square,
1 from the
translated square, 4 from joining the
original edges to the translated ones.
There are 12 edges 4 from the original
square, 4 from the translated square, 4
from joining the original and
translated vertices.
There are 8 vertices 4 from the original
square, 4 from the translated square,
A 3-dimensional cube C3 is a cube.
5
To get a 4-dimensional hypercube C4, translate
the cube in a direction not in the 3-space of the
cube and join the original and translated cubes.
It will have 8 cubic facets 2 from the
original and translated cubes, 6 from joining
the original and translated faces of C3.
It will have 24 square faces 6 from the
original cube, 6 from the translated cube,
12 from joining the original and translated edges
of C3.
It will have 32 edges 12 from the original
cube, 12 from the translated cube, 8 from
joining the original and translated vertices of
C3.
It will have 16 vertices 8 from the
original cube, 8 from the translated cube.
6
(No Transcript)
7
(No Transcript)
8
The 4-Dimensional Hypercube
9
The 4-Dimensional Hypercube
10
The 4-Dimensional Hypercube
11
The 4-Dimensional Hypercube
12
The 4-Dimensional Hypercube
13
The 4-Dimensional Hypercube
Place the translated cube inside the original
cube.