Randomized Block Design

1
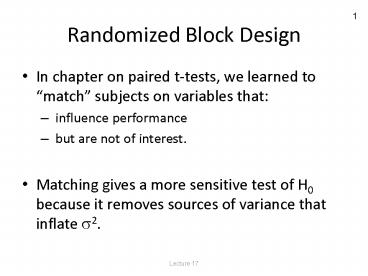
Randomized Block Design

• In chapter on paired t-tests, we learned to
  match subjects on variables that
• influence performance
• but are not of interest.
• Matching gives a more sensitive test of H0
  because it removes sources of variance that
  inflate ?2.

2
Randomized Block Design (RBD)

• In the analysis of variance, the matched subjects
  design is called the Randomized Block Design.
• subjects are first put into blocks
• a block is a group matched on some variable
• subjects in a block are then randomly assigned
  to treatments
• for p treatments, you need p subjects per block

3
Sums of squares in the RBD

• We compute SSTreat as before. Compute SSB (SS for
  Blocks) analogously
• Compute deviations of block means from grand
  mean.
• Square deviations, then add them up.
• SSTotal is composed of SSTreat SSE.
• Does SSB come from SSTreat or from SSE?

4
Where does SSB come from?
SST
SSB
SSTotal
SSE
Residual SSE
5
Conceptual Formulas

• SST Sb(XTi XG)2 p-1
• SSB Sp(XBi XG)2 b-1
• SSTotal S(Xi XG)2 n-1
• SSE SSTotal SST SSB (b-1)(p-1)
• n-b-p1
• MST SST/(p-1)
• MSB SSB/(b-1)
• MSE SSE/(b-1)(p-1) SSE/(n-b-p1)

6
Summary table

• Source df SS MS F
• Treat p-1 SSTreat SST/(p-1) MST/MSE
• Blocks b-1 SSB SSB/(b-1) MSB/MSE
• Error n-p-b1 SSE SSE/(n-b-p1)
• Total n-1 SSTotal

7
Computational Formulas

• CM (SX)2 SSTotal SX2 CM
• n
• SSTreat ST2i CM SSB SB2i CM
• b p
• SSE SSTotal SST SSB
• p of samples TiTotal for ith treatment
• b of blocks BiTotal for ith block

8
Randomized Block Design Example 1a

• 1. Five Grade 10 high school students in an
  advanced math program are tested at the beginning
  of the term. Later in the term, they write a
  midterm and then a final exam. Each test/exam
  contains similar mathematics problems, and a
  comparison will be done to see whether
  significant differences exist between mean scores
  on the 3 exams. The students scores on the exams
  are
•  
• Student First exam Midterm Final
•  
• Grumpy 78 84 82
• Sneezy 81 86 91
• Dopey 79 80 83
• Goofy 77 80 82
• Sleepy 86 91 94

9
Randomized Block Design Example 1a

• a. Is there an overall significant difference
  between mean scores on the 3 exams (? .05).
•  
• b. Although no specific predictions were made
  beforehand, after inspecting the data it could be
  seen that Sneezy consistently obtained higher
  exam scores than Goofy. Regardless of the results
  of your analysis in part (a), perform a post-hoc
  test to determine whether Sneezy and Goofy differ
  significantly on their overall average on the 3
  exams (? .05).

10
Randomized Block Design Example 1a

• H0 ?1 ?2 ?3
• HA At least two differ significantly
• Statistical test F MST
• MSE
• Rej. region Fobt gt F(2, 8, .05) 4.46

11
Randomized Block Design Example 1a

• CM 104834.4
• SSTotal SX2 CM
• 782 812 942 104834.4
• 105198 104834.4
• 363.6

12
Randomized Block Design Example 1a

• SSTreat S(Ti2) CM
• b
• 4012 4212 4322 104834.4
• 5 5 5
• 104933.2 104834.4
• 98.8

13
Randomized Block Design Example 1a

• SSB SB2i CM
• p
• SSB 2442 2712 104834.4
• 3 3
• 105075.33 104834.4
• 240.93

14
Randomized Block Design Example 1a

• SSE SSTotal SSTreat SSB
• 363.6 98.8 240.93
• 23.87

15
Randomized Block Design Example 1a

• Source df SS MS F
• Treat 2 98.8 49.4 16.55
• Blocks 4 240.93 60.23 20.18
• Error 8 23.87 2.98
• Total 14 363.6
• Decision Reject HO average scores do differ
  across exams.

16
Randomized Block Design Example 1b

• H0 ?w ?A
• HA ?W ? ?A
• (Note this is a post-hoc test. Well do N-K.)
• Statistical test Q Xi Xj
• vMSE/n

17
Randomized Block Design Example 1b

• Rank order sample means
• Sleepy Sneezy Grumpy Dopey Goofy
• 90.3 86 81.3 80.6 79.67
• Qcrit Q(4, 8, .05) 4.53

r 4
18
Randomized Block Design Example 1b

• Qobt
• 86 79.67 6.33 6.35
• v(2.984)/3 0.997
• Reject HO. Sneezy Goofy differ significantly on
  their overall average on the 3 exams.

19
RBD Example 2

• 2. People in a weight loss program are weighed at
  the beginning of the program, 3 weeks after
  starting, and 3 months after starting. The
  following are the weights (in pounds) of a random
  sample of 5 participants at each of these time
  periods.
•  
• Person Start 3 Wks 3 Months
•  
• Mickey 210 201 193
• Minnie 245 240 242
• Hewey 236 228 200
• Dewey 197 190 167
• Louie 340 328 290

20
RBD Example 2

• a. Are there significant differences between
  weights across the 3 time periods? (? .05)
•  
• b. Regardless of your answer in part a., perform
  the appropriate tests to determine at which time
  periods the participants mean weights differ
  significantly.

21
Randomized Block Design Example 2a

• H0 ?1 ?2 ?3
• HA At least two differ significantly
• Statistical test F MST
• MSE
• Rej. region Fobt gt F(2, 8, .05) 4.46

22
Randomized Block Design Example 2a

• CM 35072 819936.6
• 15
• SSTotal SX2 CM
• 2102 2452 2902 819936.6
• 855701 819936.6
• 35764.4

23
Randomized Block Design Example 2a

• SSTreat S(Ti2) CM
• b
• 12282 11872 10922 819936.6
• 5 5 5
• 821883.4 819936.6
• 1946.8

24
Randomized Block Design Example 2a

• SSB SB2i CM
• p
• SSB 6042 7272 9582 819936.6
• 3 3 3
• 852973.67 819936.6
• 33037.07

25
Randomized Block Design Example 2a

• SSE SSTotal SSTreat SSB
• 35764.4 1946.8 33037.07
• 780.5

26
Randomized Block Design Example 2a

• Source df SS MS F
• Treat 2 1946.8 973.4 9.977
• Blocks 4 33037.07 8259.27 84.656
• Error 8 780.5 97.563
• Total 14 35764.4
• Decision Reject HO weights do differ across
  the 3 time periods.