DOE 72 Checking the Randomized Complete Block Design

1
DOE 7-2 Checking the Randomized Complete Block
Design
Recall that using residuals is one way of
checking for hidden bias in an experiment. We
first used it in DOE 6-1 a

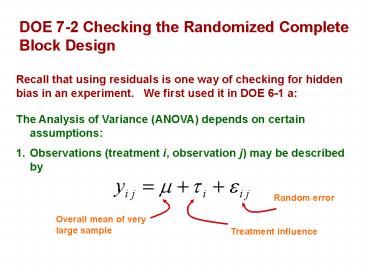
• The Analysis of Variance (ANOVA) depends on
  certain assumptions
• Observations (treatment i, observation j) may be
  described by

Random error
Overall mean of very large sample
Treatment influence
2
2. The experimental errors eij are normally and
independently distributed with mean 0 and a
constant (but unknown) variance.
In practice, these assumptions are not always
obeyed exactlyat least in a first attempt at an
experiment design. It is always wise to check
these conditions before carrying through an ANOVA
calculation. We can do this by looking at
residuals, eij yij - yi?/b where yi?/b is the
average of the ith treatment. If ANOVA can be
applied then the residuals should be normally
distributedthey should show no obvious
patterns. If the residuals follow a pattern then
the supposedly random experimental errors (eij)
are not fully random!
3
Recall the RCBD for Vascular Graft Experiment in
DOE 7-1the response variable is the percentage
of tubes in a production run without any flicks.
Residuals eij yij - yi?/6
4
yij
Probability Plot
Checking the residuals of the vascular graft
experiment.
5
Lineargood.
Good scatter.
yij
Probability Plot
Checking the residuals of the vascular graft
experiment.
6
The residuals graphed against the extrusion
pressure (left) and batch of raw materials
(right). Well scattered No apparent bias by
category.
7
Another aspect of the RCBD to be mindful of the
assumption of additivity of the model.
8
Another aspect of the RCBD to be mindful of the
assumption of additivity of the model. That is
Block effect on the experimental outcome
9
Another aspect of the RCBD to be mindful of the
assumption of additivity of the model. That is
Block effect on the experimental outcome
The additivity assumption says that the
treatment, block, and random effects are distinct
and dont interfere with each other. This might
not be true what if the block conditions
interact with one treatment but not the others?
This would produce a bias in the data set.
10
RCBD and choice of sample size (i.e., how many
blocks?) As always, increasing the number of
blocks increases the number of repetitions of
each treatment, with a resulting increased
sensitivity in the experiment (smaller a and ß
values). As before (see DOE 6-1b ANOVA and Sample
Size), we must estimate a parameter F, determined
by
to be able to use the OC curves (appendix handout
613-620) and thus find ß for a given a. The
degrees of freedom are v1 a -1 and v2
(a-1)(b-1).
11
RCBD and choice of sample size (i.e., how many
blocks?) As always, increasing the number of
blocks increases the number of repetitions of
each treatment, with a resulting increased
sensitivity in the experiment (smaller a and ß
values). As before (see DOE 6-1b ANOVA and Sample
Size), we must estimate a parameter F, determined
by
or
to be able to use the OC curves (appendix handout
613-620) and thus find ß for a given a. The
degrees of freedom are v1 a -1 and v2
(a-1)(b-1).
12
Example How many blocks are needed for the
vascular graft experiment? (The case study used
6, but were these sufficient?)
13
Example How many blocks are needed for the
vascular graft experiment? (The case study used
6, but were these sufficient?) Lets say that we
want to reject the null hypothesis if any two
treatment means differ by 6 (the table shows
yieldthe percentage of graft tubes without
flicks). The data in the table allows us to
estimate s at about 3. The number of treatments
is 4 ( a), so
14
Example How many blocks are needed for the
vascular graft experiment? (The case study used
6, but were these sufficient?) Lets say that we
want to reject the null hypothesis if any two
treatment means differ by 6 (the table shows
yieldthe percentage of graft tubes without
flicks). The data in the table allows us to
estimate s at about 3. The number of treatments
is 4 ( a), so
If b 5, F 1.58. If we set a 0.05 then with
v1 3 and v2 12, then OC curve 614 top
shows
15
ß 0.45
F 1.58
16
If b 6 then F 1.73, v1 3 and v2 15.
ß 0.25
So, the Power 1 ß 0.75 or 75
F 1.73