Chapter Opener

1
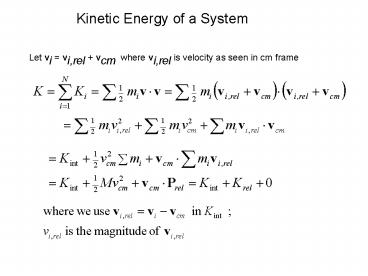
Kinetic Energy of a System
Let vi vi,rel vcm where vi,rel is velocity
as seen in cm frame
2
Momentum p of Body of Mass m, Velocity v

• momentum p mv (it is a vector tangent to
  path)
• units of momentum kgm/s Ns
• handy K p2/2m p ?(2m K)
• N2 is therefore Fnet dp/dt for constant m
• undo the derivative dp Fnet dt
• for 1d motion, or for any one component, we get

• the change in a bodys momentum in some process
  is the area under the Fnet(t) graph
• impulse J is the (vector) change in momentum in
  an interaction

3
Impulse is Change in a Bodys Momentum Dp
Dp pf - pi
pi pf
Dp
4

• top graph is precisely the slope of bottom graph
• F dp/dt
• in this case, body has stopped at the instant
  force is Fmax

• example object bounces from a perfect hard
  surface
• Dp pf - pi -.20 - (.20) -.40 kgm/s
• this number (-) is the area under the F(t) graph

5
Well do ONLY one-dimensional collisions the
line of the colliders velocities is the same as
the line of the internal contact forces
6
The 1d totally inelastic collision

• bodies are stuck together, finally vf final
  velocity
• system kinetic energy is LOST!!
• special case v2i 0 and v1i incoming
  velocity
• initial system momentum along x Pi p1i p2i
  m1v1i
• final system momentum Pf p1f p2f (m1 m2)
  vf
• apply conservation of system momentum Pi Pf

• note that in these relations v is 1d velocity
  not speed!!
• general case if both bodies are moving then v2i
  is not zero

7
The 1d elastic collision

• now system kinetic energy is conserved too
• special case v2i 0 and v1i incoming
  velocity
• initial system momentum Pi p1i p2i m1v1i
• final system momentum Pf p1f p2f m1v1f
  m2v2f
• apply conservation of system momentum Pi Pf

• apply conservation of system kinetic energy
  (assume potential energy change is zero) Ki Kf

8
Rearranging and factoring we get

• Now divide the second by the first and get

• general case if both bodies are moving then v2i
  is not zero

9
Some limiting cases for the situation v2i 0
m1 ltlt m2 little guy just bounces off
m1 ltlt m2 incomer gets halted and waiter takes off
at that speed
m1 gtgtm2 little guy takes off at TWICE incomers
speed
10
An easy collision example
A golf club head has a mass of 300 g, and it
strikes a golf ball of mass 100 g, such that the
golf ball flies away at 50 m/s. Find initial and
final speeds of golf club head. Assume the
collision is elastic Known v2f Find
v1i and v1f
11
A more challenging collision example
Snow is blowing horizontally at 20 m/s. The
density of the snow is 5 g/m3. The snow strikes
the back of an initially motionless truck ((area
8 m2) and sticks. Find before the truck is
moving (much) a) The impulse delivered to the
truck in one second, b) The force on the truck
caused by the snow
In 1 s, the snow moves 20 m, so there is a total
volume V (20 m)(8 m2) 160 m3 of snow that
strikes the truck. The total mass is M (.005
kg/m3)(160 m3) .80 kg The snow is brought to
rest, so the impulse J M Dv 16 kgm/s The
force is F Dp/Dt J/Dt (16 kgm/s)/(1 s)
16 N
12
Another more challenging collision example
9-55 Explosive bolts separate a 950-kg
communications satellite from its 640-kg booster.
If the impulse is 350 Ns, at what relative
speed do they separate?
S
B
S
B
S
B
vB
vS
System momentum is conserved, so P 0
always Thus, afterward, vS J/mS .37 m/s vB
-J/mB -.55 m/s The relative speed is therefore
vSB .37 m/s -(- .55 m/s) .92 m/s
For fun, find the kinetic energy GAIN!!