Normal Distribution

1

• Normal Distribution

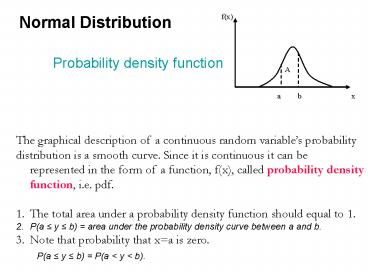
Probability density function

• The graphical description of a continuous random
  variables probability
• distribution is a smooth curve. Since it is
  continuous it can be represented in the form of a
  function, f(x), called probability density
  function, i.e. pdf.
• The total area under a probability density
  function should equal to 1.
• P(a y b) area under the probability density
  curve between a and b.
• Note that probability that xa is zero.

P(a y b) P(a lt y lt b).
2
Note

• Histogram and relative polygons can approximate
  the probability density curve.
• From the shape of the probability density curve,
  we can know the shape of the distribution such
  as skew to the right, skew to the left, bell
  shaped, multimodal or bimodal.

3
normal random variable

• Key Properties
• It is characterized by its mean ? and its
  standard deviation ?.
• The probability density function is symmetric
  about ?.
• The maximum value occurs at ?.
• The area to the left of ? is .5.
• The Standard Normal density has ? 0 and ? 1.

4
Standard Normal Probability Distribution

• A random variable that has a normal distribution
  with a mean of zero and a standard deviation of
  one is said to have a standard normal probability
  distribution.

5
Computing Probabilities

• Let X be a continuous random variable with Normal
  density (normal random variable) with parameters
  ? and ?.
• where Z is a normal random variable ? 0, ? 1.
• We sometimes write X N (?, ?) when X is normal
  random variable with parameters ? and ?.

6
How to compute probabilities

• Determine ? and ? and expression for desired
  probability, e.g. P(Xlt20), P(Xgt12), etc.
• Convert to Z, e.g. (z-score)
• Sketch a Standard normal density and shade the
  desired region.
• Use Standard Normal Table (out -gtinside) to find
  probabilities needed.
• Adjust to compute the probability of the shaded
  region

7
Examples

• 1. Let X be N (20, 5) random variable, ie ? 20
  and ? 5. Find the following probabilities
• a) P(X 23 ) (area directly above point x 23
  )
• b) P(X 23 ) (area to the left of x 23 )
• c) P(X gt 17.2 ) (area to the right of x 17.2
  )
• d)P(17.2 X 26.7 )

8
Normal Percentiles

• Definition For any continuous random variable,
  X, the p-th percentile is a number a such that
  
• P(X a) p
• Useful Formula Convert Z to X
• X ? ? Z

9
How to compute percentiles for Normal random
variables

• Sketch a Standard normal density and shade the
  given region.
• Adjust to compute the probability of the region
  that agrees with the table.
• Use Standard Normal Table (inside -gt out) to find
  the desired percentile (z-value).
• Convert z-value to X with X ? ? Z.

10
Example of Percentile Problems

• Let X be a N(20 , 5 ) random variable. Find a
  value of x such that
• P(X x ) 0.38
• P(X x ) 0.33
• P(X x ) 0.63
• P(X x ) 0.72

11
Normal Probabilities in Context

• 3. The heights of adult males in Neverland are
  normally distributed with mean 69 inches and
  variance 25 inches squared (i.e. standard
  deviation of 5 inches).
• a) Find the probability that a randomly selected
  adult male is taller than 6 feet (72 inches).
• b) Find the probability that a randomly selected
  adult male is between 5 and 6 feet tall.
• c) How tall must a male be to be among the
  tallest 10 of the population.

12

• 4. The lifetime of a certain type of television
  tube is normally distributed with mean 3.8 years
  and standard deviation of 1.2 years.
• a) Suppose that the tube is guaranteed for two
  years. What proportion of TVs will require a new
  tube before the guarantee expires?
• b) If the company wishes to set the warranty
  period so that only 10 of the tubes would need
  replacement while under warranty, how long a
  warranty must be set?

13
5.At Upton-Webber, the salaries of the employees
are normally distributed with mean 27,000 and
standard deviation 2,500.a) Mr. Smith is paid
32,000. What proportion of the employees of
Upton-Webber are paid less that Mr. Smith?b)
What proportion of the employees have their
salaries between 22,000 and 32,000?c) What
proportion of the employees have their salaries
between 30,000 and 32,000?d) Mrs. Jones claims
that her salary is high enough to just put her
among the highest paid 15 of all employees
working at Upton-Webber. Find her salary.e) Ms.
Green claims that her salary is so low that 90
of the employees make more than she does. Find
her salary.
14
End of Chapter 4
15

• Introduce the formula for calculating the Exp and
  Var
• End