Optical Measurement

1
SIGNAL PROCESSING TOOL FILTRATION,
ESTIMATION AND MODELING
U039k
V. Yatsenko, V. Boiko, P. Bidyuk, V.
Kunzevich, and O. Cheremnich - US
Collaborator - Prof. P. Pardalos
Objectives
Results
So How Does It All Work?

• New methods of signal estimation (V. Kunzevich)
• Digital filters (O. Cheremnich, V. Yatsenko, Uz.
  team)
• Models of sensor signals (O.Cheremnich,V.Boiko,
  Uz.t.)
• Adaptive filtering methods (V. Kunzevich, V.
  Yatsenko)
• Signal estimation algorithms (V. Yatsenko)
• Neural networks (P. Bidyuk)
• Compensators (O. Cheremnich, V. Yatsenko, Uz.
  team)
• Modeling of SE (V. Boiko, V. Yatsenko)
• Dynamical and information characteristics (V.
  Boiko)
• Signal processing tool (V. Boiko, V. Yatsenko)

• To simulate the sensitive element in MATLAB,
  Simulink and C.
• To obtain accurate measurements in the presence
  of noise.
• To estimate gravity as a function of time with
  reasonable accuracy.

A superconducting probe changes the signal
according to the dynamics of the sensitive
element. The optical measurement system sends out
the noise signal which depends on a position
of the levitated probe. The Signal Processing
Tool (SPT) then receives a signal..
Gravitation perturbations
Coordinates
Abstract
SPT measures mechanical coordinates with
accuracy 10 nm.
Sensitivity

• Read experimental data.
• Perform spectral analysis.
• Noise compensation.
• Filtration.
• Simulate an optical signal by adding noise.
• Estimate signal using an optimal filter.
• Estimate signal using a neural network.
• Estimate signal using an inverse system.
• Create signal with noise and test system..

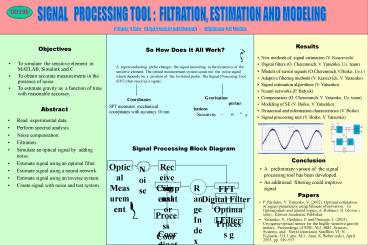
Signal Processing Block Diagram

Conclusion
Optical Measurement
Receive Signal
Noise

• A preliminary variant of the signal processing
  tool has been developed.
• An additional filtering could improve signal.

Compensator
Range Index
FFT
Papers
Digital Filter

• P. Pardalos, V. Yatsenko, V. (2002). Optimal
  estimation of signal parameters using bilinear
  observation. In Optimization and related
  topics, A. Rubinov, B. Glover ) (eds), Kluwer
  Academic Publisher.
• Yatsenko, V., Pardalos, P. and Principe, J.
  (2003), Cryogenic-optical sensor for the highly
  sensitive gravity meters, Proceedings of SPIE,
  Vol. 4881, Sensors, Systems, and
  Next-Generation Satellites VI, H. Fujisada, J.B.
  Lurie, M.L. Aten, K.Weber (eds.), April 2003, pp.
  549-557

Optimal Filter
Process Coordinates
Process g
Coordinates
g