4'2'5 NonUniform Electric Field

1
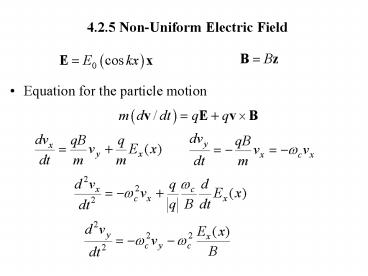
4.2.5 Non-Uniform Electric Field

• Equation for the particle motion

2
Non-Uniform Electric Field (II)

• Need to evaluate Ex(x) (Ex at the particle
  position)
• Use undisturbed orbit approximation

and
in
to obtain
3
Exercise 7

• Explain why the 1st order Taylor expansion for
  cos and sin requires krLltlt1

4
Non-Uniform Electric Field (IV)

• Use orbit averaging expecting a drift
  perpendicular to both E and B
• Velocity along x averages to zero
• Oscillating term of velocity along y averages to
  zero

• 1st order Taylor expansion for cos and sin for
  krLltlt1 yields

5
Non-Uniform Electric Field (IV)

• The orbit averaging makes the sin term to vanish
• The average of the cos term yields

• This expression was obtained as special case with
  E non-uniformity perpendicular to y and z
• The general expression for the ExB modified by
  the inomogeneity is

6
Exercise 8

• Why

has a minus sign factored while the
does not?
7
Non-Uniform Electric Field (V) Physics
Understanding

• The modification of the ExB due to the
  inomogeneity is decreasing the ExB drift itself
  for a cos(kx) field
• If an ion spends more time in regions of weaker
  E, then its average drift will be less than the
  pure ExB amount computed at the guiding center
• If the field has a linear dependence on x, that
  is depends on the first derivative dE/dx, it will
  cause contributions of weaker and larger E to be
  averaged out and the drift correction (in this
  case depending on E and dE/dx) will be zero
• Then drift correction must have a dependence on
  the second derivative for this reduced drift to
  take place

8
Non-Uniform Electric Field (VI) Physics
Understanding

• The 2nd derivative of a cos(kx) field is always
  negative w.r.t. the field itself, as required in

• An arbitrary field variation (instead of cos
  shaped) can be always expressed as a harmonic
  (Fourier) series of cos and sin functions (or
  exp(ikx) functions)
• For such a series

or in a vector form
9
Non-Uniform Electric Field (VII) Physics
Understanding

• Finally, the expression

can be then rewritten for an arbitrary field
variation as
where the finite Larmor radius effect is put in
evidence

• This drift correction is much larger for ions (in
  general)
• It is more relevant at large k, that is at
  smaller length scales

10
4.2.6 Time-Varying Electric Field

• Equation for the particle motion

11
Time-Varying Electric Field (II)

• Define an oscillating drift

• The equation for vy has been previously found as

• It can be verified that solutions of the form

apply in the assumption of slow E variation
12
Time-Varying Electric Field (III)

• The polarization drift is different for ion and
  electrons in general

• It causes a plasma polarization current

• The polarization effect is similar to what
  happens in a solid dielectric in a plasma,
  however, quasineutrality prevents any
  polarization to occur for a fixed E

13
4.2.7 Time-Varying Magnetic Field

• A time-varying magnetic field generates an
  electric field according to Faradays law

• To study the motion perpendicular to the magnetic
  field

or, considering a vector l along the
perpendicular trajectory,
14
Time-Varying Magnetic Field (II)

• By integrating over one gyration period the
  increment in perpendicular kinetic energy is

• Approximation slow-varying magnetic field
• For slow-varying B the time integral can be
  approximated by an integral over the unperturbed
  orbit
• Apply Stokes theorem

15
Time-Varying Magnetic Field (III)

• The surface S is the area of a Larmor orbit
• Because the plasma diamagnetism BdSlt0 for ions
  and vice-versa for electrons. Then

• Define the change of B during the period of one
  orbit as

• Recalling the definition of the magnetic moment m

16
Time-Varying Magnetic Field (IV)

• The slowly varying magnetic field implies the
  invariance of the magnetic moment
• Slowly-varying B cause the Larmor radius to
  expand or contract loss or gain of perpendicular
  particle kinetic energy
• The magnetic flux through a Larmor orbit is

is then constant when the magnetic moment m is
constant
17
Time-Varying Magnetic Field (V) Adiabatic
Compression

• The adiabatic compression is a plasma heating
  mechanism based on the invariance of m
• If a plasma is confined in a mirror field by
  increasing B through a coil pulse the plasma
  perpendicular energy is raised (heating)

18
4.3 Particle Motion Summary

• Charge in a uniform electric field

• Charge in an uniform magnetic field

yields the Larmor orbit solution
where
19
Particle Motion Summary (II)

• Charge in Uniform Electric and Magnetic Fields

produces the ExB drift of the guiding center

• Charge Uniform Force Field and Magnetic Field

produces the (1/q)FxB drift of the guiding
center
20
Particle Motion Summary (III)

• Charge in Motion in a Gravitational Field

produces a drift of the guiding center (normally
negligible)
21
Particle Motion Summary (IV)

• Charge Motion in Non Uniform Magnetic Field
  Grad-B Perpendicular to the Magnetic Field

the orbit-averaged solution gives a grad B drift
of the guiding center
22
Particle Motion Summary (V)

• Charge Motion in Non Uniform Magnetic Field
  Curvature Drift due to Curved Magnetic Field
• The particles in a curved magnetic field will be
  then always subjected to a gradB drift
• An additional drift is due to the centrifugal
  force

23
Particle Motion Summary (VI)

• Charge Motion in Non Uniform Magnetic Field
  Grad-B Parallel to the Magnetic Fieldin a mirror
  geometry, defining the magnetic moment

the orbit-averaged solution of
provides a force directed against the gradB
24
Particle Motion Summary (VII)

• Charge Motion in Non-Uniform Electric Field

the orbit-averaged solution produces
25
Particle Motion Summary (VIII)

• Charge Motion in a Time-Varying Electric Field

the solution in the assumption of slow E
variation
yields a polarization drift that is different
for ions and electrons
26
Particle Motion Summary (IX)

• Charge Motion in a Time-Varying Magnetic Field
  solution of

in the perpendicular (w.r.t. B) plane and under
the assumption of slow B variation shows a motion
constrained by the invariance of the magnetic
moment