Force Between Two Parallel Currents

1
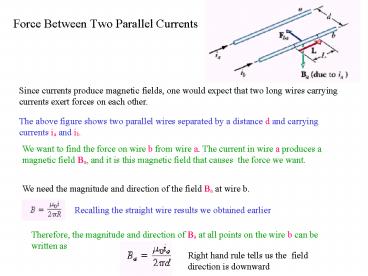
Force Between Two Parallel Currents
Since currents produce magnetic fields, one would
expect that two long wires carrying currents
exert forces on each other.
The above figure shows two parallel wires
separated by a distance d and carrying currents
ia and ib.
We want to find the force on wire b from wire a.
The current in wire a produces a magnetic field
Ba, and it is this magnetic field that causes
the force we want.
We need the magnitude and direction of the field
Ba at wire b.
Recalling the straight wire results we obtained
earlier
Therefore, the magnitude and direction of Ba at
all points on the wire b can be written as
Right hand rule tells us the field direction is
downward
2
Force Between Two Parallel Currents
Knowing the magnetic field from wire a, we can
find the force it exerts on the wire b. Recalling
the force on a wire equation from an external
magnetic field
F i L x B
We can then write the force Fba that exerts on a
length L of wire b by the field Ba is
As shown in the figure, the vectors L and Ba are
perpendicular. So the above equation becomes
The direction of Fba is L x Ba, which is directly
toward wire a.
3
Force Between Two Parallel Currents
The general procedure for finding a force on a
current-carrying wire
We use the same method to compute the force on
wire a due to the current in wire b. We would
find the force points directly toward b,
therefore the two wires with parallel currents
attract. If the two currents were anti-parallel,
we could show that the two wire repel each other.
It should be noted that the force acting between
currents is the basis of the definition for the
Ampere, the SI unit for current.
4
Rail Gun
As a current i is setup through the rail gun, the
current rapidly causes the conducting fuse to
vaporize.
The current produces magnetic field B between the
rails, and the field causes a force F to act on
the conducting gas, which is part of current
path.
The gas propels the projectile along the rails,
accelerating it by as much as 5x106 g, and then
launching it with a speed of 10km/s, all with in
1ms
5
Check Quiz
6
Amperes Law
Ampere's law is the magnetic equivalent of
Gauss's law.
It is different in that it refers to a closed
loop and the surface enclosed by it (rather than
a closed surface and the volume enclosed by it,
as is the case with Gauss's Law).
Consider a closed loop, not necessarily a circle,
which is broken into small elements of length DSi
with a magnetic field Bi at each element.
S
I
The sum over each element length, times the
component of the magnetic field along the
direction of the element, is proportional to the
current I that passes through the loop.
S
7
Amperes Law
Recall, that we can find the net electric field
due to any distribution of charges with the
inverse-square law for the differential field dE,
but if the distribution is complicated, we may
have use numerical methods.
If the distribution has planar, cylindrical, or
spherical symmetry, we can use Gausss Law to
find electric field with far less effort.
Similarly, we can find the net magnetic field due
to any distribution of current with the
inverse-square law for the differential dB, but
again if the distribution is complicated, we may
have to resort to numerical methods with a
computer.
If the distribution has some symmetry, we might
be able to use Amperes Law to find the magnetic
field with far less effort.
8
Amperes law tells us that the integral of the
tangential component of the magnetic field around
any closed path is proportional to the net
current that pierces the loop. Mathematically,
for any closed path
where ds is an infinitestial displacement vector,
tangent to the path.
The integral on the left side is path integral
around a closed loop, called an Amperian loop.
The current on the right side is the net current
through the surface that is bounded by the loop.
For example, if the loop is formed by the edges
of the screen, then ienc is the net current
through the screen
9
Amperes Law

An obvious application of Ampere's law is to a
long straight current-carrying wire.
The figure on the right shows a long wire that
carries a current i directly out of the page.
From an earlier derivation, we have shown that
the magnetic field B produced by the current has
the same magnitude at all points that are same
distance r from the wire, that is, the field has
cylindrical symmetry about the wire. So we
encircle the wire with a concentric circular
Amperian loop of radius r and field has same
magnitude at all points on the loop. The
integration will be counter-clockwise since the
current direction is out of the page. Since B is
tangent to loop at points on the loop, the
quantity
Bds Bds cos? Bds, since ?0 at all points
on the loop
10
Amperes Law
Applying Amperes Law
to the Amperian Loop we obtained the following
The resulting path integral is proportional to
the enclosed current
which can be re-written as
This is exactly what we got integrating
Biot-Savarts equation for an current in an
infinite wire
11
Amperes Law
The figure shows the cross-section of a long
straight wire of radius R that carries a
uniformly distributed current i directly out of
the the page. Since the current in uniformly
distributed about the center of the wire, the
magnetic field B that it produces must be
cylindrical symmetric
To find the magnetic field inside the wire, we
can again use an Amperian loop of radius r as
shown in the figure, where r lt R. Also symmetry
suggest that the magnetic field is tangent to the
loop. Therefore the path integral becomes
Since the current is uniformly distributed we can
write the current enclosed as
Equating of both sides Amperes Law, we have
12
Amperes Law
This can be re-written as
Thus, inside the wire, the magnitude of the
magnetic field in proportional to r, that the
magnitude is zero at the the center and a maximum
at the surface where rR
13
Solenoids and Toroids
Let consider the magnetic field produced by the
current in a long, tightly wound helical coil of
wire, which is called a solenoid.
The figure shows a section through a portion of a
stretched-out solenoid. The solenoids magnetic
field is the vector sum of the fields produced by
the individual turns. Points very close to the
each wire, the magnetic field behaves like that
produced from a single wire, but closer
inspection shows the fields between adjacent
wires cancel. It also shows that point near the
central axis is approximately parallel. In the
limiting case (ideal solenoid), the field inside
the coil is uniform and parallel to the solenoid
axis
14
Solenoids
The figure to the near left clearly illustrates
how the external fields cancel each other while
the internal filed add. The purple arrows
represent fields produced from lower part of the
turns while the pink arrows represents fields
produced from the upper part of the turns. As
shown these two contributions tend to cancel each
other out. In the limiting case of the ideal
solenoid, the external field is zero.
15
Solenoids
Lets apply Amperes Law
to the rectangular Amperian loop abcd in the
ideal solenoid in the figure, where B is uniform
within the solenoid and zero outside it. We can
write the integral as the sum of four integrals,
one for each side
16
Solenoids
The c to d integral must be zero since the
external field is zero
The d to a and b to c integrals must be zero
since the internal B-field is perpendicular to ds
the path element, that is, Bds Bds cos?, ??/2
, Bds 0.0
The only non-zero path integral is from a to
b,which yields a value of
Bh
The net current encircled by the rectangular
Amperian Loop is
Let n be the number of turns per unit length and
i is the current in the turns
17
Solenoids
Amperes law gives us
Therefore, the B field inside an ideal solenoid is
Even though this is derived for an ideal case,
the result holds quite well for real solenoids
only at interior points.
18
Toroids
Figure (a) shows a toroid, which we may describe
as a solenoid bent into a doughnut. From the
symmetry we see the field forms concentric
circles inside the toroid as shown in figure (b).
Lets choose a concentric circle of radius r as
an Amperian loop and integrate clockwise.
Amperes Law yields
where i is the current and N is the total number
of turns