Single step methods

1
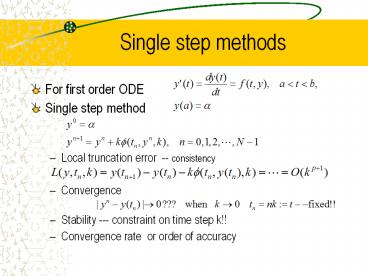
Single step methods

• For first order ODE
• Single step method
• Local truncation error -- consistency
• Convergence
• Stability --- constraint on time step k!!
• Convergence rate or order of accuracy

2
Convergence

• An example
• Forward Euler method
• Exact solution
• Numerical solution
• The error

3
Convergence

• For single-step method
• Thm Suppose the order of accuracy of the above
  single step method is pgt0 and the incremental
  function satisfying the
  Lipschitz condition for y, i.e.
• In addition, suppose be exact.
  Then we have the global truncation error
• Proof See details in class of as an exercise

4
Applications

• For forward Euler method
• Convergence if f(t,y) is Lipschitz continuous for
  y!!
• RK2 RK4
• Convergence if f(t,y) is Lipschitz continuous for
  y!!

5
Stability

• In computation, we have round-off error!!!
• Def For a numerical method, if there is a
  perturbation at step , then the
  perturbation at all steps after is
  not larger than . Then the method is called as
  stable.
• Analyze the stability of numerical methods
• Use the model problem
• Apply the method to this problem
• Find the amplification factor

6
Stability

• For forward Euler method
• For model problem
• The method
• The amplification factor
• Stability condition stability region

7
Stability

• For backward Euler method
• For model problem
• The method
• The amplification factor
• No stability condition for k (unconditionally
  stable!!) stability region

8
Stability

• Trapezoidal method -- unconditionally stable
• RK4
• For implicit methods
• Unconditionally stable!!
• For explicit methods
• Stability condition
• I-Stable methods
• RK3, RK4, implicit methods

9
Numerical example

• Conclusion
• There is no stability condition for Backward
  Euler method
• There is stability condition for Forward Euler
  and RK4
• Choice of time step
• Accuracy
• For explicit methods
• Must satisfying the stability condition!!

10
Numerical example

• The problem

11
Time-splitting (split-step) method

• The problem
• Integrate over time integral
• Formal exact solution

12
Time-splitting method

• First order splitting method
• Step 1 Solve
• Step 2 Solve
• Approximation to the original problem
• Local truncation error (see details in class)

13
Time-splitting method

• Second order splitting method (Strang splitting)
• Step 1 Solve
• Step 2 Solve
• Step 3 Solve
• Local truncation error (see details in class)

14
Time-splitting method

• Comments
• When A B are commute, the splitting methods are
  exact!!
• They are very useful in solving PDEs
• For each subproblem, we can solve them either
  analytically or numerically
• For dispersive problems, we can design high order
  splitting, e.g. 4th order or 6th order splitting
  methods
• For dissipative problems, usually, we can only
  use second order splitting method.

15
Integration factor (IF) method

• The problem
• Moving the linear term to the left hand side
• Multiplying at both sides

16
Integration factor (IF) method

• Integrating over time interval
• Multiplying both sides by
• Apply numerical quadrature to the last term
• An example

17
Multi-step methods

• The problem
• An m-step multistep method is one whose
  difference equation for finding the approximation
  at the time step can be
  represented as
• Constants
  to be determined

18
Multi-step methods

• Explicit method
• Implicit method
• Ways to determine the constants
• Taylor expansion for local truncation error
• Function interpolation via polynomial

19
Multi-step methods

• Adams-Bashforth (AB) method explicit,
  (r1)-step
• Choose r1 interpolation nodes
• Construct a polynomial based on the
  above nodes
• Numerical methods
• Order of accuracy Stability
• Explicit

20
Multi-step methods

• Two-step Adams-Bashforth (AB2) method r1
• 2 interpolation points
• Interpolation polynomial
• Numerical method
• Order of accuracy 2 Explicit Stability
  region (see details in class)

21
Multi-step methods

• Four-step Adams-Bashforth (AB4) method r3
• 4 interpolation points
• Interpolation polynomial
• Numerical method
• Order of accuracy 4 Explicit Stability
  region (exercise)

22
Multi-step methods

• Adams-Moulton (AM) method implicit, (r1)-step
• Choose r2 interpolation nodes
• Construct a polynomial based on the
  above nodes
• Numerical methods
• Order of accuracy Unconditionally stable
• Implicit

23
Multi-step methods

• Two-step Adams-Moulton (AM2) method r1
• 3 interpolation points
• Interpolation polynomial
• Numerical method
• Order of accuracy 3 Implicit Unconditionally
  stable!!

24
Multi-step methods

• Four-step Adams-Moulton (AM4) method r3
• 5 interpolation points
• Interpolation polynomial
• Numerical method
• Order of accuracy 5 Implicit Unconditionally
  stable!!

25
Multi-step methods

• Adams-Bashforth methods
• Explicit
• Stability condition
• Adams-Moulton methods
• Implicit
• Unconditionally stable
• Same number of points with one order high
  accuracy
• Predictor-corrector methods
• Combine the advantages of both AB and AM methods
• Use AB methods to predict an intermediate value
• US AM methods to correct the prediction
• Usually, we use the same steps AB and AM methods

26
Multi-step methods

• Adams two-step predictor-corrector method
• AB2 for prediction
• AM2 for correction
• Properties
• Explicit, second order accurate, better stability
  than AB2!!