Conservation of Charge

1
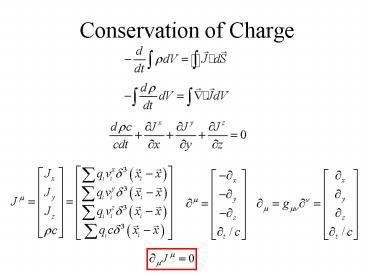
Conservation of Charge
2
Lorentz Transformation of Current
Example Lorentz transform from charges rest
frame
Total charge is invariant!
3
Lorentz Transformation of a Sheet of Charge
Sheet of charge
Length contraction
Moving charge
4
Lorentz Transformation of Sheet of Current
Sheet of current
See problem sets
Where does this come from?
5
Maxwells Equations
6
On the Way to the Wave Equation
7
Choice of Gauge
Freedom to choose
Lorentz gauge
0
The Wave Equation!!!
8
GaugeTransformation
Gauge Transformation
We want
We have
Therefore
Just a solution to Laplaces equation.
9
Lorentz Gauge 4 Vectors
Lorentz Condition
10
Lorentz Transforms of Vector Potential
But you must transform the positions also!
11
Single Charge Example
Potential in charges rest frame
Potential in moving frame
Change to moving frame coordinates
12
Single Charge Example (continued)
Evaluated at t0.
13
E Field Direction
Direction of E
Always radial!
14
E Field Magnitude
15
E Field Magnitude
Ex
Ex on x axis
Increasing b
x
Ey
Ey on y axis
Increasing b
y
16
Wave Equation
17
Electric and Magnetic Fields
4
n 1
m 1
4
18
Transformation of Field Tensor
But remember, you must change the positions also!
19
Maxwells Charge Source Equations
Maxwells Source Equations
20
Example Sheet of Charge
Sheet of charge
Transformed fields
In moving frame
21
Example Sheet of Current
Sheet of current
Transformed fields
In moving frame
Gausss and Amperes Laws
22
Maxwells Monopole Source Equations
Dual Tensor
23
Gauge Transformations
24
Summary of EM Equations
Conservation of Charge
Lorentz Condition
Wave Equation for Potentials
Field Equations
Maxwells Charge Equations
Maxwells Monopole Equations
Gauge Transformations