Notes on 2'1 Quadratic Functions

1
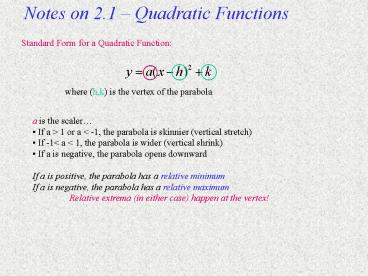
Notes on 2.1 Quadratic Functions
Standard Form for a Quadratic Function
2
In most cases, in order to get the quadratic into
standard form so you can investigate it, you must
COMPLETE THE SQUARE.
4
- 8
The vertex is (-2,-1).
Note that this is also a relative min (since the
parabola opens upward).
3
EX Find the vertex and intercepts of the
parabola
Step 1 Complete the square to find the vertex
9
9
The vertex is (3,1)
Step 2 Finding x-intercepts. SET Y 0
Step 3 Find y-int by plugging 0 in for x in
original problem. In this example, y-int (0, -8)
The x-intercepts are (2,0) and (4,0)
4
Find the vertex and intercepts of the given
parabola.
Check first to see if the equation is factorable
before completing the square.
We dont need to complete the square ?. Our
vertex is (3, 0). What are our intercepts?
X-int (3,0) at vertex Y-int (0, 18)
5
Find the vertex and intercepts of the parabola.
6
EX Find the equation for the parabola with a
vertex at (1,2) and through the point (3,-6)
Step 1 Start with standard form and fill in
what you know
Step 2 All you need is a use the other point
to find it.
So in standard form, your equation is
7
Assignment page 143 1-8,13-33 odd,39-45
odd,57,59,84-89
8
Notes on 2.2 Polynomials of Higher Degree
Leading Coefficient Test look at the highest
power of x in the equation. It will tell you
what the graph is doing on the left and right of
the graph.
Since the highest power is 3 the graph will act
like an x3 on its ends
x3 falls on the left and rises on the right
9
Since the highest power is 4, and since that term
is negative, it will act like a x4 graph
which falls on the right and on the left.
10
Finding zeros
EX Write an equation that has zeros at 3 and 4.
11
(No Transcript)
12
Assignment page156 1-8,17-26,27-47 odd,53-60

• falls left, rises right
• falls left, falls right
• falls left, rises right
• rises left, rises right
• rises left, falls right
• f(x) x2 8x
• f(x) x2 x 20
• f(x) x3 9x2 14x
• 60. f(x) x5 5x3 4x

13
2.3 Graphing Polynomials Objective To use
both long division and synthetic division to
find the factors and the zeros of
polynomials To use the zeros of polynomials
and their multiplicities of graph polynomials
14
Notes on 2.3
We begin with long division
15
(No Transcript)
16
(No Transcript)
17
Notes on 2.3 Synthetic Division
8 -14 1 5
So, x-1 is a factor of the dividend because there
is no remainder.
18
What is the y-intercept of the function?
19
-2
-6
24
-46
-48
3
-12
23
1
Is x2 a factor of the dividend? Is -2 a zero
of the function?
Before you get too excited synthetic division
only works with LINEAR divisors
20
8
-6
-5
8
-6
0
-5
Continue factoring.
Set equal to zero
21
So your choices are limited to
Which would you try first? (I was lucky.)
22
Find all rational zeros of the function
23
Find all the rational zeros of
24
Assignment page 170 7-27odd, 39-43 odd, 55-67
odd, 100-107
25
Assignment page 170 8-18 even,100-107
26
Review for 2.1-2.3 Quiz
b) Does this equation have a maximum or a
minimum? If so, what is it?
c) Find the x-intercepts of the parabola. If
they are irrational give exact answers.
d) Find the y-intercept of the parabola. If it
is irrational, give exact answers.
27
Review for 2.1-2.3 Quiz
2. Write the equation for the parabola with a
vertex of (5,8) and passing through the
point (11,-4).
28
Review for 2.1-2.3 Quiz

• Using the Leading Coefficient Test, describe the
  behavior ofthis graph on the left and right.
• Use synthetic division to find the x-intercepts
  of the graph.

29
Review for 2.1-2.3 Quiz
30
Notes on Graphing Polynomials with Real Roots
The multiplicity of a root is how many times that
roots corresponding factor appears in the
factorization of a polynomial.
x 0, multiplicity of 3 x 2, multiplicity of
2 x -5, multiplicity of 1
Note If this equation was multiplied out, it
would have a leading power of
31
So what good is multiplicity?
The multiplicity of a root helps you by telling
you how the graph behaves at that root.
We also know that since these are the only roots,
these are the only places the graph can cross the
x-axis.
32
Previous EX
x 0, multiplicity of 3 x 2, multiplicity of
2 x -5, multiplicity of 1
cut/wiggle bounce cut/straight
Question How did I know where to start drawing
the graph???
Left/right behavior!
33
EX Use x-intercepts and multiplicity to graph

• List your roots
• x 3
• x -2
• x 1
• x -6

• Give the multiplicity
• 4
• 2
• 3
• 1

• State what happens
• bounces/flat
• bounces/not flat
• cut/wiggle
• cut/straight

34
Oooo bad news you have to factor!!
35
EX Use x-intercepts, multiplicity, and
left/right behavior to graph
36
Assignment
WORKSHEET!!!!
37
Notes Graphing Reciprocal Equations by hand
EX Graph

• Notes
• There are no roots (zeros,x-intercepts) for this
  equation because it will never 0 (the top will
  never 0).
• There are, however, places where the graph is
  undefined (where the bottom 0) these are

vertical asymptotes!!

• At asymptotes, the graph can either flip from
  positive to negative or keep the same sign.
• If the multiplicity is odd, the graph flips over
  the asymptote.
• If the multiplicity is even, the graph does not
  flip.

38
x 0 is a FLIP asymptote, b/c the multp. of 0 is
odd.
x 0 is a NO FLIP asymptote, b/c the multp. of 0
is even.
While were here notice the left/right behavior
of these graphs. The first approaches y 0 as a
horizontal asymptote from the top side on both
the left and the right. The second approaches y
0 also, but on the left it does so from the
bottom.
39
So back to this thing
This has no x-intercepts (it wont cross the x
axis). It DOES have vertical asymptotes
x 0 x 2 x -5
These all have a multiplicity of 1, so each is a
flip asymptote.
40
EX Graph
What happens?
Behavior
List asymptotes
41
New example
What happens?
Behavior
List asymptotes
42
Notes Graphing Rational Functions the whole 9
yards
using x-intercepts, asymptotesand behavior.
EX Graph
What happens?
What happens?
List zeros
List asymptotes
Behavior

• flips
• flips

• x 2
• x -3

• cut/wiggle
• bounce

• (0,0)
• (1,0)

down on left up on right
43
EX Graph
What happens?
What happens?
List zeros
List asymptotes
Behavior
44
EX Graph
What happens?
What happens?
List zeros
List asymptotes
Behavior
NOTE TO SELF If the power on top power on
bottom, you have a horizontal asymptote get the
coefficients!! Then, test a large pos and a
large neg in the equation to see which side of
the asymptote youre on (below or above)
45
EX Graph
What happens?
What happens?
List zeros
List asymptotes
Behavior
NOTE TO SELF If there is a common factor on top
and bottom, that is neither a zero nor an
asymptote. It is a HOLE. Cancel the factor and
continue normally just remember to put a hole on
your graph for that x value.
46
EX Graph
What happens?
What happens?
List zeros
List asymptotes
Behavior
NOTE TO SELF If there is a common factor on top
and bottom, and there is a left-over piece in the
numerator, there is a hole on the x-axis at the
point where the factor equals zero. The behavior
at the x-intercept depends on the multiplicity
after you have canceled out the matching factor.
47
EX Graph
What happens?
What happens?
List zeros
List asymptotes
Behavior
NOTE TO SELF If there is a common factor on top
and bottom, and there is a left-over piece in
the denominator, there is an asymptote where the
factor equals zero. The behavior at the
asymptote depends on the multiplicity of the
factor after you have canceled out the matching
factors.
48
Assignment
WORKSHEET!!!!
49
Notes 2.4 Complex Numbers

• All real numbers are members of the complex set
  (because they can all be written in the form of a
  complex number with b 0)
• If a 0, the number is called pure imaginary.
• Complex numbers appear rarely in applications
  however, they are vitally important in electronic
  circuitry. You would not have color TV without
  complex numbers!

50

• To add or subtract, you combine the real parts
  and combine the imaginary parts
• (2 3i) (4 5i) 6 2i
• (2 3i) - (4 5i) -2 8i
• To multiply, FOIL as usual then simplify any i n
  powers and combine like terms
• (2 3i)(4 5i) 8 10i 12i 15i2
• 8 2i 15 23 2i
• To divide, multiply by the conjugate of the
  denominator. That will remove the i from the
  bottom

51
More Examples Ex. Find the product of the number
and its conjugate. a. b.
Ex. Perform the operation and write the result in
standard form. a. b.
Ex. Simplify the complex number and write it in
standard form. a. b.
52
Powers of i i 2 -1 i 3 i i 2 - i i 4
i 2 i 2 -1 -1 1 i 5 i 3 i 2 -i
-1 i i 6 i 3 i 3 -i -i i 2
-1 etcetcetc
EX Simplify i 30.
Assignment pg 180 A 15-35 odd, 37-38,39-65
odd, 85-87, 90-95 B (higher level) 15, 24, 39,
41, 45, 51, 57, 61, 63, 67, 71, 72, 81, 83,
85, 86, 96
53

• Quick Even Answers for pg 180 (both assignments
  A B)
• 24.
• not 4i
• The graph is up three on the vertical (imaginary)
  axis.
• False. It equals 1.
• 90. a)
• b)
• x-int y-int (0, 6)
• No x-intercepts. y-int (0, 5)
• 96. Let x equal amount withdrawn and replaced.
  x 1 liter.

54
Notes Finding Complex Solutions to Polynomials

• The degree (highest power) of a polynomial always
  tell you how many solutions the polynomial has.
• Multiplicity may seem to affect the number of
  solutions remember, solutions can repeat and
  count as more than one.
• The solutions could be real or complex.

This has 1 real solutions (it has 2 complex
solutions)
This has 2 real solutions (one has mult. of 2)
This has 3 real solutions
All of these graphs technically have 3 solutions
they are all cubic equations.
55
EX Find all the solutions of
56
NOTE TO SELF Due to the ? in the quadratic
formula, complex solutions always come in
conjugate pairs!!!
57
Thought Process
Since one of the solutions is 3i, and since
complex solutions always come in conjugate pairs,
-3i must also be a solution.
So two of the factors must be (x 3i) and (x
3i)
Multiply these together (x 3i)(x 3i)
x2 9
You are left with a quadratic that you can either
factor or use the Quad Form on.
58
EX Find a polynomial that has zeros at 1, 2i,
and 3 i
Recall the NOTE TO SELF complex solutions always
come in conjugate pairs!
59
Assignment page 187 A 14-58 even, 77-80 B
(extra challenge) 14-48 mult of 4, 50-58
even, 66-70all, 77-80
60
Quick Answers to pg 187 14-58 even, 77-80

• Zeros factored form
• Zeros factored form
• Zeros factored form
• Zeros factored form
• Zeros factored form
• Zeros factored form
• Zeros factored form
• Zeros factored form
• Zeros factored form
• Zeros factored form

34. 36. 38. 40. 42. 44. 46. 48.

• a.
• b.
• c.
• a.
• b.
• c.

54. 56. 58. 78. 80.
61
Review for Chap 2 Test
62
(No Transcript)
63
(No Transcript)
64
(No Transcript)
65
(No Transcript)
66
Graphing