Speed of Sound Waves

1
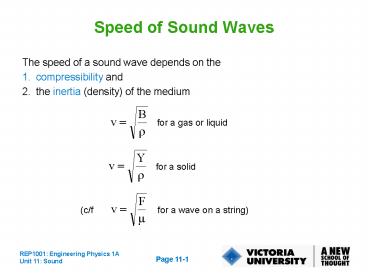
Speed of Sound Waves

• The speed of a sound wave depends on the
• compressibility and
• the inertia (density) of the medium

2
Speed of Sound Waves

• Example 1 Calculate the speed at which a sound
  wave travels down an aluminium bar (Y 7.0 x 1010
  N/m2 , ? 2.7 x 103 kg/m3 )

5.1?103 m/s 5.1 km/s
Example 2 Calculate the speed of sound in water
(B 2.1 x 109 N/m2 , ? 1.0 x 103 kg/m3)
1.5?103 m/s 1.5 km/s
3
Speed of Sound Waves

• Speed of sound in various materials (m/s)

4
Mathematical Representation of Longitudinal
(Sound) Waves

• Consider a long narrow tube containing a gas or
  liquid, which has a movable piston at one end.

The piston moves forward, increase in pressure
and density (compression or condensation).
When the piston stops moving, compressed region
continues to move forward with a speed v.
The piston moves backwards, pressure and density
decreases (rarefaction).
If the piston oscillates sinusoidally then a
series of condensations and rarefactions follow
each other in a regular pattern down the tube
with a speed v.
5
(No Transcript)
6
Mathematical Representation of Longitudinal
(Sound) Waves
If D(x,t) is the displacement of an element of
the fluid from its equilibrium position
then D(x, t) DMsin(kx-?t) where DM is
the maximum displacement of the fluid from its
equilibrium position. (DM is thus the
displacement amplitude)
The variation in pressure of the fluid from its
equilibrium pressure, ?P, is given by ?P
?PMcos(kx-?t) where ?PM is the maximum change in
pressure from the equilibrium pressure. (?PM is
thus the pressure amplitude)
Maximum change in pressure is ?PM 2?f?v?DM
7
Intensity of Sound Waves

• The power transmitted by a sound wave is given by

Introduce concept of Intensity of the sound wave
defined as power per unit area
8
Intensity of Sound Waves

• Example The faintest sound that the human ear
  can detect at a frequency of 1000 Hz (the
  threshold of hearing) has an intensity of about
  10-12 W/m2. What is the pressure and
  displacement amplitudes for such a sound.?
  (Assume v 343 m/s and ? 1.29 kg/m3)

(Compare with air pressure which is about 105
N/m2 !)
9
Intensity of Sound Waves

• Exercise Repeat this problem for the threshold
  of pain which has an intensity of approximately
  1.00 W/m2.

Answer ?PM 30 N/m2
DM 1.1 x 10-5 m
10
The Decibel Scale of Intensities

• The human ear can detect a wide range of sound
  intensities.

• It does not respond to each intensity in a linear
  manner.

• Introduce a logarithmic scale where the sound
  level is defined in terms of the intensity of the
  sound by

11
The Decibel Scale of Intensities

• Example 1 Calculate the sound level (dB) of the
  threshold of hearing and the threshold of pain.

Threshold of hearing I 10-12 W/m2
? ?10log(10-12/10-12?
10log(1) 0 dB
Threshold of pain I 1.0 W/m2
? ?10log(1.0/10-12?
10log(1012) 120 dB
12
The Decibel Scale of Intensities

• Typical sound levels
• Whisper 30dB
• Normal Conversation 50 - 60dB
• Busy Traffic 80dB
• Motor Mower 100dB
• Rock Concert 110 - 120 dB
• Jet Engine 130 -150 dB

13
The Decibel Scale of Intensities

• Example 2 Calculate the difference in sound
  levels between two sounds, one of which has
  double the intensity of the other.

Here I?I? 2
10?0.301 3.01 dB
? ? 10log(2)
This example illustrates that for the sound
intensity to double the sound level goes up by
approximately 3 dB.
14
Spherical Sound Waves

• Sound from a point source spreads out in a series
  of spheres from that point source.

As the wave fronts spread out they become larger.
The power must be distributed over a surface area
of 4?r2 where r is the distance from the source.
The sound intensity a distance r from the point
source is
If I1 is the intensity at a distance r1 from the
source and I2 is the intensity at a distance r2
then
15
Spherical Sound Waves

• Intensity as a function of radial distance from
  point source

1/r2 dependence
16
Spherical Sound Waves

• Example A rock group is playing in a studio.
  Sound emerges from the open door and spreads
  uniformly in all directions. If the sound level
  5m from the door is 80 dB, at what distance from
  the door should the sound be barely audible (0
  dB) ?

Let I1 intensity at 5m and I0 intensity at
the distance we want to find.
17
Doppler Effect

• When a sound source and observer move towards
  each other the frequency of the sound observed is
  higher than that emitted by the source.

When a sound source and observer move away from
each other the frequency of the sound observed is
lower than that emitted by the source.
Whilst the effect is the same, the mathematics
depends on whether the source or the observer is
moving with respect to the air
18
Doppler Effect

• Case 1 Observer moves directly towards a
  stationary sound source with a speed v0.

19
Doppler Effect

• Case 1 Observer moves directly towards a
  stationary sound source with a speed v0.

Frequency of sound is f, wavelength is ? and the
speed of the sound is v f ?
The speed of the observer is v0
As the observer moves towards the sound source,
the speed of the waves relative to the observer
increases to a new value v v v0 but the
wavelength is unchanged.
20
Doppler Effect

• Case 2 Sound source moves directly towards the
  observer with a speed of vs .

21
Doppler Effect

• Case 2 Sound source moves directly towards the
  observer with a speed of vs .

The observer sees the wavefronts as bunched up.
The observed wavelength ?is shorter than the
wavelength of the source.
The observed wavelength is shortened by the
distance that the source travels in one cycle.
In that time the source travels a distance vsT
vs/f
But
22
Doppler Effect

• Combing these two case in a general expression
  gives

Where the upper signs represent toward the
lower signs represent away from
23
Doppler Effect

• Example An Australian and an American submarine
  travelling at 50 km/hr and 70 km/hr respectively
  are approaching each other head on. The
  Australian submarine sends out a 1000 Hz sonar
  signal to the American sub. Sonar waves travel
  at 5470 km/hr in salt water.
• (a) Calculate the frequency heard by the American
  Sub

Observer American Sub, Source Australian Sub.
24
Doppler Effect

• Example An Australian and an American submarine
  travelling at 50 km/hr and 70 km/hr respectively
  are approaching each other head on. The
  Australian submarine sends out a 1000 Hz sonar
  signal to the American sub. Sonar waves travel
  at 5470 km/hr in salt water.
• (b) Calculate the frequency heard by the
  Australian Sub in the signal reflected back to
  it by the American Sub.

Observer Australian Sub, Source American Sub.
The frequency that bounces off the American sub
is f 1022 Hz
25
Shock Waves

• The shape of the wavefront is dependent on the
  speed of the object

vobj 0
vobj lt vsound
vobj vsound
vobj gt vsound
26
Shock Waves

• When the sources vobj is moving faster than the
  wave speed, vsnd the wave fronts generates an
  envelope in the shape of a cone with an apex half
  angle of ? where

The ratio vobj/vsnd is referred to as the Mach
Number and the cone formed is called the shock
wave.