Expected Value Decision Making:

1

• Expected Value Decision Making
• Medical decision-making problems often can not
  be solved by reasoning based on patho-physiology.
  Clinicians need a method for choosing among
  treatments when the outcome of a treatment is
  unpredictable, as are the results of a surgical
  procedure.
• Let us consider the following example
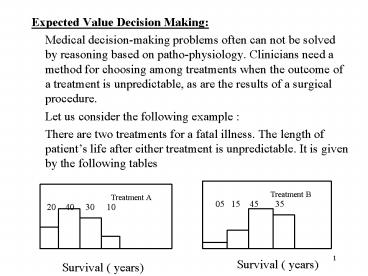
• There are two treatments for a fatal illness.
  The length of patients life after either
  treatment is unpredictable. It is given by the
  following tables

Treatment B
Treatment A
05 15 45 35
20 40 30 10
Survival ( years)
Survival ( years)
2

• Each treatment is associated with uncertainty.
  Regardless the treatment, the patient will die
  after 4 years. There is no way to know which year
  will be his last
• Which treatment is preferable?
• A choice among treatments is choice among
  gambles.
• How should you choose among the gambles?
• We shall propose a method for choosing- called as
  expected value decision making Characterize
  each gamble by one number and use this number to
  compare gambles.

3

• The ideal criterion for choosing a gamble should
  be a number that reflects preference for the
  outcomes of the gamble.
• Utility number is the name given to a measure of
  preference that has a desirable property for
  decision making.
• The gamble with the highest utility value should
  be preferred
• We can perform this calculation for both
  treatments.
• The mean survival rate for treatment A 1 x
  0.22 x 0.43 x 0.34 x 0.1 2.3 years.
• Similarly, for treatment B it will be 3.1 years
• If the length of life is our decision, treatment
  B should be selected.
• Representing Choices with Decision Tree
• The choice between treatments A and B is
  represented diagrammatically in the Figure.
  Events that are under control of a chance node
  are represented by a chance node ( circle). Each
  line represents an outcome.

4

• Associated with each line is the probability of
  the outcome occurring. We can calculate the mean
  survival for the chance node. The average length
  of life is called expected survival.

p 0.05
p 0.20
Survival 1 year
Survival 1 year
p 0.40
p 0.15
Survival 2 years
Survival 2 years
p 0.45
Exp value 3.1 years
p 0.30
Survival 3 years
Exp value 2.3 years
Survival 3 years
p 0.35
p 0.10
Survival 4 years
Survival 4 years
Treatment B
Treatment A
A chance node representation for survival rates
for two possible treatments
5

• To use expected value of decision making. We
  follow this strategy when there are treatment
  choices with uncertain outcomes.
• 1. Calculate the expected value of each decision
  alternatives, then
• 2. Pick the alternative with the highest expected
  value.
• Performing the Decision Analysis
• We extend the concept of expected-value of
  decision making by introducing the concept of
  decision analysis. There are four major steps in
  the decision analysis
• 1. Create a decision tree this step is the most
  difficult, because it requires formulating the
  decision problem, assigning probabilities, and
  measuring the outcomes.
• 2. Calculate the expected value of each decision
  alternative.
• 3. Choose the decision alternative with the
  highest expected value.
• 4. Use sensitivity analysis to test the
  conclusions of the analysis.

6

• Many health professionals balk when they learn
  about the technique of decision analysis, because
  they recognize the possibility of errors in
  assigning values to both the probabilities and
  the utilities in a decision tree.
• The first step in the decision analysis is to
  create a decision tree that represents the
  decision problem. Consider the following decision
  problem
• The patient is Mr. Danby, 66-years old, who is
  crippled with arthritis of both knees severe
  enough for free movement. In addition to it he
  has emphysema, a disease in which lungs lose
  their ability to exchange oxygen and CO2. He is
  considering knee-replacement surgery
• In the surgery there is a risk he may not survive
  the operation.
• Possible outcomes could be death from the
  infection, death from procedure, poor mobility
  and full recovery.

7
Operative death
Operative death
Surgery
Infection
Survival
Full mobility
Survival
No infection
Poor mobility
No Surgery
Decision tree for knee- replacement surgery. The
square represents the decision node and circle
represent chance nodes
8

• Mr. Danbys internist is familiar with the
  decision analysis. Using the conventions of the
  decision analysis, the internist sketches the
  decision tree. Square box denote the decision
  node and lines from the decision nodes represent
  actions.
• p( full recovery after the surgery ) 0.6,
• p( partial recovery after the surgery) 0.4.
• p( operative death) 0.05, p(survival )
  0.95
• p( post-surgery infection) 0.05, p(no
  infection) 0.95
• These probabilities could be very subjective at
  times.
• For the decision analysis to work, you have to
  assign utility values for the each outcome.
• The possible outcomes are
• Full mobility, Poor mobility ( if infection
  develops after the operation
• Wheel chair bound Death

9

• It is important that assessment of utilities is
  done properly.
• Assessment of Utilities
• It is more complex than assigning probabilities.
  For some therapies, these values may be
  available. However, there are some outcomes, such
  as physical and mental disabilities, prolonged
  hospitalization, pains and death for which it is
  difficult to assign utility values.
• You have to assign reasonable utility values for
  all of these.
• All the positive and negative aspects of the
  outcomes must be considered in assessment of the
  utility values.
• Proper units have to be developed to consider the
  degree of pains, adverse drug reactions and
  death.

10

• Though, the assessment of probabilities is
  exclusive responsibility of physicians, the
  assessment of utilities must be done in
  cooperation with the patient and his family.
  Some individual may take higher risks to return
  to normal life.
• The main advantage of the decision analysis is
  that construction of decision tree alone forces
  the physician to think of various outcomes in
  the diagnosis, which is often ignored
  anticipating all the possible outcomes of a given
  condition.
• In clinical studies, where formal construction of
  tree appears warranted, some methods are
  available for simplifying the tree and
  calculations. Some of the tree branches can be
  pruned without any significant problems.

11

• Outcome for the surgery
• Survival Years of full function
• (years) Functional status equivalent to
  outcome
• 10 Full mobility 10
• ( successful surgery)
• 10 Poor mobility 6
• ( status quo or unsuccessful surgery)
• 10 Wheal chair-bound 3
• (the outcome if a second surgery is necessary
• 0 Death 0

12
(No Transcript)
13

• The following calculations are performed for node
  A
• 1. Calculate the expected value of operative
  death after the surgery ( prosthesis). Multiply
  this by utility factor ( 0.05 x 0 0 )
• 2. Calculate the expected value of surviving
  surgery(prosthesis) 3 x 0.95 2.85
• 3. Add the expected value calculated in steps 1
  and 2 to obtain the expected of developing
  infection 0 2.85 2.85.
• Perform the similar calculations for node B ( 0.6
  x 10 0.4 x 6) 8.4
• Obtain the expected value of surviving
  knee-replacement surgery ( node C ) as follows
• 1. Multiply the expected value of infected
  prosthesis by the by the value of probability of
  infection 2.85 x 0.05 0.143
• 2. Multiply the expected value of no infection by
  the probability of no infection 0.95 x 8.4
  7.98

14

• 3. Add the expected value calculated in steps 1
  and 2 , which gives 0.143 7.98 8.123.
• Perform the expected value of chance node, by
  the same procedure for the node D ( 8.135 x 0.95
  0 x 0.05 7.7 ).
• The conclusion
• For surgery, Mr Danby average life expectancy,
  measured in terms of normal mobility is 7.7 .
  This does not mean Mr. Danby is guaranteed 7.7
  years of mobile life by accepting the surgery.
• However, it means that if the physician had 100
  similar patients who underwent the surgery, the
  average number of mobile years would be 7.7 years
• However, by accepting no surgery, the average
  length of life ( equivalent to full mobility )
  will be 6 years. Some patients may live longer,
  and some shorter.
• This is the principle of the decision analysis.

15

• Performing Sensitivity Analysis
• Sensitivity analysis is a test of validity of the
  conclusions of the analysis over a wide range of
  assumptions about the probabilities and values of
  utilities.
• There is often a wide range of reasonable
  probabilities that a physician could use with
  equal confidence.
• The sensitivity analysis is used to answer this
  question
• Do my conclusions regarding the preferred choice
  change when the probability and outcome estimates
  are assigned values that lie in a reasonable
  range?
• To come up with a satisfactory answer to this
  question, the decision analysis is performed for
  a reasonable probabilities (range)and outcome
  ranges and the expected value of decisions are
  examined in the light of various outcomes.
• The appropriate action is taken.
• The sensitivity value should be small.

16

• .

Expected Years of Healthy Life
25 is threshold
17

• .

Expected Years of Healthy Life
20 is threshold
18

• Sensitivity Analysis
• Data from clinical studies often represent only
  an approximation of the probability of a given
  outcome.
• Therefore, it is essential to study the effects
  of changing some of the probabilities.
• If the decision proves to be very sensitive to
  one or more of the values substituted, an
  additional data gathering or more careful
  consideration of existing such data may be
  necessary.
• Unfortunately, the sensitivity analysis is very
  tedious. If you have to test a large number of
  probabilities and utilities.
• However, there are standard computer based
  techniques available which can automatically
  identify sensitive variables and flag them. To
  obtain any meaningful results, we have to ensure
  that all the sensitive variables are estimated
  very carefully.

19

• Treat, Test or Do Nothing?
• The physician who faces a diagnostic challenge
  and has evaluated one patients symptoms. He must
  then choose among the following actions
• 1. Do nothing further ( neither perform
  additional tests nor treat the patient.
• 2. Obtain additional diagnostic information
  (test) before choosing whether to treat.
• 3. Treat without waiting for more information.
• This decision is simplified when the physician
  knows the true state of the patient and the
  further testing is unnecessary. At this stage the
  doctor needs only to assess the tradeoffs among
  various therapeutic actions.
• Deciding among treating, testing, and doing
  nothing sounds difficult, but you have learnt the
  principles to solve this kind of problem. There
  are three steps

20

• 1. Determine the treatment-threshold probability
• 2. Determine the pre-test probability of the
  disease.
• 3. Decide whether a test result could affect
  affect your decision to treat.
• The treatment-threshold probability is the
  probability of the disease at which you are
  indifferent between treating and not treating.
  Above the threshold, you should treat.
• Do not order a test unless it could change the
  management of your patient.
• Example
• Your patient has a possible blood clot in the
  vessels of lungs (plumonary embolus). You have
  assigned the pretest probability as 0.05 and the
  treatment threshold is 0.10.
• If you obtain no further information, you would
  treat the patient if the pretest probability was
  above 0.10

21

• You decide whether a positive lung scan would
  raise the probability of the disease to above the
  threshold level(0.10)
• You have reviewed the literature and learnt the
  true positive rate of the lung scan is 0.75 and
  the false positive rate of 0.25.
• A negative test scan would decrease the posttest
  probability below threshold. A positive test will
  move the probability towards the threshold and
  could alter your decision to treat the patient.
• You therefore use Bayes theorem to calculate the
  posttest probability before ordering the test.

22

• Decision Analysis ( Further examples)
• Let us first consider a non clinical example to
  show the application of the decision analysis.
• Example
• The quarterbacks team has the possession of the
  ball on the 18th yard line of his opponent and
  with one minute left in the game and his team
  trails by 4 points. It is fourth down, with 5
  yards to go for a first down.
• Analysis
• The choices are to pass, kick a field goal, or
  attempt a running play. Before the quarterback
  can decide which choice to make, he must know
  what the outcome choices may be. A decision tree
  representing the choices and possible outcome has
  to be constructed.
• At the square node, the choice is in the hand of
  decision maker and at the circular node, the
  outcome is dictated by a chance.

23

• Next, the quarterback must assess the likelihood
  of each outcome. The probabilities are indicated.
• In order to obtain some index of the worth of
  each outcome, utility values are to be assigned.
• Is should be apparent that the relative worth of
  each of the alternate course of action is
  function of both the probability of the outcome
  and the value of the utility.
• Even though the probability of the field goal is
  0.9, its value is limited, because it generates
  only 3 points and fails to win the game.
• The best choice is the one with highest expected
  value of the event.
• The highest value is 350 units, and corresponds
  to a complete pass.

24
0.48
0.06
0.03
0.03
25
(No Transcript)
26
350
133.2
0.48
0.03
18.0
0.90
0.02
94.2
0.15
-25
0.78
0.03
0.02
27

• Example of Decision Theory in a clinical problem
• A 24 year-old women had both her kidneys removed
  and she received a kidney transplant. A
  splenectomy was carried out to correct
  leukopenia. Recently she is admitted to hospital
  with many complications. The diagnosis of
  subdiaphragmatic abscess was considered at this
  time, and the possibility of surgery is
  considered (suspected of having subphrenic
  abscess). The decision tree is shown in the
  figure.
• The choices are - either to operate or not to
  operate and the consequences of both choices are
  to be described explicitly with all the
  probabilities
• The expected value of the surgery is 62.5 units
  and that of no surgery is 81.1 unit.
• Since the expected value of no surgery exceeds
  the expected value of the surgery, the best
  solution is that to treat the patient medically.

28
(No Transcript)
29
89.2
36.8
59.8
60.6
21.3
62.5
63.8
37.0
81.1
0.30
0.55
0.70