Task Scheduling Problem Description

1
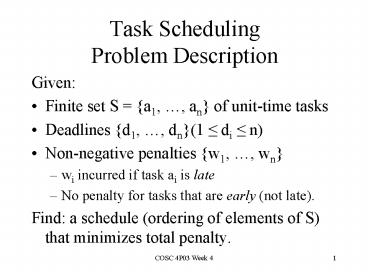
Task SchedulingProblem Description

• Given
• Finite set S a1, , an of unit-time tasks
• Deadlines d1, , dn(1 di n)
• Non-negative penalties w1, , wn
• wi incurred if task ai is late
• No penalty for tasks that are early (not late).
• Find a schedule (ordering of elements of S) that
  minimizes total penalty.

2
Task Scheduling Algorithm and Example

• A ?
• n 0
• sort S into non-increasing order by penalty wi
• for each x in S (taken in non-increasing order)
• if Nt(A U x) t for all 1tn1
• A A U x
• n n1
• return A

3
Approximation Algorithm for TSP

• Triangle inequality c(u,w) c(u,v) c(v,w)
• A simple 2-approximation algorithm assuming
  triangle inequality is satisfied
• Select a vertex r as root
• Compute an MST T for G from root r
• Visit the vertices of T in preorder
• Visit r
• Running time O(V2)

4
Combinatorial Structures - Graphs
Adjacency Matrix
List of Edges 1,2, 1,3, 1,6, 2,3,
2,4, 2,6, 2,7, 3,4, 3,5, 3,6, 3,7,
4,7, 5,6, 5,7, 6,7
5
Combinatorial Structures Incidence Matrix of
Graph
List of Edges 1,2, 1,3, 1,6, 2,3,
2,4, 2,6, 2,7, 3,4, 3,5, 3,6, 3,7,
4,7, 5,6, 5,7, 6,7
6
Combinatorial Structures Projective Plane of
Order 2
Incidence Matrix
List of Lines 1,2,4,2,3,5,3,4,6,4,5,7,5
,6,1,6,7,2,7,1,3
7
(7,4,3) Hamming Code Generator Matrix
List of Codewords
8
Lexicographic Order Subsets of 1, , n

• Algorithm to find rank of subset T
• FindRank(n, T)
• rank 0
• for (i 1 i lt n i)
• if i is in T
• rank rank 2(n-i)
• return rank

9
Lexicographic Order Subsets of 1, , n

• Algorithm to find subset that has rank r
• Unrank(int n, int r)
• T
• for (i n i gt 1 i--)
• if ((r 2) 1)
• T T U i
• r r/2 // integer division!
• return T