Integration by Parts

1
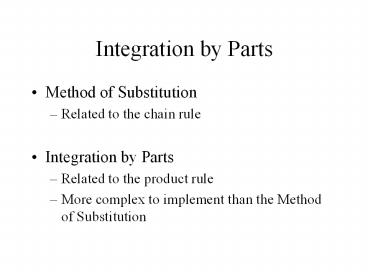
Integration by Parts

• Method of Substitution
• Related to the chain rule
• Integration by Parts
• Related to the product rule
• More complex to implement than the Method of
  Substitution

2
Derivation of Integration by Parts Formula
Let u and v be differentiable functions of x.
3
Derivation of Integration by Parts Formula
Let u and v be differentiable functions of x.
(Product Rule)
4
Derivation of Integration by Parts Formula
Let u and v be differentiable functions of x.
(Product Rule)
(Integrate both sides)
5
Derivation of Integration by Parts Formula
Let u and v be differentiable functions of x.
(Product Rule)
(Integrate both sides)
(FTC sum rule)
6
Derivation of Integration by Parts Formula
Let u and v be differentiable functions of x.
(Product Rule)
(Integrate both sides)
(FTC sum rule)
7
Derivation of Integration by Parts Formula
Let u and v be differentiable functions of x.
(Product Rule)
(Integrate both sides)
(FTC sum rule)
(Rearrange terms)
8
Integration by Parts Formula

• What good does it do us?
• We can trade one integral for another.
• This is only helpful if the integral we start
  with is difficult and we can trade it for a good
  (i.e., solvable) one.

9
Classic Example
10
Helpful Hints

• For u, choose a function whose derivative is
  nicer.
• LIATE
• dv must include everything else (including dx).