Complex exponential form of Fourier series

1

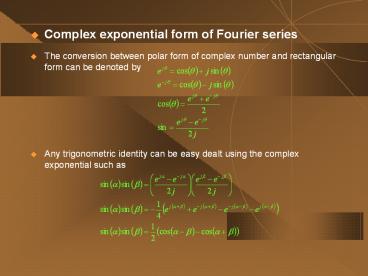
• Complex exponential form of Fourier series
• The conversion between polar form of complex
  number and rectangular form can be denoted by
• Any trigonometric identity can be easy dealt
  using the complex exponential such as

2

• We know that the complex exponential functions
• formed an orthonormal set basis functions.
• Then we can write the signal representation
• The coefficients cm can be determined
• Since the basis functions are complex, we really
  have to take notice of the conjugate in the
  definition on how to find the coefficient of the
  basis function.

3

• The magnitude and phase of the exponential
  Fourier series coefficients of a real signal
• There is important thing to note about the above
  equation is that if x(t) is real, then the right
  hand side must also be real. If we write
• it is apparent that the only way the imaginary
  part of the phasor can be cancelled perfectly
  for all t is by the phasor since they rotate
  at the same speed.

4

• Thus we require
• since the sum of a complex number and its
  conjugate is always real.
• The mathematical concept of a negative frequency
  phasor to cancel it, since this means that
• Now we can plot in magnitude or phase form.
  Since the magnitude is an even function and
  since the phase of the Fourier series
  spectrum is an odd function.

5

• It is common to refer to the Fourier series
  produced by the sine/cosine expansion as a single
  sided spectrum (i.e. only positive frequencies)
  and that by the complex exponentials as a double
  sided spectrum (i.e. positive and negative
  frequencies).
• Relating the complex exponential Fourier series
  to the sine - cosine form
• The sine - cosine Fourier series of a real signal
  and its exponential form
• where is the spacing of the harmonics in
  radian/sec and is actual frequency of a
  harmonic.
• Substituting the above equation and the previous
  equation we obtain
• So this confirms the statement that for a real
  signal .

6

• It also gives a simple way of changing between
  the coefficients of sine-cosine Fourier series
  and the exponential kind.
• EXAMPLE
• Sin-cos Fourier series for a pulse train with a
  period T, amplitude A and pulse width d

7

• Firstly calculate the DC value of the signal
• Calculate the coefficients of the cosine basis
  function
• where

8

• Calculate the coefficients of the sine terms