More coherence

1

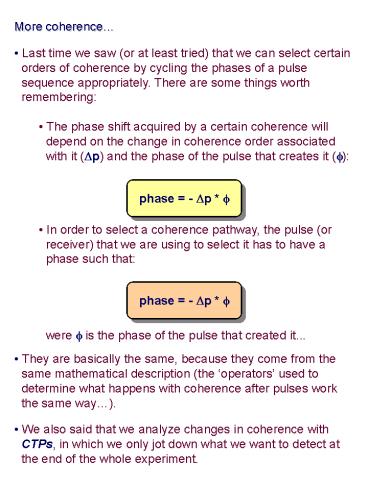
• More coherence
• Last time we saw (or at least tried) that we can
  select certain
• orders of coherence by cycling the phases of a
  pulse
• sequence appropriately. There are some things
  worth
• remembering
• The phase shift acquired by a certain coherence
  will
• depend on the change in coherence order
  associated
• with it (Dp) and the phase of the pulse that
  creates it (f)
• In order to select a coherence pathway, the
  pulse (or
• receiver) that we are using to select it has to
  have a
• phase such that

phase - Dp f
phase - Dp f
2

• Multiple quantum filters
• Weve been saying for a while now that in a
  coupled spin
• system there are zero- (p 0), single- (p
  ?1),
• double- (p ?2), etc-quantum transitions. In a
  three-spin
• J-coupled system we have

bbb
p 0
p ? 1
bba
bab
abb
p ? 2
aab
baa
aba
p ? 3
aaa
3

• Multiple quantum filtered COSY (MQF-COSY)
• For example, the CTP for selecting
  double-quantum
• coherence or higher is

90
90
90
p 2 p 1 p 0 p - 1 p - 2
4

• MQF-COSY (continued)
• A normal COSY of a certain sample with singlets,
  doublets
• and other multiplets may look like this. This
  is not selecting
• any coherence in particular
• Now, if we use a MQF sequence with the phase
  cycle set to
• select DQF, singlets would disappear
  (single-quantum)

5

• MQF-COSY ()
• If we design the appropriate phase cycle, we can
  select only
• coherence orders p gt 2, and this would filter
  also double-
• quantum transitions. Doublets and their
  correlations would
• disappear from the 2D spectrum

6

• 13C-13C correlations - INADEQUATE
• These are all homonuclear correlations with 1H.
  Why not with
• 13C? Obvious reasons. The diagonal
  (single-quantum) is
• 99 times bigger than any cross-peak. We would
  need a truck-
• load of sample and even then seeing the small
  peaks is hard.
• What we do is use MQ filters to remove
  single-quantum stuff
• (isolated 13C signals), and select only the
  double quantum
• transitions (the J13C-13C coupling).
• The pulse sequence is INADEQUATE (Incredible
  Natural
• Abundance DoublE QUAntum Transfer Experiment),
  and
• looks like this

180y
90x
90x
90f
t1
D
D
13C
y
7

• INADEQUATE - CTP
• Just for fun, we can write down the CTP for the
  experiment
• in order to see which coehrences we are
  selecting

180y
90x
90x
90f
t1
D
D
13C
2 1 0 - 1 - 2
Pulse 1,3 0 3 2 1 4 7 6 5 2 5 4 3 6 1 0 7 3 6 5
4 7 2 1 0 1 4 3 2 5 0 7 6 0 3 2
1 4 7 6 5 2 5 4 3 6 1 0 7 3 6 5 4 7 2 1 0 1 4 3 2
5 0 7 6 Pulse 2 0 3 2 1 4 7 6 5 2 5 4 3 6 1 0
7 3 6 5 4 7 2 1 0 1 4 3 2 5 0 7 6
4 7 6 5 0 3 2 1 6 1 0 7 2 5 4 3 7 2 1 0 3 6 5 4
5 0 7 6 1 4 3 2 Pulse 3 0 1 2 3 0 1 2 3 0 1
2 3 0 1 2 3 0 1 2 3 0 1 2 3 0 1 2 3 0 1 2 3
0 1 2 3 0 1 2 3 0 1 2 3 0 1 2 3 0 1
2 3 0 1 2 3 0 1 2 3 0 1 2 3 Receiver 0 0 0 0 0
0 0 0 2 2 2 2 2 2 2 2 1 1 1 1 1 1 1 1 3 3 3 3 3 3
3 3 0 0 0 0 0 0 0 0 2 2 2 2 2 2
2 2 1 1 1 1 1 1 1 1 3 3 3 3 3 3 3 3
8

• INADEQUATE
• For a singlet, the first three pulses will put
  magnetization in
• the ltzgt axis in lt-zgt, pointing down. Now, if
  we do a 0, 1, 2,
• 3 cycle for the fourth pulse, we get
• If we put the receiver running backwards (0, 3,
  2, 1), we will

y
y
1
3
x
x
y
y
4
2
x
x
y
y
180y D (1 / 4J)
90x D (1 / 4J)
x
x
9

• INADEQUATE (continued)
• The third is, for now, Divine intervention, and
  creates DQC
• Now we use the same phase cycle as before for
  the last pulse
• and the receiver. The change in phase for the
  DQF is twice as
• for SQF (remember that fcoherence - Dp
  fpulse )

y
Evolution of DQF
90x
90f
x
y
y
1
3
x
x
y
y
4
2
x
x
10

• INADEQUATE (continued)
• So, what the heck happened in t1? We cannot see
  it because
• it is DQ, and vectors wont cut it. However, we
  can more or
• less explain it if we look at the 2-spin system
  energy diagram
• As weve seen before, DQ transitions involve
  processes that
• have frequencies equal to the sum of the
  frequencies from

bb (1/2, 1/2)
wC2
(-1/2, 1/2) ab
ba (1/2, -1/2)
wDQ
wC1
wDQ wC1 wC2
aa (-1/2, -1/2)
11

• INADEQUATE ()
• For an example we had seen before, the 2D plot
  would look
• like this

1
4
2
3
5
6
7
6-7
5
3
7
4
5-6
2
6
1
4-5
2-3
3-4
1-2
12
How pulse programs really look like cosydf arx-
version 2D COSY with double quantum filter A.
Wokaun R.R. Ernst, Chem. Phys. Lett. 52, 407
(1977) U. Piantini et al., J. Am. Chem. Soc.
104, 6800 (1982) A.J. Shaka R. Freeman, J.
Magn. Reson. 51, 169 (1983) d03u d134u 1 ze 2
d1 3 p1 ph1 d0 d20 p1 ph2 d13 p1 ph3
d20 go2 ph31 d1 wr 0 if 0 id0 zd lo to 3
times td1 exit ph10 2 1 3 1 3 0 2 1 3 2 0 2 0
1 3 ph20 2 1 3 2 0 1 3 1 3 2 0 3 1 2 0 ph30 0 0
0 1 1 1 1 1 1 1 1 2 2 2 2 ph310 0 2 2 0 0 2 2 1
1 3 3 1 1 3 3 tl0 transmitter power level
(default) p1 90 degree transmitter high power
pulse d0 incremented delay (2D)
3 usec d1 relaxation delay 1-5
T1 d13 short delay (e.g. to compensate delay
line) 3 usec d20 to enhance intensity of
DQC in0 1/(1 SW) 2 DW nd0 1 NS 8
n DS 2 or 4 td1 number of experiments MC2 QF
DQF-COSY in a Bruker ARX-500
Pulse program
Pulse 1...
Pulse 2...
Pulse 3...
Receiver...
13
inad arx-version 2D INADEQUATE p2p12 d03u
d41s/(cnst34) d1220u d12 dl5 d12 cpd 1
ze 2 d1 3 p1 ph1 d4 p2 ph2 d4 p1 ph1
d0 p1 ph3 go2 ph31 d1 wr 0 if 0 id0 zd
lo to 3 times td1 d4 do exit ph1(8) 0 3 2 1 4
7 6 5 2 5 4 3 6 1 0 7 3 6 5 4 7 2 1 0 1 4 3 2 5 0
7 6 ph2(8) 0 3 2 1 4 7 6 5 2 5 4 3 6 1 0 7 3 6 5
4 7 2 1 0 1 4 3 2 5 0 7 6 4 7 6 5 0
3 2 1 6 1 0 7 2 5 4 3 7 2 1 0 3 6 5 4 5 0 7 6 1 4
3 2 ph3 0 1 2 3 ph31 0 0 0 0 0 0 0 0 2 2
2 2 2 2 2 2 1 1 1 1 1 1 1 1 3 3 3 3 3 3 3
3 tl0 transmitter power level (default) dl5
decoupler power level for CPD/BB decoupling p1
90 degree transmitter high power pulse p2 180
degree transmitter high power pulse p31 90
degree pulse for slave timer (cpd-sequence) d0
incremented delay (2D) 3
usec d1 relaxation delay 1-5 T1 d4
1/(4J(CC)) d12 delay for power switching
20 usec in0 1/(2 SW(X))
DW(X) nd0 1 NS 32 n DS 4 td1 number of
experiments MC2 QF cpd cpd-decoupling
according to sequence defined by cpdprg
INADEQUATE in a Bruker ARX-500
Pulse program
Pulse 1...
Pulse 2...
Pulse 3...
Receiver...
14

• Summary
• The phase cycle IS what makes the multiple pulse
  sequence
• to do what we want.
• By designing an appropriate pulse cycle we can
  select
• magnetization associated with certain types of
  coherence.
• This means that we can select different type of
  signals and
• filter unwanted stuff from the spectrum.
• In the MQF-COSY we only look at things that can
  give us
• coherence order equal or higher than the filter
  we are using.
• In the INADEQUATE experiment we select
  double-quantum
• 13C-13C coherence, and therefore allows us to
  see 13C-13C
• coupled pairs.
• We are still looking at the 13C-satellites, so
  we need a boat-
• load of sample.