Tutorial on Symmetry and Symmetry operation

1
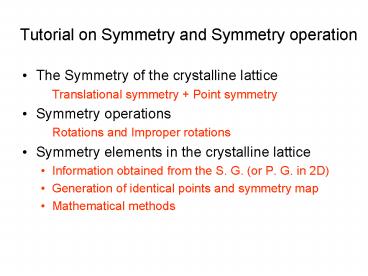
Tutorial on Symmetry and Symmetry operation

• The Symmetry of the crystalline lattice
• Translational symmetry Point symmetry
• Symmetry operations
• Rotations and Improper rotations
• Symmetry elements in the crystalline lattice
• Information obtained from the S. G. (or P. G. in
  2D)
• Generation of identical points and symmetry map
• Mathematical methods

2

• 1. Prove that for conventional crystal lattice,
  there are only 1, 2, 3, 4, and 6 fold rotation
  axis.

3
This is actually the direct deduction of the
basic symmetry property of the crystalline lattice
Assuming point A and B are the two closest
lattice points in a crystal, and the lattice
vector a is determined by AB . There is
an n fold rotational axis perpendicular to the
paper plane, passing through lattice point A
Rotate AB to AC and AD by angle q, where q
360/n. Consequently, both C and D are lattice
points. Summation of lattice vector AC and AD
gives AE, when point E should also be a lattice
point, and
4

• 2. Consider the space group Pna21, which belongs
  to the orthorhombic crystal system. Given that
  the position of the symmetry elements along its
  characteristic directions are (1/4, y, z), (x, ¼,
  z), and (0, 0, z), respectively
• Draw the lattice projection along its 001
  crystalline direction, and locate the positions
  of the identical points
• Based on the answer in part (1), draw the map
  containing all the symmetry elements in this
  project

5
½ c
½ c
a
½ c
½ c
½ c
b
6
½ c
½ c
a
½ c
½ c
½ c
b
7
a
b
8
3. What and where is the symmetry element
connecting two points (x, y, z) and (1/2-y,
x-1/4, z1/4)?
9
R is a 4 fold rotational axis along 001
direction, therefore there is a t01/4