Vectors

1
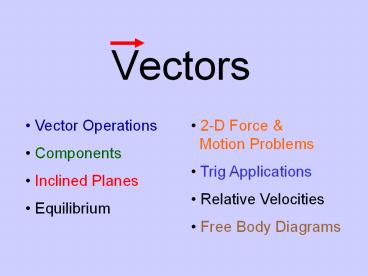
Vectors

• 2-D Force Motion Problems
• Trig Applications
• Relative Velocities
• Free Body Diagrams

• Vector Operations
• Components
• Inclined Planes
• Equilibrium

2
Vector Addition
Suppose 3 forces act on an object at the same
time. Fnet is not 15 N because these forces
arent working together. But theyre not
completely opposing each either. So how do find
Fnet ? The answer is to add the vectors ... not
their magnitudes, but the vectors themselves.
There are two basic ways to add vectors w/
pictures
8 N
4 N
3 N

• Tip to tail method
• Parallelogram method

3
Tip to Tail Methodin-line examples
20 N
12 N
Place the tail of one vector at the tip of the
other. The vector sum (also called the
resultant) is shown in red. It starts where the
black vector began and goes to the tip of the
blue one. In these cases, the vector sum
represents the net force. You can only add or
subtract magnitudes when the vectors are in-line!
16 N
9 N
20 N
16 N
21 N
4 N
9 N
12 N
4
Tip to Tail 2 Vectors
To add the red and blue displacement vectors
first note

• Vectors can only be added if they are of the
  same quantityin this case, displacement.
• The magnitude of the resultant must be less
  than 7 m (5 2 7) and greater than 3 m
  (5 - 2 3).

5 m
2 m
Interpretation Walking 5 m in the direction of
the blue vector and then 2 m in the direction of
the red one is equivalent to walking in the
direction of the black vector. The distance
walked this way is the black vectors magnitude.
Place the vectors tip to tail and draw a vector
from the tail of the first to the tip of the
second.
2 m
5 m
blue red
5
Commutative Property
red blue
blue red
As with scalars quantities and ordinary numbers,
the order of addition is irrelevant with vectors.
Note that the resultant (black vector) is the
same magnitude and direction in each case.
(Well learn how to find the resultants
magnitude soon.)
6
Tip to Tail 3 Vectors
We can add 3 or more vectors by placing them tip
to tail in any order, so long as they are of the
same type (force, velocity, displacement, etc.).
blue green red
7
Parallelogram Method
This time well add red blue by placing the
tails together and drawing a parallelogram with
dotted lines. The resultants tail is at the
same point as the other tails. Its tip is at
the intersection of the dotted lines.
Note Opposite sides of a parallelogram are
congruent.
8
Comparison of Methods
red blue
The resultant has the same magnitude and
direction regardless of the method used.
Tip to tail method
Parallelogram method
9
Opposite of a Vector
If v is 17 m/s up and to the right, then -v
is 17 m/s down and to the left. The directions
are opposite the magnitudes are the same.
v
- v
10
Scalar Multiplication
Scalar multiplication means multiplying a vector
by a real number, such as 8.6. The result is a
parallel vector of a different length. If the
scalar is positive, the direction doesnt change.
If its negative, the direction is exactly
opposite.
x
3x
-2x
Blue is 3 times longer than red in the same
direction. Black is half as long as red. Green
is twice as long as red in the opposite direction.
x
½
11
Vector Subtraction
Put vector tails together and complete the
triangle, pointing to the vector that comes
first in the subtraction.
red - blue
Why it works In the first diagram, blue and
black are tip to tail, so blue black red
? red blue black.
blue - red
Note that red - blue is the opposite of blue -
red.
12
Other Operations

• Vectors are not multiplied, at least not the way
  numbers are, but there are two types of vector
  products that will be explained later.
• Cross product
• Dot product
• These products are different than scalar mult.
• There is no such thing as division of vectors
• Vectors can be divided by scalars.
• Dividing by a scalar is the same as multiplying
  by its reciprocal.

13
Comparison of Vectors
Which vector is bigger?
43 m
15 N
27 m/s
0.056 km
The question of size here doesnt make sense.
Its like asking, Whats bigger, an hour or a
gallon? You can only compare vectors if they
are of the same quantity. Here, reds magnitude
is greater than blues, since 0.056 km 56 m gt
43 m, so red must be drawn longer than blue, but
these are the only two we can compare.
14
Vector Components
A 150 N force is exerted up and to the right.
This force can be thought of as two separate
forces working together, one to the right, and
the other up. These components are perpendicular
to each other. Note that the vector sum of the
components is the original vector (green red
black). The components can also be drawn like
this
Vertical component
150 N
Horizontal component
15
Finding Components with Trig
Multiply the magnitude of the original vector by
the sine cosine of the angle made with the
given. The units of the components are the same
as the units for the original vector.
Heres the correspondence cosine ? adjacent
side sine ? opposite side
v
v sin ?
?
v cos ?
16
Component Example
30.814 m/s
25?
14.369 m/s
34 m/s
A helicopter is flying at 34 m/s at 25? S of W
(south of west). The magnitude of the horizontal
component is 34 cos 25? ? 30.814 m/s. This is
how fast the copter is traveling to the west.
The magnitude of the vertical component is 34 sin
25? ? 14.369 m/s. This is how fast its moving
to the south.
Note that 30.814 14.369 gt 34. Adding up vector
components gives the original vector (green red
black), but adding up the magnitudes of the
components is meaningless.
17
Pythagorean Theorem
30.814 m/s
25?
14.369 m/s
34 m/s
Since components always form a right triangle,
the Pythagorean theorem holds (14.369)2
(30.814)2 (34)2.
Note that a component can be as long, but no
longer than, the vector itself. This is because
the sides of a right triangle cant be longer
than the hypotenuse.
18
Other component pairs
v cos ?
v cos ?
?
?
v cos ?
?
v sin ?
v sin ?
v
v
v
v sin ?
There are an infinite number of component pairs
into which a vector can be split. Note that
green red black in all 3 diagrams, and that
green and red are always perpendicular. The
angle is different in each diagram, as well as
the lengths of the components, but the
Pythagorean theorem holds for each. The pair of
components used depends on the geometry of the
problem.
19
Component Form
Instead of a magnitude and an angle, vectors are
often specified by listing their horizontal and
vertical components. For example, consider this
acceleration vector
a 10 m/s2 at 53.13? N of W
In component form
a ?-3, 4 ? m/s2
4 m/s2
a 10 m/s2
Some books use parentheses rather than angle
brackets. The vector F ?2, -1, 3? N indicates
a force that is a combination of 2 N to the east,
1 N south, and 3 N up. Its magnitude is found w/
the Pythag. theoremF 22 (-1)2 321/2
3.742 N
53.13?
3 m/s2
20
Finding the direction of a vector
x ?5, -2? meters is clearly a position to the
southeast of a given reference point. If the
reference pt. is the origin, then x is in the
4th quadrant. The tangent of the angle relative
to the east is given by
tan ? 2 m / 5 m ? ? tan -1(0.4)
21.801?
The magnitude of x is (25 4)1/2 5.385 m.
Thus, ?5, -2? meters is equivalent to 5.385 m
at 21.801? S of E.
5 m
?
2 m
21
Adding vectors in component form
If F1 ? 3, 7 ? N and
F2 ? 2, -4 ? N, then the
net force is simply given by
Fnet ? 5, 3 ? N. Just add the horizontal and
vertical components separately.
F2
F1
Fnet
22
Inclined Plane
A crate of chop suey of mass m is setting on a
ramp with angle of inclination ?. The weight
vector is straight down. The parallel component
(blue) acts parallel to the ramp and is the
component of the weight pulling the crate down
the ramp. The perpendicular component (red) acts
perpendicular to the ramp and is the component of
the weight that tries to crush the ramp.
m
parallel component
?
Note red blue black
perpendicular component
continued on next slide
mg
23
Inclined Plane (continued)
The diagram contains two right triangles. ? is
the angle between black and blue. ? ? 90?
since they are both angles of the right triangle
on the right. Since blue and red are
perpendicular, the angle between red and black
must also be ?. Imagine the parallel component
sliding down (dotted blue) to form a right
triangle. Being opposite ?, we use sine. Red
is
adjacent to ?, so we use cosine.
m
mg sin ?
?
?
?
mg cos ?
mg
continued on next slide
mg sin ?
24
Inclined Plane (continued)
m
mg sin ?
?
mg cos ?
mg
The diagram does not represent 3 different forces
are acting on the chop suey at the same time.
All 3 acting together at one time would double
the weight, since the components add up to
another weight vector. Either work with mg
alone or work with both components together.
25
How the incline affects the components
m
mg sin ?
m
mg sin ?
mg cos ?
mg cos ?
mg
mg
The steeper the incline, the greater ? is, and
the greater sin ? is. Thus, a steep incline
means a large parallel component and a small
horizontal one. Conversely, a gradual incline
means a large horizontal component and a small
vertical one.
Extreme cases When ? 0, the ramp is flat red
mg blue 0.When ? 90?, the ramp is
vertical red 0 blue mg.
26
Inclined Plane - Pythagorean Theorem
m
mg sin?
?
Lets show that the Pythagorean theorem holds for
components on the inclined plane
mg cos?
mg
(mg sin? )2 (mg cos? )2 (mg)2 (sin2? cos2?
) (mg)2 (1) (mg)2
27
Inclined Plane Normal Force
Recall normal force is perpen-dicular to the
contact surface. As long as the ramp itself
isnt accelerating and no other forces are
lifting the box off the ramp or pushing it into
the ramp, N matches the perpendicular component
of the weight. This must be the case, otherwise
the box would be accelerating in the direction of
red or green. N gt mg cos? would mean the box is
jumping off the ramp. N lt mg cos? would mean
that the ramp is being crushed.
N mg cos?
mg sin?
m
?
mg cos?
mg
28
Net Force on a Frictionless Inclined Plane
With no friction, Fnet mg N mg cos?
mg sin? N mg sin?. (mg cos? N 0
since their magnitudes are equal but theyre in
directions opposite. That is, the perpendicular
component of the weight and the normal cancel
out.)
N mg cos?
mg sin?
m
?
mg cos?
Therefore, the net force is the parallel force in
this case.
mg
29
Acceleration on a Frictionless Ramp
Here Fnet mg sin? m a. So, a g sin?.
Since sin? has no units, a has the same units
as g, as they should. Both the net force and
the acceleration are down the ramp.
m
mg sin?
?
mg cos?
mg
30
Incline with friction at equilibrium
At equilibrium Fnet 0, so all forces must
cancel out. Here, the normal force cancels the
perpendicular component of the weight, and the
static frictional force cancels the parallel
component of the weight.
N mg cos?
fs mg sin?
m
mg sin?
?
mg cos?
mg
continued on next slide
31
Incline with friction at equilibrium (cont.)
fs ? ?s N ?s mg cos?. Also,fs mg sin?
(only because we have equilibrium). So, mg sin?
? ?s mg cos ?.Since the mgs cancel and tan ?
sin? / cos?, we have ?s ? tan?.
N mg cos?
fs mg sin?
m
mg sin?
?
mg cos?
mg
continued on next slide
32
Incline with friction at equilibrium (cont.)
Suppose we slowly crank up the angle, gradually
making the ramp steeper and steeper, until the
box is just about to budge. At this angle, fs
fs, max ?s N ?s mg cos ?. So now we havemg
sin? ?s mg cos?, and ?s tan?.
N mg cos?
fs mg sin?

mg sin?
?
(Neither of these quantities have units.)
mg cos?
An adjustable ramp is a convenient way to find
the coefficient of static friction between two
materials.
mg
33
Acceleration on a ramp with friction
In order for the box to budge, mg sin? must be
greater than fs, max which means tan? must be
greater than ?s. If this is the case, forget
about ?s and use ?k. fk ?kN ?k mg cos?.
Fnet mg sin? - fk ma. So, mg sin? - ?k mg
cos? ma. The ms cancel, which means a is
independent of the size of the box. Solving for
a we get a g sin? - ?k g cos?. Once again,
the units work out right.
N mg cos?
fk ?k mg cos?

mg sin?
?
mg cos?
mg
34
Parallel applied force on ramp
In this case FA and mg sin? are working
together against friction. Assuming FA mg
sin? gt fs, max the box budges and the 2nd Law
tells us FA mg sin ? - fk ma.Mass does not
cancel out this time.
N
fk

FA
mg sin?
?
If FA were directed up the ramp, wed have
acceleration up or down the ramp depending on the
size of FA compared to mg sin?. If FA were
bigger, friction acts down the ramp and a is up
the ramp.
mg
mg cos?
35
Non-parallel applied force on ramp
Suppose the applied force acts on the box, at an
angle ? above the horizontal, rather than
parallel to the ramp. We must resolve FA into
parallel and perpendicular components (orange and
gray) using the angle ? ?. FA serves to
increase acceleration directly and indirectly
directly by orange pulling the box down the ramp,
and indirectly by gray lightening the contact
force with the ramp (thereby reducing friction).
FA sin(? ? )
FA
N
fk
?

?
FA cos(? ? )
mg sin?
?
mg
mg cos?
continued on next slide
36
Non-parallel applied force on ramp (cont.)
FA sin (? ?)
Because of the perp. comp. of FA, N lt mg cos?.
Assuming FA sin(? ? ) is not big enough to
lift the box off the ramp, there is no
acceleration in the perpendicular direction. So,
FA sin(? ? ) N mg cos?. Remember, N is
what a scale would read if placed under the box,
and a scale reads less if a force lifts up on the
box. So, N mg cos? - FA sin(? ? ), which
means fk ?k N ?k mg cos? - FA sin(? ?
).
FA
N
fk
?

?
FA cos(? ? )
mg sin?
?
mg cos?
mg
continued on next slide
37
Non-parallel applied force on ramp (cont.)
Assuming the combined force of orange and blue
is enough to budge the box, we have Fnet
orange blue - brown ma.
FA sin(? ? )
FA
N
fk
?

?
FA cos(? ? )
mg sin?
?
Substituting, we haveFA cos(? ? ) mg sin?
- ?k mg cos? - FA sin(? ? ) ma.
mg cos?
mg
38
Hanging Sign Problem
The Hanging Sign Problem
continued on next slide
39
Hanging sign f.b.d.
Free Body Diagram
Since the sign is not accelerating in any
direction, its in equilibrium. Since its not
moving either, we call it Static Equilibrium.
Thus, red green black 0.
continued on next slide
40
Hanging sign force triangle
Fnet 0 means a closed vector polygon !
As long as Fnet 0, this is true no matter many
forces are involved.
Vector Equation
T1 T2 mg 0
continued on next slide
41
Hanging sign equations
Components Scalar Equations
We use Newtons 2nd Law twice, once in each
dimension
42
Hanging sign sample
Sample Problem
Answers
T1 347.65 N T2 606.60 N
Accurately draw all vectors and find T1 T2.
43
Vector Force Lab Simulation
Go to the link below. This is not exactly the
same as the hanging sign problem, but it is
static equilibrium with three forces.
Equilibrium link

1. Change the strengths of the three forces (left,
   right, and below) to any values you choose. (The
   program wont allow a change that is physically
   impossible.)
2. Record the angles that are displayed below the
   forces. They are measured from the vertical.
3. Using the angles given and the blue and red
   tensions, do the math to prove that the computer
   program really is displaying a system in
   equilibrium.
4. Now click on the Parallelogram of Forces box and
   write a clear explanation of what is being
   displayed and why.

44
3 - Way Tug-o-War
Bugs Bunny, Yosemite Sam, and the Tweety Bird
are fighting over a giant 450 g Acme super ball.
If their forces remain constant, how far, and in
what direction, will the ball move in 3 s,
assuming the super ball is initially at rest ?
Sam 111 N
Tweety 64 N
38
43
Bugs 95 N
To answer this question, we must find a, so we
can do kinematics. But in order to find a, we
must first find Fnet.
continued on next slide
45
3 - Way Tug-o-War (continued)
Sam 111 N
First, all vectors are split into horiz. vert.
comps. Sams are purple, Tweetys orange. Bugs
is already done since hes purely vertical. The
vector sum of all components is the same as the
sum of the original three vectors. Avoid much
rounding until the end.
continued on next slide
46
3 - Way Tug-o-War (continued)
Next we combine all parallel vectors by adding or
subtracting 68.3384 43.6479 - 95
16.9863, and 87.4692 - 46.8066
40.6626. A new picture shows the net vertical
and horizontal forces on the super ball.
Interpretation Sam Tweety together slightly
overpower Bugs vertically by about 17 N. But Sam
Tweety oppose each other horizontally, where
Sam overpowers Tweety by about 41 N.
16.9863 N
40.6626 N
continued on next slide
47
3 - Way Tug-o-War (continued)
Fnet 44.0679 N
16.9863 N
?
40.6626 N
Find Fnet using the Pythagorean theorem. Find
? using trig tan? 16.9863 N / 40.6626 N. The
newtons cancel out, so ? tan-1(16.9863 /
40.6626) 22.6689?. (tan-1 is the same as
arctan.) Therefore, the superball experiences a
net force of about 44 N in the direction of about
23? north of west. This is the combined effect
of all three cartoon characters.
continued on next slide
48
3 - Way Tug-o-War (final)
a Fnet / m 44.0679 N / 0.45 kg 97.9287
m/s2. Note the conversion from grams to
kilograms, which is necessary since 1 m/s2 1 N
/ kg. As always, a is in the same direction as
Fnet.. a is constant for the full 3 s, since
the forces are constant.
Now its kinematics time Using the fact?x v0
t 0.5 a t 2 0 0.5 (97.9287)(3)2
440.6792 m ? 441 m,rounding at the end.
97.9287 m/s2
22.6689?
So the super ball will move about 441 m at about
23? N of W. To find out how far north or west,
use trig and find the components of the
displacement vector.
49
3 - Way Tug-o-War Practice Problem
The 3 Stooges are fighting over a 10 000 g (10
thousand gram) Snickers Bar. The fight lasts 9.6
s, and their forces are constant. The floor on
which theyre standing has a huge coordinate
system painted on it, and the candy bar is at the
origin. What are its final coordinates?
Answer
Hint Find this angle first.
Curly 1000 N
( -203.66 , 2246.22 ) in meters
Larry 150 N
78?
93?
Moe 500 N
50
How to budge a stubborn mule
It would be pretty tough to budge this mule by
pulling directly on his collar. But it would be
relatively easy to budge him using this set-up.
(explanation on next slide)
Big Force
Little Force
51
How to budge a stubborn mule (cont.)
little force
tree
mule
overhead view
Just before the mule budges, we have static
equilibrium. This means the tension forces in
the rope segments must cancel out the little
applied force. But because of the small angle,
the tension is huge, enough to budge the mule!
(more explanation on next slide)
little force
T
T
tree
mule
52
How to budge a stubborn mule (final)
Because ? is so small, the tensions must be
large to have vertical components (orange) big
enough to team up and cancel the little force.
Since the tension is the same throughout the
rope, the big tension forces shown acting at the
middle are the same as the forces acting on the
tree and mule. So the mule is pulled in the
direction of the rope with a force equal to the
tension. This set-up magnifies your force
greatly.
little force
?
?
T
T
tree
mule
53
Relative Velocities in 1 D
Schmedrick and his dog, Rover, are goofing around
on a train. Schmed can throw a fast ball at 23
m/s. Rover can run at 9 m/s. The train goes 15
m/s.
Question 1 If Rover is sitting beside the
tracks with a radar gun as the train goes by, and
Schmedrick is on the train throwing a fastball in
the direction of the train, how fast does Rover
clock the ball? vBT velocity of the ball with
respect to the train 23 m/svTG velocity of
the train with respect to the ground 15 m/svBG
velocity of the ball with respect to ground
38 m/s This is a simple example, but in general,
to get the answer we add vectors vBG vBT
vTG (In this case we can simply add magnitudes
since the vectors are parallel.)
continued on next slide
54
Relative Velocities in 1 D (cont.)
vBG vBT vTG

• Velocities are not absolute they depend on the
  motion of the person who is doing the
  measuring.
• Write a vector sum so that the inner subscripts
  match.
• The outer subscripts give the subscripts for the
  resultant.
• This trick works even when vectors dont line
  up.
• Vector diagrams help (especially when we move to
  2-D).

vBT 23 m/s
vTG 15 m/s
vBG 38 m/s
continued on next slide
55
Relative Velocities in 1 D (cont.)
Question 2 Lets choose the positive direction
to be to the right. If Schmedrick is standing
still on the ground and Rover is running to the
right, then the velocity of Rover with respect to
Schmedrick vRS 9 m/s. From Rovers
perspective, though, he is the one who is still
and Schmedrick (and the rest of the landscape) is
moving to the left at 9 m/s. This means the
velocity of Schmedrick with respect to Rover
vSR -9 m/s. Therefore, vRS - vSR The
moral of the story is that you get the opposite
of a vector if you reverse the subscripts.
vRS
vSR
continued on next slide
56
Relative Velocities in 1 D (cont.)
Question 3 If Rover is chasing the train as
Schmed goes by throwing a fastball, at what speed
does Rover clock the ball now?
Note, because Rover is chasing the train, he will
measure a slower speed. (In fact, if Rover could
run at 38 m/s hed say the fastball is at rest.)
This time we need the velocity of the ball with
respect to Rover vBR vBT vTG vGR vBT
vTG - vRG 23 15 - 9 29
m/s. Note how the inner subscripts match up again
and the outer most give the subscripts of the
resultant. Also, we make use of the fact that
vGR - vRG.
vBT 23 m/s
vTG 15 m/s
vBG 29 m/s
vRG 9 m/s
57
River Crossing
campsite
0.3 m/s
Current
river
boat
Youre directly across a 20 m wide river from
your buddies campsite. Your only means of
crossing is your trusty rowboat, which you can
row at 0.5 m/s in still water. If you aim your
boat directly at the camp, youll end up to the
right of it because of the current. At what
angle should you row in order to trying to land
right at the campsite, and how long will it take
you to get there?
continued on next slide
58
River Crossing (cont.)
campsite
0.3 m/s
0.3 m/s
Current
?
0.4 m/s
0.5 m/s
river
boat
Because of the current, your boat points in the
direction of red but moves in the direction of
green. The Pythagorean theorem tells us that
greens magnitude is 0.4 m/s. This is the speed
youre moving with respect to the campsite.
Thus, t d / v (20 m) / (0.4 m/s) 50 s. ?
tan-1(0.3 / 0.4) ? 36.9?.
continued on next slide
59
River Crossing Relative Velocities
The red vector is the velocity of the boat with
respect to the water, vBW, which is what your
speedometer would read.Blue is the velocity of
the water w/ resp. to the camp, vWC. Green is
the velocity of the boat with respect to the
camp, vBC.
The only thing that could vary in our problem was
?. It had to be determined so that red blue
gave a vector pointing directly across the river,
which is the way you wanted to go.
continued on next slide
campsite
0.3 m/s
0.3 m/s
Current
?
0.4 m/s
0.5 m/s
river
60
River Crossing Relative Velocities (cont.)
vWC
vBW vel. of boat w/ respect to water vWC
vel. of water w/ respect to camp vBC vel. of
boat w/ respect to camp
vBW
vBC
?
Look how they add up
vBW vWC vBC
The inner subscripts match the out ones give
subscripts of the resultant. This technique
works in 1, 2, or 3 dimensions w/ any number or
vectors.
61
Law of Sines
The river problem involved a right triangle. If
it hadnt we would have had to use either
component techniques or the two laws youll also
do in trig class Law of Sines Law of
Cosines.
sin A
sin B
sin C
Law of Sines


a
c
b
Side a is opposite angle A, b is opposite B,
and c is opposite C.
62
Law of Cosines
C
b
a
c
A
B
a 2 b 2 c 2 - 2 b c cosA
Law of Cosines
These two sides are repeated.
This side is always opposite this angle.
It doesnt matter which side is called a, b, and
c, so long as the two rules above are followed.
This law is like the Pythagorean theorem with a
built in correction term of -2 b c cos A. This
term allows us to work with non-right triangles.
Note if A 90?, this term drops out (cos 90?
0), and we have the normal Pythagorean theorem.
63
Wonder Woman Jet Problem
Suppose Wonder Woman is flying her invisible jet.
Her onboard controls display a velocity of 304
mph 10? E of N. A wind blows at 195 mph in the
direction of 32? N of E. What is her velocity
with respect to Aqua Man, who is resting poolside
down on the ground?
vWA vel. of Wonder Woman w/ resp. to the
air vAG vel. of the air w/ resp. to the ground
(and Aqua Man) vWG vel. of Wonder Woman w/
resp. to the ground (Aqua Man)
We know the first two vectors we need to find
the third. First well find it using the laws of
sines cosines, then well check the result
using components. Either way, we need to make a
vector diagram.
continued on next slide
64
Wonder Woman Jet Problem (cont.)
vAG
195 mph
32?
32?
80?
100?
vWG
vWG
vWA
304 mph
10?
vWA vAG vWG
80?
The 80? angle at the lower right is the
complement of the 10? angle. The two 80? angles
are alternate interior. The 100? angle is the
supplement of the 80? angle. Now we know the
angle between red and blue is 132?.
continued on next slide
65
Wonder Woman Jet Problem (cont.)
By the law of cosines v 2 (304)2 (195)2 - 2
(304) (195) cos 132?. So, v 458 mph. Note that
the last term above appears negative, but its
actually positive, since cos 132? lt 0. The law
of sines says
sin 132?
sin?

v
195 mph
195
So, sin? 195 sin 132? / 458, and ? ? 18.45?
132?
v
This mean the angle between green and the
horizontal is 80? - 18.45? ? 61.6?
304 mph
Therefore, from Aqua Mans perspective, Wonder
Woman is flying at 458 mph at 61.6? N of E.
?
80?
66
Wonder Woman Problem Component Method
This time well add vectors via components as
weve done before. Note that because of the
angles given here, we use cosine for the vertical
comp. of red but sine for the vertical comp. of
blue. All units are mph.
vAG 195 mph
195
103.3343
32?
165.3694
299.3816
304
vWA 304 mph
10?
52.789
continued on next slide
67
Wonder Woman Component Method (cont.)
Combine vertical horiz. comps. separately and
use Pythag. theorem. ? tan-1(218.1584 /
402.7159) 28.4452?. ? is measured from the
vertical, which is why its 10? more than ?.
218.1584 mph
52.789
165.3694
195
103.3343
103.3343
165.3694
402.7159 mph
458.0100 mph
299.3816
299.3816
304
?
52.789
68
Comparison of Methods

• We ended up with same result for Wonder Woman
  doing it in two different ways. Each way
  requires some work. You will only want to use
  the laws of sines cosines if
• the vectors form a triangle.
• youre dealing with exactly 3 vectors.
  (If youre adding 3 vectors, the resultant makes
  a total of 4, and this method would require
  using 2 separate triangles.)
• Regardless of the method, draw a vector diagram!
  To determine which two vectors add to the third,
  use the subscript trick.

69
Free body diagrams 1
For the next several slides, draw a free body
diagram for each mass in the set-up and find a
(or write a system of 2nd Law
equations from which you could
find a.)
F2
v
F1
m
floor
Two applied forces F2 lt mg coef. of kinetic
friction ?k
answer
70
Free body diagrams 2
Bodies start at rest m3 gt m1 m2 frictionless
pulley with negligible mass. answer
71
Free body diagrams 3
answer
v
m1 gt m3
m2
?k
m1
m3
72
Free body diagrams 4
answer
m
v
Rock falling down in a pool of water
73
Free body diagrams 5
answer
A large crate of cotton candy and a small iron
block of the same mass are falling in air at the
same speed, accelerating down.
cotton candy
Fe
74
Free body diagrams 6 a
answer
The boxes are not slidingcoefficients of
static friction are given.
There is no friction acting on m2.
It would not be in equilibrium otherwise. T
m3 g f1 ? ?1 N1 ?1(m1 m2) g f1s reaction
pair acting on table is not shown.
?2 is extraneous info in this problem, but not
in the next slide.
N1
T
T
m1
f1
N2
m3
m2 g
m2
m1 g
m2 g
m3 g
75
Free body diagrams 6 b
Boxes accelerating (clockwise) m1 m2 are
sliding coefs of kinetic friction given.
answer
v
76
Free body diagrams 7
Boxes moving clockwise at constant speed.
answer
v
m2
?k
?
77
Free body diagrams 8
Mr. Stickman is out for a walk. Hes moseying
along but picking up speed with each step. The
coef. of static friction between the grass and
his stick sneakers is ?s.
answer
Heres a case where friction is a good thing.
Without it we couldnt walk. (Its difficult to
walk on ice since ?s is so small.) We use fs
here since we assume hes not slipping. Note
friction is in the direction of motion in this
case. His pushing force does not appear in the
free body diag. since it acts on the ground, not
him. The reaction to his push is friction.
Fnet fs So, ma fs ? fs, max ?s m g Thus,
a ? ?s g.
N
v
fs
mg
78
Free body diagrams 9
answer
F
?
m
v
ground
?k
79
Dot Products
First recall vector addition in component form
Its just component-wise addition. Note that the
sum of two vectors is a vector.
For a dot product we do component-wise
multiplication and add up the results
Note that the dot product of two vectors is a
scalar!
Dot products are used to find the work done by a
force applied over a distance, as well see in
the future.
80
Dot Product Properties

• The dot product of two vectors is a scalar.
• It can be proven that a ? b a b cos?, where
  ? is the angle between a and b.
• The dot product of perpendicular vectors is
  zero.
• The dot product of parallel vectors is simply
  the product of their magnitudes.
• A dot product is commutative
• A dot product can be performed on two vectors of
  the same dimension, no matter how big the
  dimension.

81
Unit Vectors in 2-D
Any vector can be written as the sum of its
components.
The vector v ? -3, 4 ? indicates 3 units left
and 4 units up, which is the sum of its
components v ? -3, 4 ? ?
-3, 0 ? ? 0, 4 ?
Lets factor out what we can from each vector in
the sum v ? -3, 4 ? -3 ?
1, 0 ? 4 ? 0, 1 ? The vectors on the right
side are each of magnitude one. For this reason
they are called unit vectors.
A shorthand for the unit vector ? 1, 0 ? is i.
A shorthand for the unit vector ? 0, 1 ? is j.
Thus, v ? -3, 4 ? -3 i 4 j
82
Unit Vectors in 3-D
One way to interpret the vector v ? 7, -5, 9 ?
is that it indicates 7 units east, 5 units south,
and 9 units up. v can be written as the sum
components as follows
v ? 7, -5, 9 ? ? 7, 0, 0 ? ? 0, -5, 0 ? ?
0, 0, 9 ?
7 ? 1, 0, 0 ? - 5 ? 0, 1, 0 ? 9 ? 0, 0, 1 ?
7 i - 5 j 9 k
In 3-D we define these unit vectorsi ? 1, 0,
0 ?, j ? 0, 1, 0 ?, and k ? 0, 0, 1 ?
(continued on next slide)
83
Unit Vectors in 3-D (cont.)
z
P
1
k
j
y
1
1
i
The x-, y-, and z-axes are mutually
perpendicular, as are i, j, and k. The yellow
plane is the x-y plane. i and j are in this
plane. Any point in space
x
can be reached from the origin using a linear
combination of these 3 unit vectors. Ex P
(-1.8, -1.4, 1.2) so the vector
-1.8 i 1.4 j 1.2 k will extend from the
origin to P.
84
Determinants
To take a determinant of a 2 ? 2 matrix,
multiply diagonals and subtract. The determinant
of A is written A and it equals 3 (11) -
4 (-2) 33 8 41.
In order to do cross products we will need to
find determinants of 3 ? 3 matrices. One way to
do this is to expand about the 1st row using
minors, which are smaller determinants within a
determinant. To find the minor of an element,
cross out its row and column and keep what
remains.
Minor of a
Minor of b
Minor of c
cont. on next slide
85
Determinants (cont.)
By definition,
(Minor of b) c
a
(Minor of a) - b
(Minor of c )
- b
a
c
a (e i - h f ) - b (d i - g f ) c (d h -
g e)
Determinants can be expanded about any row or
column. Besides cross products, determinants have
many other purposes, such as solving systems of
linear equations.
86
Cross Products
Let v1 ? x1, y1, z1 ?
and v2 ? x2, y2, z2 ?.
By definition, the cross product of these
vectors (pronounced v1 cross v2) is given by
the following determinant.
(y1 z2 - y2 z1) i - (x1 z2 - x2 z1) j (x1
y2 - x2 y1) k
Note that the cross product of two vectors is
another vector!Cross products are used a lot in
physics, e.g., torque is a vector defined as the
cross product of a displacement vector and a
force vector. Well learn about torque in another
unit.
87
Right hand rule
A quick way to determine the direction of a cross
product is to use the right hand rule. To find a
? b, place the knife edge of your right hand
(pinky side) along a and curl your hand toward
b, making a fist. Your thumb then points in the
direction of
a ? b.
a ? b
It can be proven that the magnitude of
a ? b
is given by
a b sin?
b
a ? b
?
where ? is the angle between a and b.
a
88
Dot Product vs. Cross Product
1. The dot product of two vectors is a scalar
the cross product is another vector
(perpendicular to each of the original). 2. A
dot product is commutative a cross product is
not. In fact,
a ? b
- b ? a.
3. Dot product definition
i j k
Cross product definition
v1 ? v2
x1 y1 z1
x2 y2 z2
a b sin?
a ? b
4. a ? b a b cos?, and