Quantitaive Genetics

1

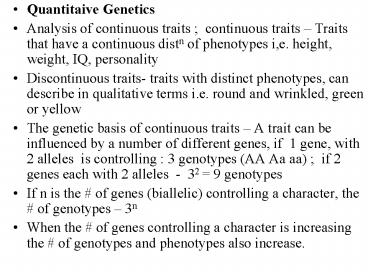
• Quantitaive Genetics
• Analysis of continuous traits continuous
  traits Traits that have a continuous distn of
  phenotypes i,e. height, weight, IQ, personality
• Discontinuous traits- traits with distinct
  phenotypes, can describe in qualitative terms
  i.e. round and wrinkled, green or yellow
• The genetic basis of continuous traits A trait
  can be influenced by a number of different genes,
  if 1 gene, with 2 alleles is controlling 3
  genotypes (AA Aa aa) if 2 genes each with 2
  alleles - 32 9 genotypes
• If n is the of genes (biallelic) controlling a
  character, the of genotypes 3n
• When the of genes controlling a character is
  increasing the of genotypes and phenotypes also
  increase.

2

• of Genes of Genotypes
• 1 3
• 2 9
• 5 243
• 10 59,049

3

• Multiple factor hypothesis- Many factors or genes
  contribute to a phenotype in a cumulative way.
• Two or more pairs of genes account for the
  hereditary influence on the phenotype in an
  additive way, I.e AABB
• Corolla length of Nicotiana , Fig. 6-1
• Each gene locus may be occupied by either an
  additive allele (homozygous form, AABBCC) which
  contributes a set amount to the phenotype or by a
  non additive allele which does not contribute
  quantitatively to the phenotype AaBb

4

• Analyses of polygenic traits When a large
  sample is picked from a population of individuals
  showing a specific quantitative character, the
  values follow a normal distribution. To describe
  a distribution we need certain descriptors and
  the most basic descriptors of a distn are its
  Mean and Variance

5
(No Transcript)
6

• Mean The arithmetic average of the set of
  measurements
• Mean S xi/n of if the individuals are
  classified into classes, Mean S fi xi/ S fi
• Varaince- The degree to which the values within
  the distribution diverge from the mean is given
  by
• s2 S (xi-x)2/n-1,
• S xi 2- n x2/ n-1 (when individuals are
  classified into frequency classes n S fi
  (xi 2)- (S fi (xi)2/ n(n-1))
• Standard deviation, s ?s2 expresses variation
  around the mean in the original units, the ( x
  1s) includes 68 of all values in the sample
• Standard error of the mean, S x s/?n
  estimates how much the means of other similar
  samples drawn from the same popn might vary.
• Fig.6.2,

7

• In polygenic inheritence, although many genes are
  involved each gene follow Mendelian inheritance.
• Crossing of two contrasting populations of
  Nicotiana longiflora (tobacco) fig. 6.2
• The F1, F2 and selected F3 data demonstrated a
  distinct pattern.
• Points to note F1 was intermediate minor
  variability among individuals, F2 larger
  variation the majority resembled F1 but fewer
  plants of extreme phenotypes. Selected F2,
  selfed and obtained a sample (F3) for each F2 .
• Wheat grain color experiment
• Red grain x white grain shows that the additive
  alleles influence the phenotype in a quantitative
  manner - multiple factor inheritance fig 6.3

8

• Variation of fruit weight in F1 and F2
• weight
  (oz)
• x 6 7 8 9 10 11 12 13
  14 15 16 17 18 S
• in F1 4 14 16 12
  6 52
• in F2 1 1 2 0 9 13 17 14
  7 4 3 0 1 72
• F1 f(x) 40 154 192 156
  84 626
• fx2 400 1694
  2304 2028 1176 7602
• For F1 s2 52 x 7602 (626)2
  for F2 s2 4.27
• 52
  (52-1) mean 12.11
• 1.29
• mean 12.04
• The means are almost same for F1 and F2 but a
  larger variance in F2

9

• Additive, dominance, and epistatic effects can
  all contribute to the phenotype of a quantitative
  trait, but generally additive interactions are
  the most important.
• All of the above factors are genetic in nature,
  but the environment also affects quantitative
  traits. The primary affect of the environment is
  to change the value for a particular genotype.
  This variation would be the result of the
  different environments in which the genotype was
  grown or reared. The consequence of this
  environmental effect is that the distribution
  even more resembles a normal distribution

10

• Calculating the of genes- If the ratio of F2
  individuals resembling either of the two most
  extreme phenotypes can be determined then the
  of gene pairs involved is 1/4n i.e. When in
  dihybrid cross the of extreme phenotypes are
  1/16 1/42 n2 AABB or aabb
• Fig 6.4

11

• Heritability- Defined as the proportion of
  variation attributable to genetic factors to the
  total phenotypic variation.
• Phenotype Genotype environment genotype x
  environment
• We would need to know the proportion of heritable
  variation for traits which are important such as
  milk prodn in cattle, eggs laid by hens, blood
  pressure in humans
• The components of phenotypic variation-
• To estimate the genetic component of a trait we
  must first know the total phenotypic varn of the
  popn.
• Vp Vg Ve Vge H2b Vg/Vp (broad sense
  heritability)

12

• Note heritability is specific for a trait and
  can only be measured for a popn, and is valid
  only for the situation that it was measured,
  since the environmental variation changes from
  situation to situation H2 varies from 0 to 1
• Heritability does not indicate the degree
  to which a trait is genetic, it measures the
  proportion of the phenotypic variance that is the
  result of genetic factors

13

• In estimating broad sense H, we only consider the
  amount of variation due to genetic effects as a
  whole, but ignores the actual additive,
  dominance and gene interaction effects making
  its usefulness limited.
• Narrow sense heritability measures the
  proportion of phenotypic variance that is due to
  additive genetic variance (VA). So h2 VA/VP
• VG VA VD h2 VA/ VE VA VD
• h2, values are useful in predicting the
  phenotypes of offspring during selection
  procedures. When the value is closer to 1.0 the
  greater is our ability to make predictions.

14

• The process of selecting a specific group of
  organisms from a heterogenous popn for future
  breeding purposes is called artificial selection
• Artificial selection select a segment of
  parental popn with mean (Ts) that expresses the
  most desirable quantitative phenotypes out of the
  base population mean (T) , and interbreeding the
  selected individuals yield offspring of mean (T)
  
• Selection differential (s) Ts T
• ( T-T ) is defined as response
• response to selection R h2 s
• h2 R/S

15
(No Transcript)
16

• A base sunflower population has a mean of 100
  days to flowering. Two parents were selected that
  had a mean of 90 days to flowering. The
  quantitative trait days to flowering has a
  heritability of 0.2. What would be the mean of a
  population derived from crossing these two
  parents.
• R h2SR 0.2(90 - 100) days to floweringR
  -2 days
• The new population mean would therefore be 98
  days to flowering (100 days - 2 days).

17

• Estimates of heritability for traits in different
  organisms
• Trait Heritability
• Mice - tail length 60 , Body weight 37 ,
  litter size 15
• Chickens body weight 50 , egg prodn 20,
  egg hatchability 15
• In general H2 is low for traits essential for
  survival - traits that are less critical to
  survival show higher H2
• Twin studies in humans- human twins are very
  useful for studying the heredity versus
  environment problems. Monozygotic or identical
  twins are identical in genetic composition.

18

• But raised in different environments ,differences
  can be studied for certain traits. Characters
  that remain similar in different environments
  have a strong genetic component.
• Mapping quantitative trait loci- locating or
  mapping genes responsible for a quantitative
  trait, localize genes on a particular chromosome
  or chromosomes-
• Ex. Phenotypic trait in Drosophila, resistance
  to insecticide DDT
• Strains resistant to DDT strains sensitive to
  DDT cross to strains identified having dominant
  markers for each chromosome ( 2n 8)
• larger number of offspring produced containing
  different combinations of marker chromosomes and
  chromosomes from either resistant or sensitive
  strains

19

• The markers used in this experiments are called
  RFLP (restriction fragment length
  polymorphisms) they represent specific nuclease
  clevage sites.
• RLFP maps are available for a variety of
  organisms- when both the marker and the
  phenotypic trait are expressed together
  (cosegregate) in several generations it indicates
  that the two are linked- can estimate the map
  lengths and locate the gene relative to the
  marker.
• RFLP analysis has resulted in extensive mapping
  of QTL s in Tomato many loci have been
  identified responsible for fruit wt. Soluable
  solid content and acidity.
• 28 QTLs responible for variation in fruit wt have
  been identified. Out of these, two distinct
  alleles of fw 2.2 has been isolated and used in
  genetic transformation increase and decrease
  fruit wt

20

• Population genetics
• The discipline within evolutionary biology that
  studies changes in allele frequencies is known as
  popn genetics
• Rather than studying the inheritance of a trait,
  population genetics attempts to describe how the
  frequency of the alleles which control the trait
  change over time. To study frequency changes, we
  analyze populations rather than individuals
• Populations a group of individuals from the
  same species that lives in the same geographical
  area and most often interbreeds.
• Gene pool- consists of all gametes made by all
  the breeding members of a popn in a single
  generation

21

• For a population of individuals to succeed over
  evolutionary time, it must contain genetic
  variability. Because we do not know all the
  genetic variables that would predict evolutionary
  success, we study the variability of different
  phenotypes and genotypes to provide an overview
  of the population
• Narrowing the genetic variability is an
  indication of a population becoming extinct since
  they might not have enough phenotypes to resist
  the changing environmental conditions.
• One method of expressing variability is by
  analyzing the genetic data and expressing the
  data in terms of gene (or allelic) frequencies.

22

• The proportion of gametes in a gene pool that
  carry a particular allele represents the
  frequency with which this allele occurs in the
  popn
• Calculating allele frequencies to do this,
  genotypes of a large number of individuals have
  to be determined can infer genotpye by
  phenotype or by analyzing proteins or DNA
  sequences.
• Determining allele frequencies of popns at CCR5
  gene influence an individuals susceptibility to
  infection by HIV. It has been found that the
  mutant genotype ?32/ ?32 was uninfected (yet
  exposed) . Researchers wanted to find out which
  human popns have this allele and how common it
  is?

23

• 100 French individuals from Brittany, France was
  surveyed using direct DNA analyses
• Normal allele CCR5-1
• individuals genotype ?32 alleles
  -1 alleles
• 79 1/1
  158
• 20 1/ ?32 20
  20
• 01 ?32 / ?32 02
• total individuals 100 total alleles 200
  
• frequency of CCR5-1 allele 178/200 0.89
  89
• ?32
  22/200 0.11 11

24

• ?32 allele frequency was studied for 18 European
  popns
• Northern European populations had the highest
  frequency and the, frequency declines to the
  south and to the west, popns without European
  ancestry the allele was absent
• By convention one of the alleles is given the
  designation P and the other q. Thus for the data
  we presented above, p0.89 and q0.11. Because we
  are analyzing all the alleles, the frequencies
  should sum to 1.0 and p q 1. Furthermore, a
  population is considered to be polymorphic if two
  alleles are segregating and if the frequency of
  the most frequent allele is less than 0.99.

25

• Hardy Weinberg Law- They queried why doesnt the
  dominant alleles increase in popns? Why is there
  a balance among all types of genoptyes? To answer
  these queries Hardy (British mathematician) and
  Weinberg ( German physician) independently
  proposed a mathematical model which showed that
  genotype and allele frequencies remain constant
  in popns through time given some assumptions.
• Assumptions Popns are infinitely large no
  selection- all genotypes have equal rate of
  survival and reproductive success no mutation
  individuals do not migrate to or out of a popn
  individuals mate randomly

26

• But in a natural pop these forces interact and
  stays in an equilibrium state I.e. Hardy-Weinberg
  equilibrium
• Important point HW law identifies the real world
  forces that cause allele frequencies to change
• If p equals the frequency of allele A and q is
  the frequency of allele a, union of gametes would
  occur as follows
• P q
• P p2 pq AA genotype freq. p2
• q pq q2 aa genotype freq.
  q2
• Aa
  2pq
• p f(AA) ½f(Aa) (substitute from the above
  table)p p2 ½(2pq) (factor out p and divide)

27

• Demonstration of Hardy-Weinberg law-
• Imagine a popn in which the freq. Of allele A in
  both sperm and eggs is 0.7 and a is 0.3. If
  individuals mate randomly for any one zygote the
  probability that both egg and sperm contain A, is
  0.7 x 0.7 0.49 f (AA)
• Likewise the prob. Of Aa is 0.7 x 0.3 0.21 or
  aA 0.21and the prob. Of aa is 0.3 x 0.3
  0.09 therefore the frequency of Aa is 0.42
• As a check 0.49 0.42 0.09 1
• What will be the frequency distribution of
  alleles in the next generation?
• According to HW law the allele freq. Should be
  constant provided the assumptions work

28

• The AA individuals constitute 49 - we can
  predict that the gametes they produce will
  constitute 49 of the gene pool, these gametes
  will carry allele A
• Aa individuals will form 42 of the popn, so
  their gametes will constitute 42 of the new gene
  pool, half of these gametes would carry A
• So the freq. Of allele A in the new gene pool is
  0.49 0.5x0.42 0.7
• Similarly allele a in the new gene pool will be
  0.5 x 0.42 0.090.3

29

• We can now generalize the case Imagine a gene
  pool in which the freq. Of allele A is p and
  allele a is q such that pq1
• The genotype AA will have a freq. Of p x p p2
  
• Since the prob. That egg carries A and sperm
  carries a and vice versa,
• P x q p x q 2pq and aa is q2 thus the
  distribution of genotypes among the zygotes are
  p2 2pq q2 1
• Following HW law we can predict the freq. Of
  allele A in the new gene pool will be f(A)
• p2 ½ 2pq p2 pq since pq 1 q
  1-p
• p2 p(1-p) p2 p -
  p2 p

30

• Therefore after 1 generation of random mating the
  genotype frequencies will remain p2 2pq q2
  this type of a popn is said to be in HW
  equilibrium the maximum freq. Of a heterozygote
  is 0.5, this happens only when A and a are 0.5
  I.e. P0.5, q 0.5 so 2pq 0.5
• When gene frq. are betn 0.33 and 0.66, the hets
  are the most common genotype and when the freq.
  Of one allele is low the hom. For that allele is
  rarest genoptye

31

• Estimating gene freq. With HW
• Albinism in humans (hom.recessive)
• Freq. Is about 1 in 40 000 humans or 0.000025 so
  it is the freq. Of aa genotype
• H-W says q2 0.000025 then q 0.005 p 1-q
  0.995
• Allele freq. For albinism 0.005 and wild type
  allele freq. 0.995 and hetrozygote freq. 2
  pq 2 x 0.005 x 0.995 0.00995 almost 1 of the
  popn can be carriers

32

• 1. Infinitely large population
• No such population actually exists.
• The effect that is of concern is genetic drift a
  problem in small populations.
• Genetic drift - is a change in gene frequency
  that is the result of chance deviation from
  expected genotypic frequencies.
• 2. Random mating
• Random mating - matings in a population that
  occur in proportion to their allelic frequencies
• For example, if the allelic frequencies in a
  population are
• f(M) 0.91f(N) 0.09 then the probability of
  MM individuals occurring is 0.91 x 0.91 0.828.
• If a significant deviation occurred, then random
  mating did not happen in this population.

33

• Point to remember about random mating  Within a
  population, random mating can be occurring at
  some loci but not at others.
• Examples of random mating loci - blood type, RFLP
  patterns
• Examples of non-random mating loci - intelligence
  , physical stature
• 3. No evolutionary forces affecting the
  population
• The principal forces are
• Mutation
• Migration
• Selection
• Some loci in a population may be affected by
  these forces, and others may not those loci not
  affected by the forces can by analyzed as a
  Hardy-Weinberg population

34

• A species evolves when gene frequencies changes
  and the species moves it to a higher level of
  adaptation for a specific ecological niche.
• Mutation of alleles and migration of individuals
  with those new alleles will create variation in
  the population. Selection will then choose the
  better adapted individuals and the population
  will have evolved.
• Example peppered moth in England
• The moth can be either dark or light colored.
• Prior to the industrialization of central
  England, the light-colored allele was most
  prevalent. The light-colored moths would hide on
  the white-barked trees and avoid bird predation.

35

• But the pollution generated by the new industries
  stained the light-colored trees dark.
• Gradually the light-colored moth was attacked and
  that allele became much less prevalent.
• In its place, the dark-colored allele became the
  most predominant allele because moths that
  carried that allele could camouflage themselves
  on the stained trees and avoid being eaten by
  their bird predators.
• The population had evolved to a higher adaptive
  condition.

36

• Mutation
• Classified as beneficial, harmful or neutral
• Can occur by point mutations (changes in a single
  nucleotide) or small insertions or deletions of
  the nucleotide sequence.
• Harmful mutations are lost if they reduce fitness
  
• If fitness is improved by a mutation, then the
  frequency of that allele will increase from
  generation to generation
• The mutation could be a change in one allele to
  resemble one currently in the population, for
  example from a dominant to a recessive allele.
• The mutation could generate an entirely new
  allele

37

• Migration
• Migration will change gene frequencies by
  bringing more copies of an existing allele or by
  bringing in a new allele that has arisen by
  mutation.
• Because mutations do not occur in every
  population, migration will be required for that
  allele to spread throughout that species, - will
  occur only after the migrant has successfully
  mated with an individual in the population. The
  term that is used to described this introduction
  of new alleles is gene flow.
• The two effects of migration are to
• increase variability within a population
• prevent a population of that species from
  diverging to the extent that it becomes a new
  species

38

• Selection
• A population undergoes selection when certain
  alleles are preferentially found in a new
  generation because of the increased fitness of
  the parent.
• The alleles in the individual with increased
  fitness will increase in frequency in the
  population.

39

• Calculating genotypic frequencies form number of
  individuals
• Genotype of Individuals Genotypic
• 
  frequencies
• MM 1787 MM 1787/6129
  29
• MN 3039 MN 3039/6129
  50
• NN 1303 NN 1303/6129
  21
• Total 6129
• The allele frequency for the M allele will be
• f(M) (2 x 1787) 3039/12,258 0.5395
• and the frequency for the N allele will be
• f(N) (2 x 1303) 3039/12,258 0.4605

40

• The following example will illustrate that two
  different populations from the same species do
  not have to have the same allelic frequencies.
• Percent
• Location MM MN NN p q
• Greenland 83.5 15.6 0.90 0.92 0.08
• Iceland 31.2 51.5 17.30 0.57 0.43
• Clearly the allelic frequencies vary between
  these populations

41
(No Transcript)