Equilibrium states

1
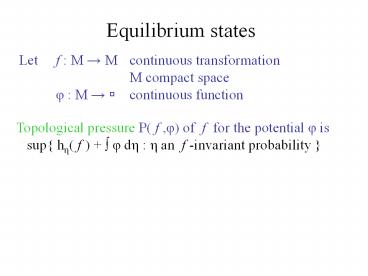
Equilibrium states
Let f M ? M continuous transformation M
compact space f M ? ? continuous function
Topological pressure P( f ,f) of f for the
potential f is sup h?( f ) ? f d? ? an f
-invariant probability
2
Equilibrium states
Let f M ? M continuous transformation M
compact space f M ? ? continuous function
Topological pressure P( f ,f) of f for the
potential f is sup h?( f ) ? f d? ? an f
-invariant probability
Equilibrium state of f for f is an f -invariant
probability µ on M such that hµ ( f ) ? f dµ
P( f ,f)
Rem If f 0 then P( f ,f) htop( f )
topological entropy. Equilibrium states are the
measures of maximal entropy.
3

• Fundamental Questions
• Existence and uniqueness of equilibrium states
• Ergodic and geometric properties
• Dynamical implications

4

• Fundamental Questions
• Existence and uniqueness of equilibrium states
• Ergodic and geometric properties
• Dynamical implications

Sinai, Ruelle, Bowen (1970-76) equilibrium
states theory for uniformly hyperbolic (Axiom A)
maps and flows.
Not much is known outside the Axiom A setting,
even assuming non-uniform hyperbolicity i.e. all
Lyapunov exponents different from zero.
I report on recent results by Krerley Oliveira
(2002), for a robust class of non-uniformly
expanding maps.
5
Uniformly hyperbolic systems

• A homeomorphism f M ? M is uniformly hyperbolic
  if
• there are C, c, e, d gt 0 such that, for all n ?
  1,
• d( f n( x), f n( y)) ? C e?cn d( x, y) if y ?
  Ws( x,e).
• d( f ?n( x), f ?n( y)) ? C e?cn d( x, y) if
  y ? Wu( x,e).
• Ws( x,e) ? Wu( y,e) has exactly one point if d(
  x, y) ? d,
• and it depends continuously on ( x, y).

Thm (Sinai, Ruelle, Bowen) Let f be uniformly
hyperbolic and transitive (dense orbits). Then
every Hölder continuous potential has a unique
equilibrium state.
6
Gibbs measures
Consider a statistical mechanics system with
finitely many states 1, 2, , n, corresponding to
energies E1, E2, , En, in contact with a heat
source at constant temperature T.
Physical fact state i occurs with probability
e?ß Ei ? e?ß Ej
1 ?T
µi
ß
n 1
7
Gibbs measures
Consider a statistical mechanics system with
finitely many states 1, 2, , n, corresponding to
energies E1, E2, , En, in contact with a heat
source at constant temperature T.
Physical fact state i occurs with probability
e?ß Ei ? e?ß Ej
1 ?T
µi
ß
n 1
?
The system minimizes the free energy E ? T S
?i µi Ei ? T ?i µi log µi
energy
entropy
8
Now consider a one-dimensional lattice
gas ??? ? ? ? ? ? ? ? ???
?-2 ?-1 ?0 ?1 ?2

?i ?1, 2, , N configuration is a sequence ?
? ?i

• Assumptions on the energy (translation
  invariant)
• associated to each site i A( ?i )
• interaction between sites i and j 2 B( i ?
  j , ?i , ?j )
• Total energy associated to the 0th site
• E(?) ? A(?0) ?k?0 B (k, ?0 , ?k ).
• Assume B is Hölder i.e. it decays exponentially
  with k.

9
Let T be the left translation (shift) in
configuration space.
Thm There is a unique translation-invariant
probability µ in configuration space admitting a
constant P such that, for every ? , This µ
minimizes the free energy among all
T-invariant probabilities.
µ ( ? ?i ?i for i 0, , n-1 ) ? exp (
? n P ? S ß E( Tj? ) )
n-1 j0
10
Let T be the left translation (shift) in
configuration space.
Thm There is a unique translation-invariant
probability µ in configuration space admitting a
constant P such that, for every ? , This µ
minimizes the free energy among all T-invariant
probabilities.
µ ( ? ?i ?i for i 0, , n-1 ) ? exp (
? n P ? S ß E( Tj? ) )

Gibbs measure
n-1 j0
P pressure of T for f ?ß E
11
Finally, uniformly hyperbolic maps may be reduced
to one-dimensional lattice gases, via Markov
partitions

R( j)
R( i)


f (R( i))
Fixing a Markov partition R R(1), , R( N) of
M , we have a dictionary
x ? M ? itinerary ?n relative to R f M ?
M ? left translation T f M ? ? ? ?ß E
?E? ?T P( f, f) ? pressure P of ?ß E h?( f )
? f d? ? free energy E ? T S
12
Equilibrium states and physical measures
Suppose M is a manifold and f M ? M is a
C1Hölder transitive Anosov diffeomorphism.
Consider the potential
f(x) ? ? log det (Df Eu( x))
Thm (Sinai, Ruelle, Bowen) The equilibrium state
µ is the physical measure of f for Lebesgue
almost every point x for every continuous
function ? M ? ?.
n-1 j0
1 n
? ?( f j( x) ) ??? dµ
13

• For non hyperbolic (non Axiom A) systems
• Markov partitions are not known to exist in
  general
• When they do exist, Markov partitions often
  involve
• infinitely many subsets
• ? lattices with infinitely many states.

Bressaud, Bruin, Buzzi, Keller, Maume, Sarig,
Schmitt, Urbanski, Yuri, unimodal maps,
piecewise expanding maps (1D and higher), finite
and countable state lattices, measures of maximal
entropy.
Assuming non-uniform hyperbolicity, there has
been progress concerning physical measures
14
Physical measures for non-hyperbolic maps
Thm (Alves, Bonatti, Viana) Let f M ? M be a
C2 local diffeomorphism non-uniformly
expanding lim ? log Df ( f
j(x))?1 lt ? c lt 0 (? all Lyapunov exponents
are strictly positive) at Lebesgue almost every
point x ? M.
n-1 j0
1 n
15
Physical measures for non-hyperbolic maps
Thm (Alves, Bonatti, Viana) Let f M ? M be a
C2 local diffeomorphism non-uniformly
expanding lim ? log Df ( f
j(x))?1 lt ? c lt 0 (? all Lyapunov exponents
are strictly positive) at Lebesgue almost every
point x ? M.
n-1 j0
1 n
Then f has a finite number of physical (SRB)
measures, which are ergodic and absolutely
continuous, and the union of their basins
contains Lebesgue almost every point.
16
These physical measures are equilibrium states
for the potential f ? log det D f . Under
additional assumptions, for instance
transitivity, they are unique.
17
These physical measures are equilibrium states
for the potential f ? log det D f . Under
additional assumptions, for instance
transitivity, they are unique.
One difficulty in extending to other potentials
How to formulate the condition of non-uniform
hyperbolicity ? Most equilibrium states should be
singular measures ...
Rem (Alves, Araújo, Saussol, Cao) Lyapunov
exponents positive almost everywhere for every
invariant probability ? f uniformly expanding.
18
A robust class of non-uniformly expanding maps

• Consider C1 local diffeomorphisms f M ? M such
  that there is a partition RR(0) , R(1) ,, R(
  p) of M such that f is injective on each R( i)
  and
• f is never too contracting ?D f ?1? lt 1 d
• f is expanding outside R(0) ?D f ?1? lt ? lt 1
• every f ( R( i)) is a union of elements of R and
  the forward orbit of R( i) intersects every R( j)
  .

19
Thm (Oliveira) Assume 1, 2, 3 with dgt0 not too
large relative to ?. Then for very Hölder
continuous potential f M ? ? satisfying there
exists a unique equilibrium state µ for f, and
it is an ergodic weak Gibbs measure.
99 100
max f - min f ? htop( f ) ()
In particular, f has a unique measure of maximal
entropy.
20
Thm (Oliveira) Assume 1, 2, 3 with dgt0 not too
large relative to ?. Then for very Hölder
continuous potential f M ? ? satisfying there
exists a unique equilibrium state µ for f, and
it is an ergodic weak Gibbs measure.
99 100
max f - min f ? htop( f ) ()
There is Kgt1 and for µ-almost every x there is a
non-lacunary sequence of n ? ? such that
µ(x ? M f j( x) ? R( ?j ) for j0, , n-1)
?K
K?1?
n-1 j0
exp( ?n P S f ( f j( x) )
In particular, f has a unique measure of maximal
entropy.
21
Step 1 Construction of an expanding reference
measure Transfer operator Lf g( y) ?
ef(x) g( x) acting on functions continuous on
each R( j). Dual transfer operator Lf acting
on probabilities by ?g d(L f ?) ? (Lf g) d ?
S
S
f ( x) ? y


22

L1 There exists a probability ? such that Lf? ?
?? for some ? gt exp(max f htop(
f )).
1 100
23

L1 There exists a probability ? such that Lf? ?
?? for some ? gt exp(max f htop(
f )).
1 100
?
?
L2 Relative to ?, almost every point spends only
a small fraction of time in R( 0).
24

L1 There exists a probability ? such that Lf? ?
?? for some ? gt exp(max f htop(
f )).
1 100
?
?
L2 Relative to ?, almost every point spends only
a small fraction of time in R( 0).
?
lim ? log Df ( f j(x))?1 lt ?
c.
L3 The probability ? is expanding ??almost
everywhere, Moreover, ? is a weak Gibbs
measure.
n-1 j0
1 n
25
Step 2 Construction of a weak Gibbs invariant
measure
S
S
n
Snf(x)
Iterated transfer operator Lf g( y) ?
e g( x)
n
f ( x) ? y
26
Step 2 Construction of a weak Gibbs invariant
measure
S
S
n
Snf(x)
Iterated transfer operator Lf g( y) ?
e g( x)
n
f ( x) ? y
S
Snf(x)
Modified operators Hn,f g( y) ? e
g( x) where the sum is over the
pre-images x for which n is a hyperbolic time.
hyperbolic
27
Def n is hyperbolic time for x ?D f j( f
n?j( x))?1? ? e?cj/2 for every 1 ? j ? n
expansion ? ecj/2
? ? ??? ? ??? ?
x f ( x) f n?j( x) f n( x)
n-1 j0
1 n
Lemma If lim ? log Df ( f
j(x))?1 lt ? c then a definite positive
fraction of times are hyperbolic.
28
L4 The sequence of functions ??n Hn,f1 is
equicontinuous.
L5 If h is any accumulation point, then h is a
fixed point of the transfer operator and µ ? h ?
is f-invariant. Moreover, µ is weak Gibbs
measure and equilibrium state.
29
L4 The sequence of functions ??n Pn,f1 is
equicontinuous.
L5 If h is any accumulation point, then h is a
fixed point of the transfer operator and µ ? h ?
is f-invariant. Moreover, µ is weak Gibbs
measure and equilibrium state.
Step 3 Conclusion proof of uniqueness
L6 Every equilibrium state is a weak Gibbs
measure.
L7 Any two weak Gibbs measures are equivalent.
30
Rem These equilibrium states are expanding
measures all Lyapunov exponents are positive ?
condition ().
Question Do equilibrium states exist for all
potentials (not necessarily with positive
Lyapunov exponents) ?
31
Rem These equilibrium states are expanding
measures all Lyapunov exponents are positive ?
condition ().
Question Do equilibrium states exist for all
potentials (not necessarily with positive
Lyapunov exponents) ?
Oliveira also proves existence of equilibrium
states for continuous potentials with
not-too-large oscillation, under different
methods and under somewhat different assumptions.