SIGNAL DETECTION THEORY - PowerPoint PPT Presentation
Title:
SIGNAL DETECTION THEORY
Description:
SIGNAL DETECTION THEORY A situation is described in terms of two states of the world: a signal is present ( – PowerPoint PPT presentation
Number of Views:60
Avg rating:3.0/5.0
Title: SIGNAL DETECTION THEORY
1
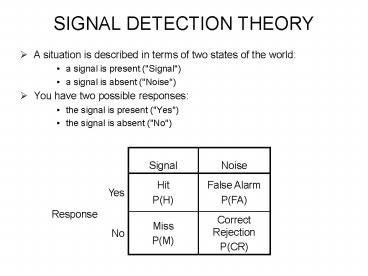
SIGNAL DETECTION THEORY
- A situation is described in terms of two states
of the world - a signal is present ("Signal")
- a signal is absent ("Noise")
- You have two possible responses
- the signal is present ("Yes")
- the signal is absent ("No")
Signal Noise
Response Yes Hit P(H) False Alarm P(FA)
Response No Miss P(M) Correct Rejection P(CR)
2
- The theory assumes that what you are doing is
- First, you collect sensory evidence concerning
the presence or absence of the signal. - Next, you decide whether this evidence
constitutes a signal. This means that you must
have some criterion C that you use as a "cutoff"
if the evidence is less than C, you decide "No"
if the evidence exceeds C, you decide "Yes".
3
Measures of Performance in SDT
- 1. Response bias (b)
- We can describe performance in terms of response
bias you may be prone to say "yes" (which is
"risky") or you may be prone to say "no" (which
is "conservative"). - Response bias the ratio of the heights of the two
curves at the cutoff point and is measured by the
quantity - P(X/S)
- ß
- P(X/N)
- where X "evidence variable
- S signal
- N noise
- Note your book has a simplified equation
for representing ?. You may refer to whichever
best helps you understand the concept of response
bias.
4
- Studies of human performance show that humans do
change ß in response to changes in probabilities
and payoffs -- but not as much as they should! - This phenomenon is called sluggish beta.
- Note the terms risky and conservativerefer
only to a persons propensity to say yes
(signal) or no (noise). - Examples
- radiologists reading x-rays for signs of tumors
- radar operators on a battle ship looking for
incoming enemy aircraft - scanning a parking lot for a parking space
5
- The cutoff (C) for determining the presence of a
signal vs the response bias parameter (ß). - Not the same but correlated.
- Risky strategy ß ? and C ?
- More conservative both C and ß ?
- Setting ß
- Strategy can be affected by relative costs and
values assigned to outcomes. - Examples
- radiologists reading x-rays for signs of tumors
- radar operators on a battle ship looking for
incoming enemy aircraft
6
Measures of Performance in SDT
- 2. Sensitivity (d)
- Signal detection theory distinguishes response
bias from sensitivity - a function of the keenness or sensitivity of the
human's detection mechanisms and the relative
strength of the signal in noise. - For example, a person may be "risky" (i.e., prone
to say "Yes, I detect a signal") but may have bad
eyesight (or be looking at a very fuzzy screen)
and thus may often miss signals because of this
low sensitivity. - Table 4.5 on page 84 of your textbook gives some
possible values of d corresponding to observed
P(H) and P(FA). This value may also be
calculated from the probabilities of a hit and a
false alarm.
7
Receiver Operating Characteristic (ROC) curve
- Plots the probability of a hit against the
probability of a false alarm. - Each curve represents the same sensitivity at
different levels of response bias.
8
Your Turn .
- An experiment was performed to determine how
students who participated in a distance learning
course responded to a signal from the instructor
indicating that they had been called on to
respond to a question. The results for two of the
students using a particular type of signal and
with varying feedback (i.e., rewards for
responding) are given in the table below. Plot
the results for both students on the same ROC
curve. Who is more sensitive? Identify risky
and conservative behavior. Comment on the
results.
Feedback P(fa) P(hit)
Negative 0.1 0.3
Student 1 Neutral 0.33 0.55
Positive 0.65 0.78
Negative 0.05 0.33
Student 2 Neutral 0.35 0.68
Positive 0.7 0.86