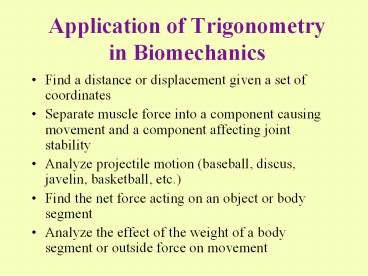
Application of Trigonometry in Biomechanics

1
Application of Trigonometry in Biomechanics

• Find a distance or displacement given a set of
  coordinates
• Separate muscle force into a component causing
  movement and a component affecting joint
  stability
• Analyze projectile motion (baseball, discus,
  javelin, basketball, etc.)
• Find the net force acting on an object or body
  segment
• Analyze the effect of the weight of a body
  segment or outside force on movement

2
Trigonometry - Basics

• Q F - general
• a ß g 180

A
ß
g
B
C
a
3
Right triangle - Basics
90

• Q F - general
• a ß 90
• Pythagorean Theorem
• A2 B2 C2

A
ß
g
B
C - hypotenuse
a
4
2000 Super Bowl St. Louis vs. TennesseeKurt
Warner of the St. Louis Rams is standing on his
own 20 yard line and is 25 yards from the right
sideline. He throws the ball to a receiver that
on the opponents 35 yard line and is 2 yards
from the right sideline when he catches the ball.
A) What was the horizontal distance covered
by the ball before it was caught?B) If the ball
was in the air for 3.5 seconds, what was the
average forward or horizontal velocity of the
ball during flight?
5
Football Field
50 yd line
quarterback
receiver
C
B
A
6

• Given position 1 (pos1) 20 yds
• position 2 (pos2) 100 yds 35 yds 65 yds
• Dt 3.5 sec
• Quarterback 25 yards from sideline (DQ-gtS )
• Receiver 2 yards from sideline (DR-gtS )
• Find
• The horizontal distance traveled by the football
  (df)
• The average horizontal velocity of the football
  (vf)

7
Diagram and Derived Information

• A 65 yds 20 yds 45 yds
• B DQ-gtS - DR-gtS 25 yds 2 yds 23 yds
• C Horiz. Distance (Range) traveled by the
  football df

8
Formulas

• A) C2 A2 B2
• B) df C
• C) vf df /Dt

Solutions A) Df Df 50.53 yds 151.61
ft B) vf 151.61 ft / 3.5 sec vf 43.32
ft / sec
(45 yds)2 (23 yds)2
9
Right triangle - Basics

• sin q length of the opposite side
• length of the hypotenuse
• sin a A/C
• cos q length of the adjacent side
• length of the hypotenuse
• cos a B/C
• tan q length of the opposite side
• length of the adjacent
• tan a A/B

A
ß
g
B
a
C - hypotenuse
10
Trig. example

• Justine is performing leg extension exercises.
  The distance from her knee to her foot 25 cm.
  If the position of her leg is 50 below
  horizontal, what are the horizontal and vertical
  distances from her knee to her foot?
• Given dk-gtf 25 cm
• ß 50
• Find x (horizontal - side A) and y (vertical -
  side B)

11
Trig. example

• Given dk-gtf 25 cm C
• ß 50
• Find x (horizontal - side A) and y (vertical -
  side B)
• DIAGRAM

A or x
ß 50
B or y
C
12
Trig. example

• Formula sin q opp/hyp
• cos q adj/hyp
• Solutions
• cos 50 x/25 cm
• x 25 cm cos 50
• x 16.1 cm
• sin 50 y/25 cm
• y 25 cm sin 50
• y 19.15 cm

A
ß 50
B
C
13
Law of cosines

• can be used for any triangle
• c2 a2 b2 - 2abcosq

Pythagorean theorem
Correction for angle q
a
q
b
ß
a
c
14
Law of sines

• can be used for any triangle
• The ratio between a side and the sine of the
  opposite angle is constant for any given triangle
• a/sina b/sinß c/sing

a
q
b
ß
a
c
15
Example

• The vastus lateralis is pulling with a force of
  200 N on the quadriceps tendon. The vastus
  medialis is pulling with a force of 160 N on the
  quadriceps tendon. If the forces of the two
  muscles pull at an angle of 28 with each other,
  find the net force.
• Given FVL 200 N FVM 160 N
• q 28
• Find Fnet

16
Example

• Diagram
• Formula
• c2 a2 b2 - 2abcosq
• To find net, add, the arrows representing the
  force tip to tail NET is the shortcut

VL
Vm
28
160N
28
Fnet
200 N
17
Example

• Formula
• c2 a2 b2 - 2abcosq
• To find net, add, the arrows representing the
  force tip to tail NET is the shortcut

28
Fnet
Q 180 - 28 152
18
Example

• Solution
• c2 a2 b2 - 2abcosq
• c ?(2002 1602 - 2200160cos 152)
• c ?(65,600 - 64,000 (-0.883))
• c ?(122,112N2)
• C 349.44 N

160N
Fnet
28
152
200 N