Trend Lines

1
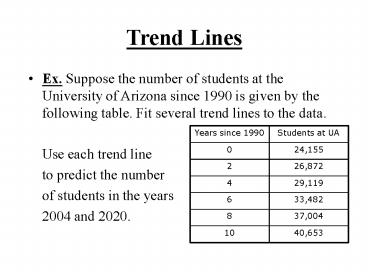
Trend Lines

• Ex. Suppose the number of students at the
  University of Arizona since 1990 is given by the
  following table. Fit several trend lines to the
  data.
• Use each trend line
• to predict the number
• of students in the years
• 2004 and 2020.

Years since 1990 Students at UA
0 24,155
2 26,872
4 29,119
6 33,482
8 37,004
10 40,653
2
Trend Lines

• Linear Approx. 46,956 students in 2004
• Approx. 73,756 students in 2020

3
Trend Lines

• Quadratic Approx. 49,976 students in 2004
• Approx. 100,478 students in 2020

4
Trend Lines

• Exponential Approx. 50,493 students in 2004
• Approx. 117,710 students in 2020

5
Demand, Revenue, Cost, Profit

• Ex. Suppose the following data represents the
  total number of shoes sold in a month at a
  particular price in
• dollars. Use a second
• degree polynomial
• trend line to find a
• formula for the Demand
• function

Number of shoes Price
200 76
350 68
450 59
700 53
900 40
1100 24
6
Demand, Revenue, Cost, Profit
7
Demand, Revenue, Cost, Profit

• Generating graph of revenue
• Use Plotting Points method
• Use interval 0, q where q is the q-intercept
  from Demand graph

8
Demand, Revenue, Cost, Profit
9
Demand, Revenue, Cost, Profit

• Optimal quantity to maximize revenue is about 800
  units.
• Maximum Revenue is about 36,000
• Price should be about 45

10
Demand, Revenue, Cost, Profit

• Ex. If the fixed cost is 2000 and the variable
  cost is 35 per unit, determine a formula for
  total cost and graph C(q).
• C(q) 2000 35q

11
Demand, Revenue, Cost, Profit
12
Demand, Revenue, Cost, Profit

• Graph of Revenue and Cost (determine profit)

13
Demand, Revenue, Cost, Profit

• Profit function P(q) R(q) - C(q)

14
Demand, Revenue, Cost, Profit

• Project (Demand)

15
Demand, Revenue, Cost, Profit

• Project
• - Keep units straight
• - Prices (dollars)
• - Revenue (millions of dollars)
• - Quantities in test markets (whole units)
• - Quantities in national market (thousands of
  units)

16
Demand, Revenue, Cost, Profit

• Project (Demand)
• - Convert test market data to national data
• - Determine quadratic demand trend line (8
  decimal places)

17
Demand, Revenue, Cost, Profit

• Project (Revenue)
• - Units should be millions of dollars
• - Typically
• - Must adjust for units

18
Demand, Revenue, Cost, Profit

• Project (Revenue)
• Must convert revenue to millions of dollars
• Use this formula

19
Demand, Revenue, Cost, Profit

• Project (Revenue)

20
Demand, Revenue, Cost, Profit

• Project (Cost)
• - Use COST function from Visual Basic Editor
• (will be explained in class)

21
Demand, Revenue, Cost, Profit

• Project (Cost)
• 7 parameters for COST function
• quantity
• fixed cost
• batch size 1
• batch size 2
• marginal cost 1
• marginal cost 2
• marginal cost 3

22
Demand, Revenue, Cost, Profit

• Project (Revenue and Cost)
• - Graph both R(q) and C(q)
• - Use plotting points method

23
Demand, Revenue, Cost, Profit

• Project (Revenue and Cost)

24
Demand, Revenue, Cost, Profit

• Project (Profit)

25
Demand, Revenue, Cost, Profit

• Project (Revenue and Cost)
• - Determine important information from graphs
• Break-even pts at about
  300,000 and 800,000 units
• (zero profit)
• Max profit at about 575,000
  units
• Negative profit q lt 300K
  and q gt 800K

Break-even pts
Largest gap max profit
26
Demand, Revenue, Cost, Profit

• Project (Revenue and Cost)
• - Determine important information from graphs
• Break-even pts at about
  300,000 and 800,000 units
• (zero profit)
• Max profit at about 575,000
  units
• Negative profit q lt 300K
  and q gt 800K

Max profit
Break-even pts
27
Demand, Revenue, Cost, Profit

• Project (What to do)
• - Create Demand graph using trend lines
• - Create Revenue and Cost graph
• - Create Profit graph