Chapter 20 Complex variables

1
Chapter 20 Complex variables
2
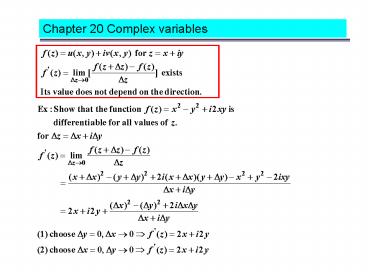
Chapter 20 Complex variables
3
Chapter 20 Complex variables
4
Chapter 20 Complex variables
20.2 Cauchy-Riemann relation
A function f(z)u(x,y)iv(x,y) is differentiable
and analytic, there must be particular connection
between u(x,y) and v(x,y)
5
Chapter 20 Complex variables
6
Chapter 20 Complex variables
7
Chapter 20 Complex variables
20.3 Power series in a complex variable
8
Chapter 20 Complex variables
20.4 Some elementary functions
9
Chapter 20 Complex variables
10
Chapter 20 Complex variables
11
Chapter 20 Complex variables
20.5 Multivalued functions and branch cuts
A logarithmic function, a complex power and a
complex root are all multivalued. Is the
properties of analytic function still applied?
(A)
(A)
(B)
(B)
12
Chapter 20 Complex variables
Branch point z remains unchanged while z
traverse a closed contour C about some point. But
a function f(z) changes after one complete
circuit.
Branch cut It is a line (or curve) in the
complex plane that we must cross , so the
function remains single-valued.
13
Chapter 20 Complex variables
14
Chapter 20 Complex variables
(A)
(B)
15
Chapter 20 Complex variables
20.6 Singularities and zeros of complex function
16
Chapter 20 Complex variables
17
Chapter 20 Complex variables
18
Chapter 20 Complex variables
19
Chapter 20 Complex variables
20
Chapter 20 Complex variables
20.10 Complex integral
21
Chapter 20 Complex variables
22
Chapter 20 Complex variables
23
Chapter 20 Complex variables
24
Chapter 20 Complex variables
25
Chapter 20 Complex variables
20.11 Cauchy theorem
26
Chapter 20 Complex variables
27
Chapter 20 Complex variables
28
Chapter 20 Complex variables
20.12 Cauchy