Fraunhofer diffraction from Circular apertures:

1
Lecture 20
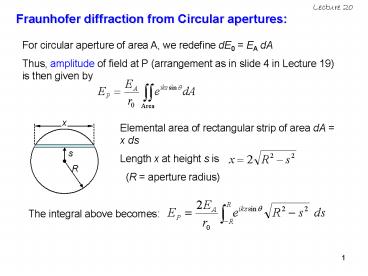
Fraunhofer diffraction from Circular apertures
For circular aperture of area A, we redefine dE0
EA dA Thus, amplitude of field at P
(arrangement as in slide 4 in Lecture 19) is then
given by
Elemental area of rectangular strip of area dA
x ds Length x at height s is (R aperture
radius)
The integral above becomes
2
Circular apertures
Using substitution v s/R and ? kR sin ? we
can rewrite as
This standard definite integral takes value of
J1(?) first-order Bessel function of the first
kind, given by
From the series expansion, the ratio J1(?)/? has
limit ½ as ? ? 0 and this Bessel function
oscillates with its amplitude decreasing as ?
gets larger.
3
Circular apertures (Bessel function)
This is the order of Bessel function that we are
interested in
4
Circular apertures (Bessel function)
Zeroes of Bessel function Jn(x) occur at x
s n0 n1 n2 n3 n4 n5
1 2.405 3.832 5.135 6.379 7.586 8.780
2 5.520 7.016 8.147 9.760 11.064 12.339
3 8.654 10.173 11.620 13.017 14.373 15.700
4 11.792 13.323 14.796 16.224 17.616 18.982
5 14.931 16.470 17.960 19.410 20.827 22.220
6 18.071 19.616 21.117 22.583 24.018 25.431
7 21.212 22.760 24.270 25.749 27.200 28.628
8 24.353 25.903 27.421 28.909 30.371 31.813
9 27.494 29.047 30.571 32.050 33.512 34.983
s order of zero
5
Circular apertures (Bessel function)
Thus irradiance for circular aperture of diameter
D can now be written as
(20-1)
I0 irradiance at ? ? 0 or at ? 0.
From the Table of zeroes of Bessel function, the
1st zero of J1(?) occurs at ? 3.832, thus,
central maximum of irradiance falls to zero when
(20-2)
Comparing functions J1(x)/x and (sin x)/x
both approaches a maximum when x ? 0, thus,
their irradiances is greatest at center of
pattern (? 0) their pattern is symmetrical
about optical axis through center of circular
aperture at 1st minimum, m 1 for slit pattern
(in m? b sin ?) analogous to m 1.22 for
circular aperture
6
Circular apertures (Bessel function)
Central maximum is a circle of light that
corresponds to the zeroth order of diffraction
called the Airy disc. The Airy disc is the
diffracted image of the circular aperture.
The pattern has a rotational symmetry about the
optical axis
From (20-2) given by D sin ? 1.22?, the
far-field angular radius of this Airy disc is
approximated (sin ? ? ?) to
(20-3)
7
Resolution

• A telescope with a round objective is subject to
  diffraction effects as with a circular aperture.
• sharpness of the primary image of distant star is
  then limited by diffraction
• this image occupies the region of Airy disc
• This inevitable diffraction blurring in the image
  restricts the resolution of the instrument (in
  terms of being able to produce distinct images
  for distant object points)

Diffraction-limited images of two point objects
formed by a lens. As long as the Airy disc are
well separated, the images are well resolved.
When S1 and S2 are too close, their image
patterns overlap, and it will be difficult to
resolve as distinct object points.
8
Rayleigh criterion
The Rayleigh criterion for just resolvable images
requires the centers of the image patterns to be
not less than the angular radius of the Airy disc
(i.e. the distance between the first diffraction
minimum of the image of one source point and the
maximum of another)
(D may be the diameter of the objective lens of
telescope)
9
Quantitative example
Suppose each lens on a pair of binoculars has
diameter 35 mm. How far apart must two stars be
before they are theoretically resolvable by
either of the lenses?
1.92?10?5 rad ? 1.1?10?3 ? 0.066 4 of arc
(or 4 arcseconds) 550 nm has been chosen as the
average wavelength for visible light (ranges from
400 nm 700 nm)
If the stars are near the center of our galaxy, a
distance d of around 30,000 light years, then
their actual separation s is approximately s d
??min (30,000)(1.92?10?5) 0.58 light years
If we detect them by their long-wavelength radio
waves (say 1 km), replacing the lenses with dish
antennas, the resolution is less.
10
Rayleigh criterion for single slit differs from
that of circular aperture
Single slit Circular aperture

In the case of a microscope, the minimum
separation xmin of two just-resolved objects near
the focal plane of its objective lens (diameter
D, focal length f) is
Ratio D/f ? numerical aperture (typical value
1.2) thus xmin ? ? Thats why UV, X-ray and
electron (shorter ?) microscopes have
high-resolution
11
Resolution limits due to diffraction in the human
eye
Diffraction by eye with pupil as aperture limits
the resolution of objects subtending angle ?min

• Night vision (pupil is larger at 8mm) has
  higher resolution than daylight vision.
  Unfortunately, there isnt enough light to take
  advantage of this.
• At bright noon, pupil diameter 2 mm (taking
  average wavelength of 550 nm) theoretical
  resolvable angular distance is
• Thus for 2 lines at 1 mm apart, they must not be
  farther than (10?3/3.36?10?4 2.98 m) away in
  order to be barely-discernable (theoretically).

12
Resolution limits due to diffraction in the
telescope
A telescope has an objective of 50 cm diameter.
Its angular limit of resolution at wavelength 550
nm is
If it is used to see two objects on the surface
of the Moon (Earth-Moon distance 3.844?108 m),
the separation of the two objects such that they
are theoretically resolvable is
If the objective lens has focal length 2 m, the
corresponding distance between the images of the
objects on the focal plane of the objective is
The pupil of your eye is 4 mm in diameter when
you view the images on the objective. What is the
farthest position of your eye from the focal
plane of the objective such that you are just
able to resolve them?
13
Double Slit Diffraction
In the case of double slit, we modify the limits
of integration of the equation for the field at
point P on the screen for single slit (Eq. 19-4)
and expressing for the amplitude only
(20-5)
Specification of slit width and separation for
double slit diffraction
Integration and substitution of the limits yields
14
Double Slit Diffraction
Then substituting a term involving slit width b
and a term involving slit separation a expressed
as
(20-6)
will result in
Using Eulers equation, we get
(20-7)
Thus, the irradiance is
(20-8)
15
Double Slit Diffraction
Therefore
where
(21-5)
Double-slit interference
Single-slit diffraction
Maximum irradiance in pattern centre for Double
slit is 4 X that of single slit Rewriting the
double-slit interference term
Compare to expression for Youngs double-slit
16
Double Slit Diffraction
Interference (solid line) and diffraction (dashed
line) functions for double-slit Fraunhofer
diffraction slit separation 6 X slit width (a
6b)
Resultant irradiance for the double slit above
17
Double Slit Diffraction
Diffraction minima occur for ? m? where m
?1, ?2, , or when at condition m? b sin
? Interference maxima occur for ? p?
where p 0, ?1, ?2, , or when at condition
p? a sin ? When diffraction minima coincide
(at the same ?) with interference fringe maxima,
the fringe goes missing. Condition for missing
orders
(20-9)
(20-10)
This is achieved when slit separation is a
perfect integral of the slit width, i.e., a
nb In the example in previous slide a 6b, thus,
the missing orders are when p ?6, ?12, ...