Chapter 21: Superposition and standing wave

1
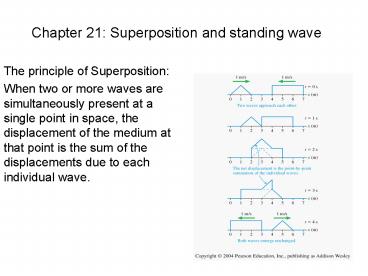
Chapter 21 Superposition and standing wave

• The principle of Superposition
• When two or more waves are simultaneously present
  at a single point in space, the displacement of
  the medium at that point is the sum of the
  displacements due to each individual wave.

2
Stop to think 21.1 page 648Stop to think
21.2 page 654Stop to think 21.3 page 658

• Example 21.1 page 651
• Example 21.2 page 654
• Example 21.5 page 656
• Example 21.6 page 658

3
Standing wave

• Ex there are two waves
• The resultant wave function is

Notice, in this function, does not contain a
function of (kx?t). So it is not an expression
for a traveling wave
4
Standing wave on a String

• A standing wave can exist on the string only if
  its wavelength is one of the values given by
• F1V/2L fundamental frequency.
• The higher-frequency standing waves are called
  harmonics,
• ex. m 2, second harmonics
• m3 third harmonics

Node
Antinode
5
Stop to think A standing wave on a string
vibrates as shown at the figure. Suppose the
tension is quadrupled while the frequency and the
length of the string are held constant. which
standing-wave pattern is produced
Answer a
6
Standing Sound Waves

• Open-open or closed-closed tube

m 1,2,3
7
Open-closed tube
8
Problem 21.54

• Model A stretched wire, which is fixed at both
  ends, creates a standing wave whose fundamental
  frequency is f1 V(wire)/2L. A standing wave in
  an open-closed tube exhibits an antinode at the
  open end and a node at the closed end.

9
469 Hz
The nodes of standing wave are spaced ?/2, it is
36 cm, so the ? 72 cm The v 469/s x 0.72 m
338m/s. It is closed to 343m/s, the speed of
sound at 20 C
10
Interference in one dimension

• The phase
• The phase difference is
• Constructive interference ?F m(2p)
• Perfect destructive interference ?F (2m 1 )p