Vertical and horizontal shifts

1
Vertical and horizontal shifts

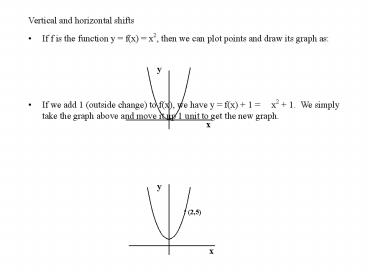
• If f is the function y f(x) x2, then we can
  plot points and draw its graph as
• If we add 1 (outside change) to f(x), we have y
  f(x) 1 x2 1. We simply take the graph
  above and move it up 1 unit to get the new graph.

y
x
y
(2,5)
x
2

• If we replace x by x2 (inside change) to form
  the function y f(x2) (x2)2, then the
  corresponding graph is obtained from the graph of
  y x2 by moving it 2 units to the left along the
  x-axis.
• If we replace x by x1 (inside change) to form
  the function y f(x1) (x1)2, then the
  corresponding graph is obtained from the graph of
  y x2 by moving it 1 unit to the right along the
  x-axis.

y
x
(-2,0)
y
x
(1,0)
3
If y g(x) is a function and k is a constant,
then the graph of

• y g(x) k is the graph of y g(x) shifted
  vertically by k units. If k gt 0, the shift is
  up, and if k lt 0, the shift is down.
• y g(xk) is the graph of y g(x) shifted
  horizontally by k units. If k gt 0, the shift
  is left, and if k lt 0, the shift is right.

Horizontal and vertical shifts of the graph of a
function are called translations.
4
An example which combines horizontal and vertical
shifts

• Problem. Use the graph of y f(x) x2 to
  sketch the graph of g(x) f(x2) 1 (x2)2
  1. Solution. The graph of g is the
  graph of f shifted to the right by 2 units and
  down 1 unit as shown below.

y
x
(2,-1)
5
Reflections and symmetry

• Suppose that we are given the function y f(x)
  as shown.
• If we define y g(x) f(x), then the graph of
  g may be obtained by reflecting the graph of f
  vertically across the x-axis as shown next.

y
x
y
x
6
Continuation of example from previous slide

• If we define y h(x) f(x), then the graph of
  h is obtained by reflecting the graph of f
  horizontally across the y-axis as shown next.
• Next, we define y p(x) f(x). The graph of
  p is obtained by reflecting the graph of f about
  the origin as shown next.

y
x
y
x
7
For any function f

• The graph of y f(x) is the reflection of the
  graph of y f(x) across the x-axis.
• The graph of y f(x) is the reflection of the
  graph of y f(x) across the y-axis.
• The graph of y f(x) is the reflection of the
  graph of y f(x) about the origin. Note
  that this reflection can be obtained by applying
  the two previous reflections in sequence.

8
Symmetries of graphs

• A function is called an even function if, for all
  values of x in the domain of f,
  The graph of an even function is symmetric across
  the y-axis. Examples of even functions are power
  functions with even exponents, such as y x2, y
  x4, y x6, ...
• A function is called an odd function if, for all
  values of x in the domain of f,
  The graph of an odd function is symmetric about
  the origin. Examples of odd functions are power
  functions with odd exponents, such as y x1, y
  x3, y x5, ...

9

• Problem. Is the function f(x) x3x even, odd,
  or neither? Solution. Since
  2 f(1) is not equal to f(1) 2, it follows
  that f is not even. Since
  f(x)
  f(x), it follows that f is odd.

y x3x
Note the symmetry about the origin.
10

• Problem. Is the function f(x) x even, odd,
  or neither? Solution. Since
  f(x) x f(x), it follows that f is
  even. Since 1 f(1) is not equal to
  f(1) 1, it follows that f is not odd.
• Question. Is it possible for a function to be
  both even and odd?

y x
Note the symmetry about the y-axis.
11
Combining shifts and reflections--an example

• In an earlier example, we discussed an investment
  of 10000 in the latest dotcom venture. This
  investment had a value of 10000(0.95)t dollars
  after t years. Suppose that we want to graph the
  amount of the loss after t years for this
  investment. The formula for the loss
  is 10000
  10000(0.95)t
• The loss is graphed on the next slide using
  Maple.

Shift Upwards
Reflect across t-axis
12
Use of Maple to graph loss on dotcom investment
gt plot(10000,10000-10000(0.95)t,t0..80,
colorblack,labels"t","L")
The graph of the loss has a horizontal asymptote,
L 10000.
13
Vertical Stretches and Compressions

• If f(x) x2 and g(x) 5x2, then the graph of g
  is obtained from the graph of f by stretching it
  vertically by a factor of 5 as the following
  Maple plot shows

14

• If f(x) x2 and g(x) -5x2, then the graph of g
  is obtained from the graph of f by stretching it
  vertically by a factor of 5 and then reflecting
  it across the x-axis as the following Maple plot
  shows

15

• If we compare the graphs of f(x) x2 and g(x)
  (1/2)x2, we notice that the graph of g can be
  found by vertically compressing the graph of f by
  a factor of 1/2.
• Generalizing the above examples yields the
  following If f is a function and k is a
  constant, then the graph of y kf(x) is
  the graph of y f(x) Vertically
  stretched by a factor of k, if k gt 1.
  Vertically compressed by a factor of k, if 0 lt k
  lt 1. Vertically stretched or
  compressed by a factor k and reflected
  across the x-axis, if k lt 0.

16
Vertical Stretch Factors and Average Rates of
Change

• If f(x) x2 and g(x) 5x2, we compute the
  average rates of change of the two functions on
  the interval 1,3 as follows
• The above computation illustrates a general
  fact

17

• If f(x) 4x2 and g(x) 4 (2x)2, then the
  graph of g is obtained from the graph of f by
  compressing it horizontally by a factor of 1/2 as
  the following Maple plot shows

18

• If f(x) 4x2 and g(x) 4 (0.5x)2, then the
  graph of g is obtained from the graph of f by
  stretching it horizontally by a factor of 2 as
  the following Maple plot shows

19

• Generalizing the two previous examples yields the
  following results for horizontal stretch or
  compression.
• If f is a function and k is a positive constant,
  then the graph of y f(kx) is the graph of
  f Horizontally compressed by a
  factor of 1/k if k gt 1. Horizontally
  stretched by a factor of 1/k if k lt 1.
  If k lt 0, then the graph of y f(kx) also
  involves a horizontal reflection about the y-axis.

20
The Family of Quadratic Functions

• A quadratic function is a function with a formula
  in one of the following forms
  Standard form y ax2bxc, where a, b, c, are
  constants, Vertex form
  y a(xh)2k, where a, h, k are
  constants,
• The graph of a quadratic function is called a
  parabola.
• Conversion from one form to the other for a
  quadratic function is discussed on the next
  slide.

21

• To convert a quadratic function from vertex form
  to standard form, simply multiply out the squared
  term. To convert from standard form to vertex
  form, we complete the square as illustrated in
  the following example.
• Example. Put the following quadratic function
  into vertex form by completing the
  square. (1) Factor out the
  coefficient of x2, which is 4.
  (2) Add and subtract the square of half the
  coefficient of the
  x-term.
  (3) Write the equation in vertex form.

perfect square
22
The Vertex of a Parabola

• Recall that the graph of a quadratic is called a
  parabola.
• The parabola corresponding to y
  a(xh)2k Has vertex
  (h, k). Has axis of
  symmetry x h.
  Opens upward if a gt 0 or downward if a lt 0.

23
Finding the vertex of a parabola

• Example. For the previous example, graph the
  parabola and find the vertex.
  We note that the vertex is at (3/2, 1). The
  graph follows

24
Finding a formula for a parabola

• If we know the vertex of a quadratic function and
  one other point, we can use the vertex form to
  find its formula, as shown in the following
  example.
• Example. A parabola has vertex at (3, 2) and
  (0, 5) is on the parabola. Find the formula for
  the corresponding quadratic, f(x). Use the
  vertex form with h 3 and k 2. This results
  in To
  find the value of a, we substitute x 0 and y
  5 into this formula, obtaining a 1/3. The
  formula is therefore

25
Finding a formula for a parabola, continued.

• If three points on a parabola are given, we can
  use the standard form of the corresponding
  quadratic to find the formula.
• Example. Suppose the points (0, 6), (1, 0), and
  (3, 0) are on a parabola. Find a formula for the
  parabola. Use the standard form y ax2bxc.
  Since (0, 6) is on the parabola, it follows that
  c 6. From the other two points, we
  have This system
  can be solved simultaneously for a and b. We
  obtain a 2 and b 8. Thus, the equation of
  the parabola is y 2x28x6.

26
Finding the zeros of a quadratic function.

• The zeros of a function f are values of x for
  which f(x) 0.
• In addition to the standard and vertex forms,
  some quadratic functions f(x) can also be
  expressed in factored form
• Example. Find the zeros of f(x) x2x 6. Set
  f(x) 0 and solve for x. We have x2x 6 0.
  We next express f(x) in factored form, so it will
  be easy to find the zeros.
  The zeros are x 3 and x 2. Note that these
  are the values r and s from the factored form.

27
Finding a formula for a parabola using the
factored form

• Example. Suppose the points (0, 6), (1, 0), and
  (3, 0) are on a parabola, as in a previous
  example. Find a formula for the parabola using
  the factored form. Since the parabola has
  x-intercepts at x 1 and x 3, its formula
  is Substituting x 0, y
  6 gives 6 3a or a 2. Thus, the equation
  is If we multiply this out, we
  get y 2x28x6, which is the same result as
  before.

28
Two methods for finding the zeros of a quadratic

• The first method involves completing the square.
  Suppose we want the roots of x2 3x 2 0. If
  we complete the square as before, we get (x
  1.5)2 0.25 0. If we rewrite this as (x
  1.5)2 0.25, we can take the square root of both
  sides of the equation to get x 1.5 0.5,
  which gives x 1 and x 2.
• The other method involves the use of the
  quadratic formula, which was presented in a
  previous slide lecture. If we apply the
  quadratic formula to x2 3x 2 0, we
  get This reduces
  to x 1.5 0.5 . Again, x 1 and x 2.

29

• What does it mean if a quadratic does not have
  real zeros? It means that the graph of the
  corresponding parabola does not cross the x-axis.
• Problem. If we have 4 feet of string, what is
  the rectangle of largest area which we can
  enclose with the string? Solution. If we let
  one side of the rectangle have length x, then the
  other side must have length (42x)/2. That is,
  the other side is 2x. Therefore, the area of
  the rectangle is a(x) x(2x) x22x. If we
  write this in vertex form, we have a(x)
  (x1)21. Thus, the vertex is at (1, 1), and
  the rectangle of maximum area is a square with a
  side length of 1 foot.

30
Summary for Transformation of Functions and their
Graphs

• If y g(x) is a function and k is a constant,
  then the graph of y g(x) k is the
  graph of y g(x) shifted vertically by k
  units.
• If y g(x) is a function and k is a constant,
  then the graph of y g(xk) is the graph
  of y g(x) shifted horizontally by k units.
• A function is called an even function if, for all
  values of x in the domain of f, f(x) f(x). The
  graph of an even function is symmetric across the
  y-axis.
• A function is called an odd function if, for all
  values of x in the domain of f, f(x) f(x).
  The graph of an odd function is symmetric about
  the origin.

31
Summary for Transformation of Fcts and their
Graphs, contd

• When a function f(x) is replaced by kf(x), the
  graph is vertically stretched or compressed and
  the average rate of change on any interval is
  also multiplied by k. If k is negative, a
  vertical reflection about the x-axis is also
  involved.
• When a function f(x) is replaced by f(kx), the
  graph is horizontally stretched or compressed by
  a factor of 1/k and, if k lt 0, reflected
  horizontally about the y-axis.
• A quadratic function has a formula in either
  standard form or vertex form. Completing the
  square converts standard to vertex form. Vertex
  form is used to find the max or min value of the
  quadratic function. A quadratic function has 0,
  1, or 2 real zeros. If a quadratic function has
  real zeros, it can also be represented in
  factored form. Methods for finding the formula
  for a quadratic function from given data points
  were discussed.