Measures of Center Mean

1
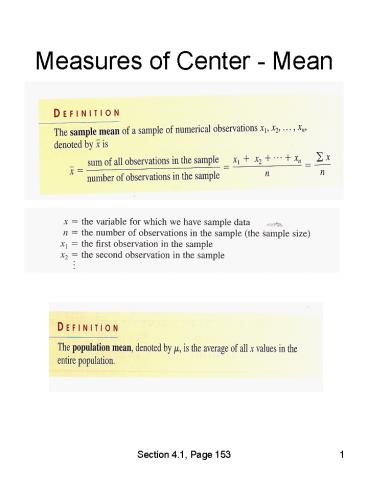
Measures of Center - Mean
2
Measures of Center - Median
CalculationArrange data in ascending
ordern40, even so the median is the average of
the two middle numbers, the 20th and 21st
numbers (1313)/2 13 TI-83 CalculationStat-E
dit Enter data in a listStat-Calculate 1
1Var Stats Enter2nd List - Enter
3
Mean and Median
Median divides the area in halfMean is the
balance point
Mean Median in symmetrical distributionTail
drags mean away from median in skewed
distributions Mean very sensitive to
outliersMedian is notFor distributions with
outliers, the median should be used as the
measure of center
4
Problems
5
Deviations From the Mean
The sum of deviations is always zero so the
average deviation is always zero. It is not
useful a a measure of variability.
6
Standard Deviation
Example Set 1, 2, 3, 4, 5 Mean 3
n5 Observation Deviation Deviation2 1
1-3-2 4 2 2-3-1 1 3
3-30 0 4 4-31 1 5
5-32 4 Total 0
10 Variance s2 10/(5-1)10/42.5Standard
Deviation s sqrt(2.5) 1.5811 TI-83
Stat-Calc-1-Var-Stats Sxsample St. Dev. (The
population standard deviation is sx )
7
Interquartile Range
The interquartile range is not sensitive to
outliers so it is a better measure of variability
than the standard deviation which is sensitive to
outliers
8
Interquartile RangeHospital Cost to Charge Ratio
Hospital Cost-to-Charge Ratios
Interquartile range (iqr) 3Q 1Q 76 -62 14
TI-83 Stat-Edit List , Enter the
data Stat-Calc-1 1-Var Stats Enter2nd List
EnterDown Arrow to Q1 (First Quartile) and Q3
(Third Quartile). IQR3Q-1Q
9
Box PlotsHospital Cost to Charge Ratio
TI-832nd Statplot Enter Highlight On Right Arrow
to 4th Type, Highlight Enter Data List Zoom
9ZoomStat Trace Key and arrows to see values
10
Outliers
Golden Rectangles
Mild Cutoffs upper 3Q 1.5IQR .7935
lover 1Q 1.5IQR .4935 Extreme Cutoffs
upper 3Q 3IQR .906 lower 1Q
3.IQR .3810
11
Problems

• Find the mean and median and standard deviation
• Find the values of the quartiles, iqr and
  standarddeviation.
• Make a modified box plot
• Identify any outliers
• Make a histogram
• Describe the distribution shape, center, and
  spread