Inversion Formula for Laplace Transforms

1
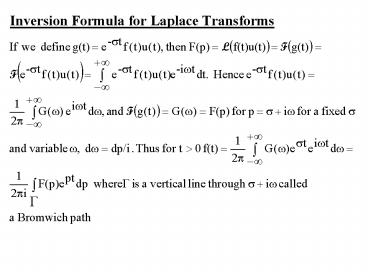
Inversion Formula for Laplace Transforms
2
If f(z) is analytic in a region R R1 lt z z0
lt R2 then
For a proof we follow the book adding a few
details. For example the integral equation
above Fig. 75 on page 192 is obtained by
considering the following diagram.
3
(No Transcript)
4
(No Transcript)
5
Thus substituting (7) (8) into (6) we get
6
Next we show a proof of the first part of (11).
First recall that
7
Example 1 on page 195 has an important integral.
For any closed contour C that encloses z 0
We now make observations about convergence of
power series.
Next we discuss uniform convergence. As
motivation note that in many practical problems  
__________________________________________________
_ This example shows an important application of
Laurent series
8
When we first encountered convergence we had a
sequence of
However when we examine a sequence of functions
fn(x) with a limit f(x) we have a new
possibility as fn(x)?f(x) is a function of x
but e is still a constant and it is possible that
we might need a different N for each x. If on
the other hand we find that there is an N that
works for all x then we say the convergence is
uniform. Here we are interested in infinite
9
Another special type of convergence is absolute
convergence. That is if converges
we say that it also converges absolutely if
converges. Now by the triangle inequality
absolute convergence implies convergence. But
for real numbers the converse is not always true.
For example
10
Absolute convergence Theorem
Uniform convergence Theorem
11
An immediate application of the uniform
convergence result is that it allows us to prove
that you can "differentiate under the summation
sign." Namely
A DIGRESSION THE GAUSSIAN
12
In the above, integrals are all real integrals.
But if we have and we let z xb, then we have
a complex integral where G is the path through
the complex number b and parallel to the real
axis as shown in the diagram below.
13
Now convert to a closed contour C by LK(-G)K'L'

-G
K'
K
Im
b
L'
L
l
l
14
(No Transcript)
15
This Fourier Transform pair is unusual in that
both the time function and the frequency spectrum
have the same functional format, i.e., they
are both gaussians. Note that the width of
is proportional to 1/a. If the gaussian
is narrow in the time domain, it is wide in the
frequency domain.
16
RESIDUES(page 221)Digression Recall ( pages
90-94) that the book uses Log (z) to denote the
principal branch of the natural logarithm. Log
(z) is analytic everywhere except on the branch
which is the oigin plus the negative real
axis,i.e., write z as reiq where 0 lt r ?p lt q
lt p , then Log z is analytic when 0 lt r ?p lt q
lt p.
17
(No Transcript)
18
Finding residues may be difficult Example 4 given
on p.224
19
(No Transcript)
20
We consider 3 types of isolated singularities.
First recall that
21
The next type is called removable, and it occurs
when all the bn are zero for n gt 1. In this case
the function will be analytic at z0 if we define
f(z) to be a0 at z0 ,eg., (sin z)/z at z
0,should be defined as 1 as f(z) (1/z) z
z3/3! z5/5! . . . 1 z2/3! z4/5!
. . . .The last type where all the bn are
non-zero is called essential we shall not
discuss this type.