Correlation Dimension dc

1
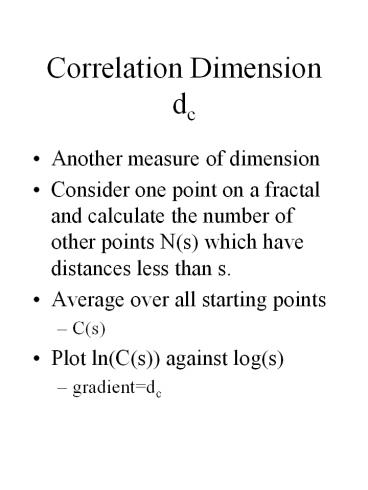
Correlation Dimensiondc

• Another measure of dimension
• Consider one point on a fractal and calculate the
  number of other points N(s) which have distances
  less than s.
• Average over all starting points
• C(s)
• Plot ln(C(s)) against log(s)
• gradientdc

2
Example

• Henon Map -
• xn1a-xn2byn
• yn1xn
• dc1.21
• Notice that the strange attractor in the Henon
  Map while it has structure at all length scales
  is not exactly self-similar

3
(No Transcript)
4
Definitions of dimension

• Two definitions so far
• dF - the number of boxes need to cover fractal
• dc - number of points within a given distance on
  fractal
• Question
• is dcdF ?
• Very often no!

5
When do the two dimensions agree ?

• For exactly self-similar fractals like the
  Sierpinski triangle
• dCdF

6
When do they not - strange attractors

• Eg. The logistic map at ac
• xax(1-x)
• dc0.498
• dF0.537
• So, in this case these two dimensions are not
  equal!
• Same is true for Henon.

7
MultiFractals

• For most real world fractals dc is not equal
  to dF !
• Strange attractors fall into this category eg.
  logistic map
• These attractors have structure at all length
  scales but are not exactly self-similar.
• Called multifractals

8
Examples of multifractals

• Diffusion limited aggregation or DLA
• grow a crystal by allowing molecules to move
  randomly until they stick to substrate
• stick preferentially near tips of growing
  structure
• (multi)fractal
• In 2D (correlation) fractal dimension DLA cluster
  is dF1.7..
• i.e massL1.7

9
(No Transcript)
10
Viscous fingering

• Similar problem
• two miscible liquids (gelatin and water),
  DLA-like structure appears when mixed carefully.
• Low surface tension
• immiscible liquids (water and oil) fingers are
  wider
• tension is large
• For oil recovery - add soap to lower surface
  tension - allows water to penetrate shales and
  flush out oil ...

11
(No Transcript)
12
Fractals in Nature

• Coastline of Norway
• Fjords of all sizes !
• Length of coastline depends on scale at which we
  look
• count how many boxes the outline of the coast
  penetrates
• see dF1.52!
• Scales from 30,000 km to 2500 km
• Bronchial tree.

13
(No Transcript)
14
(No Transcript)
15
Explanation

• It looks fractal - but how do we know for sure
  ...
• A single tube of diameter D splits into 2 tubes
  of diameter d
• 2(d/D)31 approx.
• Remember Cantors set .
• Dln(2)/ln(3)
• or .. 2(1/3)D1
• fractal with dimension D3!
• Space-filling!

16
Fractal dimensionfor multifractals

• For exact fractal
• NrD1
• Nnumber of pieces
• rlength of each
• Generalize
• eg. At each iteration split into 2 pieces but
  with different lengths r1 and r2
• r1Dr2D1