Triangle Sum Conjecture

1
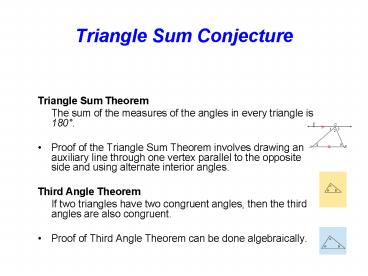
Triangle Sum Conjecture

• Triangle Sum Theorem
• The sum of the measures of the angles in every
  triangle is 180.
• Proof of the Triangle Sum Theorem involves
  drawing an auxiliary line through one vertex
  parallel to the opposite side and using alternate
  interior angles.
• Third Angle Theorem
• If two triangles have two congruent angles, then
  the third angles are also congruent.
• Proof of Third Angle Theorem can be done
  algebraically.

2
Properties of Special Triangles

• Parts of an Isosceles Triangle
• Vertex angle, base angles, legs, and base
• Isosceles Triangle Theorem and Converse
• A triangle is isosceles if and only if the base
  angles are congruent.
• Equilateral triangles are also equiangular, with
  each angle measuring 60 degrees.

3
Triangle Inequalities

• Triangle Inequality Conjecture
• The sum of the lengths of any two sides of a
  triangle is greater than the length of the third
  side.
• Given the length of two sides, the length of the
  third side must be between the sum and difference
  of the given sides.
• Side-Angle Inequality Conjecture
• In a triangle, if one side is longer than
  another side, then the angle opposite the longer
  side is larger than the angle opposite the
  shorter side.
• Exterior angle, adjacent interior angle, and
  remote interior angles
• Triangle Exterior Angle Conjecture
• The measure of an exterior angle of a triangle
  is equal to the sum of the remote interior angles.

4
Triangle Congruence Rules

• There are six sets of three measurements
  associated with triangles that can be used to
  show congruence.

Four that work
Two that dont
SSS (side-side-side)
ASA (angle-side-angle)
AAA (angle-angle-angle)
SAS (side-angle-side)
SAA (side-angle-angle)
SSA (side-side-angle)
5
Corresponding Parts of Congruent Triangles

• Corresponding Parts of Congruent Triangles are
  Congruent
• Acronym CPCTC
• Often appears as the last step in a proof
• By proving triangles are congruent, you can show
  that corresponding sides and/or angles are
  congruent
• Use information marked on a diagram, or
  relationships between parts of a diagram
• It may be necessary to add an auxiliary line to
  establish congruence

6
Two Column Proofs

• A proof is a series of statements with supporting
  reasons which show that a specific fact is true
• Most proofs are constructed as follows
• First, list the given or marked information
• Identify triangles that should be proven
  congruent
• Add statements (and reasons) that identify
  congruent parts
• State which triangles are congruent, making sure
  to list the vertices in the proper order
• Use CPCTC to show that the desired parts are
  congruent
• The final statement should match the fact that
  was to be shown

7
Isosceles Triangle Conjectures

• In a scalene triangle, the median, angle
  bisector, and altitude are separate segments
• In an isosceles triangle, the median, angle
  bisector, and altitude to the vertex angle are
  the same segment
• This segment is the line of symmetry of an
  isosceles triangle
• An equilateral triangle has three such segments