Sum Difference of cubes 5'6b

1
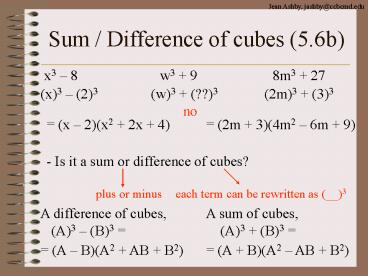
Sum / Difference of cubes (5.6b)
x3 8 w3 9
8m3 27
(x)3 (2)3
(w)3 (??)3 no
(2m)3 (3)3
(x 2)(x2 2x 4)
(2m 3)(4m2 6m 9)
- Is it a sum or difference of cubes?
plus or minus
each term can be rewritten as (__)3
A difference of cubes, (A)3 (B)3
A sum of cubes, (A)3 (B)3
(A B)(A2 AB B2)
(A B)(A2 AB B2)
2
Sum / Difference of cubes (5.6b)
When factoring cubes, follow these steps.
8x3 27
Rewrite as two cubes.
(2x)3 (3)3
Set up the two sets of parentheses.
( ___ ___ )( ___ ___ ___ )
2x 3
4x2
9
6x

What is cubed goes in the first set of
parentheses.
Square the first term. Put it first in the 2nd
set of parentheses.
Square the last term. Put it last in the 2nd set
of parentheses.
Multiply the two terms together. Put it in the
middle.
The sign from the problem goes in the 1st set.
The opposite sign goes next and the last sign is
always positive.