Computing Empirical Probability

1
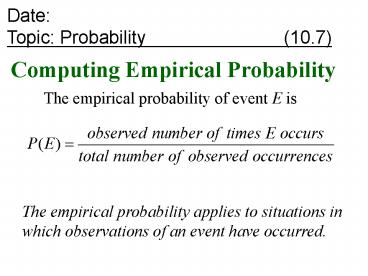
Computing Empirical Probability
DateTopic Probability
(10.7)
The empirical probability of event E is
The empirical probability applies to situations
in which observations of an event have occurred.
2
Example

• An American is randomly selected. Find the
  probability of that person getting 6 hours sleep
  on a typical night.

3
Example cont.

• The empirical probability of randomly selecting
  an American who gets six hours sleep in a typical
  night is 275/1100 or 0.25 or 25.

4
Computing Theoretical Probability
If an event E has n(E) equally-likely outcomes
and its sample space S has n(S) equally-likely
outcomes, the Theoretical probability of event
E, denoted by P(E), is
The sum of the theoretical probabilities of all
possible outcomes in the sample is 1. Theoretical
probabilities applies to experimental situations
containing equally- likely outcomes.
A die is rolled. Find the probability of getting
a number less than 5.
5
Text Example
A die is rolled. Find the probability of getting
a number less than 5.
The equally likely outcomes is S 1, 2, 3, 4,
5, 6, so
n(S) 6
The outcomes of getting a number less than 5 is E
1, 2, 3, 4, so n(E) 4
The probability of rolling a number less than 5 is
6
Example

• What is the probability of getting at most 2
  heads when a coin is tossed 3 times?

7
Example

• What is the probability of getting at most 2
  heads when a coin is tossed 3 times?

8
Example

• What is the probability of getting at most 2
  heads when a coin is tossed 3 times?

The probability of getting at most 2 heads when a
coin is tossed 3 times is 7/8
9
The Probability of an Event Not Occurring

• The probability that an event E will not occur is
  equal to one minus the probability that it will
  occur.
• P(not E) 1 - P(E)

What is the probability of not getting at most 2
heads when a coin is tossed 3 times?
10
Or Probabilities with Mutually Exclusive Events
DAY 2
If A and B are mutually exclusive events, then
P(A or B) P(A) P(B)
If one card is randomly selected from a deck of
cards, what is the probability of selecting a
king or a queen?
P(king or queen) P(king) P(queen)
The probability of selecting a king or a queen is
2/13.
11
Or Probabilities with Events That Are Not
Mutually Exclusive

• If A and B are not mutually exclusive events,
  then
• P(A or B) P(A) P(B) P(A and B)

The figure illustrates a spinner. It is equally
probable that the pointer will land on any one of
the eight regions, numbered 1 through 8. If the
pointer lands on a borderline, spin again. Find
the probability that the pointer will stop on an
even number or a number greater than 5.
12
It is possible for the pointer to land on a
number that is even and greater than 5. Two of
the numbers, 6 and 8, are even and greater than
5. These events are not mutually exclusive. The
probability of landing on a number that is even
and greater than 5 is
The probability that the pointer will stop on an
even number or on a number greater that 5 is 5/8.
13
And Probabilities with Independent Events

• If events A and B are Independent, then the
  probability of A and B is simply

P(A and B) P(A) P(B)
A wheel has 38 outcomes and 18 are red. What is
the probability of a red occurring twice in a
row?
P(red and red) P(red) P(red)
What is the probability of a family having 4
girls in a row?
P(4 girls in a row)
14
Probability