Prsentation PowerPoint

1
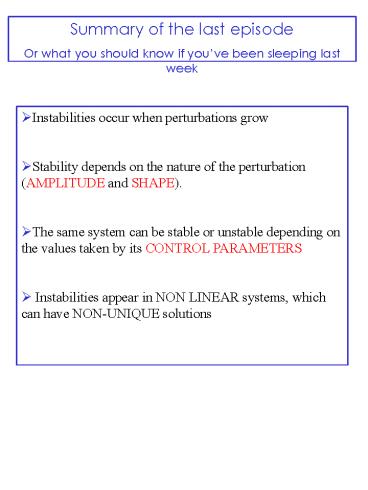
Summary of the last episode Or what you should
know if youve been sleeping last week

• Instabilities occur when perturbations grow
• Stability depends on the nature of the
  perturbation (AMPLITUDE and SHAPE).
• The same system can be stable or unstable
  depending on the values taken by its CONTROL
  PARAMETERS
• Instabilities appear in NON LINEAR systems,
  which can have NON-UNIQUE solutions

2

• Chapter 2 The linear stability
• theory
• 1. General principles
• 2. Electromagnetic pinch effect
• 3. More complex examples
• 4. Hartmann layers, or where it all goes wrong

3
1.1. The problem

• Chapter 1 systems can be stable or unstable.
• For which values of the relevant parameters are
  they unstable?
• What will be the shape of the growing
  perturbations, if any?
• How quickly do they develop?
• Need to have a go at the full non-linear
  equations governing the system dynamics. !!

4
1.2. The basic ideas
Sytsem determined by n relevant parameters
Equilibrium
ASSUMPTION At the onset, the perturbation grows
from zero
infinitesimal
linearisation around equilibrium
(Methdod can be generalised when )
5
1.3.Normal modes analysis
A, B Linear operators / ABBA ?A and B have a
common basis of eigenvectors

• TIME
• If then eigenvect From

Can be decomposed into a countable sum of normal
modes

• SPACE
• If then

Rem

• ? is complex !
• k is imaginary (no local instability)
• a vanishes NO INFORMATION ON THE PETURBATIONS
  AMPLITUDE CAN BE OBTAINED

Dispersion relation
gives the time evolution of each mode k
6

Anatomy of a Normal mode
In 2 dimensions x and Y at time t
At one point (x,y)
Phase velocityspeed of wave crests
Group velocitySpeed of energy
Exponential Growth rate For fixed
Neutral stability
7
Consequence on stability
Marginal stability ? Depends on ( the
dispertion relation)
the Marginal stability curve
Example one parameter R (convection in a
container, Rayleigh number) RltRc ?? for all
values of k ?? flow is linearly stable RgtRc
?? for at least one value of k ?? flow is
unstable
Exchange of stabilities
Perturbations either grow or decay, but dont
keep their amplitude (not always the case)
Real-life growing perturbations They are a SUM
of normal modes ? exponential growth never seen
8
2. Electromagnetic pinch effect

Goal to maintain a jet of electrically
conducting fluid within a given radius R0 ,
without walls (contactless confinement).
0
Uniform current density j0 Fluid density ?
(incompressible) magnetic permeability ? Surface
tension ? Assumption Quantities depend only on z
9
2.2. Stability analysis
Base equations (see appendix)
(Momentum budget) (Mass conservation)
(1)
Base solution
Linearised sytem
10
(2)
Form of the perturbation
(3)
Rem amplitudes are complex, (phase shifts
between variables )
injecting (3) into (2)
Eliminating R, we get the dissipation equation
11
Growth rate a given k
Marginally stable, oscillating modes
Unstable modes
Most unstable mode
All k are present there are always unstable
perturbations The system is always unstable
12
2.3. Stabilisation with a longitudinal magnetic
field
Modified Base equations (see appendix)
Base solution unchanged
Linearised sytem
Using the same perturbations as previously
13
(No Transcript)
14
3. Some more complex stability diagrams
The Taylor- Couette problem of 2 rotating
cylinders
Fluid viscosity ? Narrow gap
Relevant parameters

• Radii ratio
• Angular velocities ratio
• Taylor number

15
Taylor-couette instability
16
Taylor-couette instability with azimuthal
pressure gradient One more relevant parameter
Stability diagrams without viscosity (Tinfinity)
Stability diagrams with viscosity
17
4.The Hartmann layer
Fluid viscosity ? Density ? Electrical
conductivity ?
Magnetic field
B
U
Hartmann layer is destabilised by finite
amplitude perturbations
18
Summary Or what you should know if youve been
sleeping during the past hour

• The linear theories cannot give the amplitude of
  the perturbation.
• Use of normal modes only justfied when DLLD
• LS only gives information on the stability of
  the system for CERTAIN TYPES of INFINITESIMAL
  perturbations
• LS Only returns a NECESSARY CONDITION for
  stability, Not always SUFFICIENT.
• Very difficult to perform a linear stability
  analysis for ALL TYPES of perturbations.
• Overlooks transcient growth

19
Appendix basic equations of the
electromagnetically confined jet
20
Hydrostatic balance

Goal to maintain a jet of electrically
conducting fluid within a given radius R0 ,
without walls (contactless confinement).
0
Uniform current density j0 Fluid density ?
(incompressible) magnetic permeability ?
Calculation of the electromagnetic force in as
section of radius R with current j Ampère
theorem, on surface S(r) surrounded by curve C(r)

Electromagnetic force per unit of volume
21
Pressure p in the jet Fluid is in hydrostatic
balance
Along er
Integrating
(surface tension)
The current is conserved between two sections of
the jet of radius R and R0
Rem in fact, one could also account for the
effect of surface tension due to the curvature of
the surface in the azimuthal direction by setting
22
Equation governing the radius of the jet

Mass budget on a slice of the jet between times t
and tdt
Volume variationinwards mass flux-outwards mass
flux
assuming
using Taylor formula, for any function g(z)
expanding, and dividing by 2?Rdzdt
23

Equation for the axial momentum
Budget of momentum on a cynlinder of axis ez
small radius r, in the referencial moving with
the jet
Variation of axial momentum during dt forces
appied to V ?? dt
Using Taylor formula and dividing by ???r²dzdt
()
Budget of mass on the same cylinder
()
Eliminating ur between () and () and assuming
radial hydrostatic balance
24
Addition of a longitudinal magnetic field
Magnetic field is constant, Additional
electromagnetic force
Mass conservation
On a cylinder of small enough radius
25
Additional force only modifies the hydrostatic
pressure
Modified motion equation Momentum budget still
valid
using the new expression for the pressure