Graphs of Composite Trig Functions - PowerPoint PPT Presentation
Title:
Graphs of Composite Trig Functions
Description:
Graphs of Composite Trig Functions Objective: Be able to combine trigonometric and algebraic functions together. TS: Demonstrating understanding of concepts. – PowerPoint PPT presentation
Number of Views:38
Avg rating:3.0/5.0
Title: Graphs of Composite Trig Functions
1
Graphs of Composite Trig Functions
- Objective
- Be able to combine trigonometric and algebraic
functions together. - TS Demonstrating understanding of concepts.
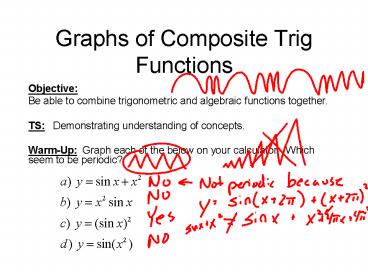
- Warm-Up Graph each of the below on your
calculator. Which seem to be periodic?
2
How can we verify if something is periodic?
- If we believe some function f(x) has a period
of a, then to verify we need to show - f(x a) f(x).
- Example Verify y(sin x)2 is periodic.
3
You Try
- Is y (sin3x)(cosx) periodic? Use your
calculator to figure out what the period is.
4
Graph the following functions one at a time in
the window -2p x 2p and -6 y 6
Which appear to be sinusoids? What
relationship between the sine and cosine
functions ensures their sum or difference is a
sinusoid?
5
Sums that are Sinusoid Functions
- Given the two functions f(x) a1sin(bxc1)
and - g(x) a2cos(bxc2) both with the same b
value then the sum (fg)(x) a1sin(bxc1)
a2cos(bxc2) is a sinusoid with period 2p/b
6
Examples
- Determine whether each of the following
functions is or is not a sinusoid.
7
Putting the two together
- Show that g(x) sin(2x) cos(3x) is periodic
but not a sinusoid.
8
What if I just want to graph some crazy trig
functions? (dont roll your eyes, you know you
want to graph crazy trig functions)
9
- Functions involving the absolute values of Trig
functions - The key is to remember absolute values
create all positive values. - Examples
- f(x) tanx b) g(x) sinx
10
- Functions involving the absolute values of Trig
functions - Examples
- b) g(x) sinx
11
- Functions involving a sinusoid and a linear
function - The key is to remember sine and cosine can
be at most 1 and at least -1. - Examples
- f(x) 3x cosx b) g(x) ½x cosx
12
- Functions involving a sinusoid and a linear
function - Examples
- b) g(x) ½x cosx
13
- Dampened Trig Functions (Trig functions muliplied
by a algebraic function) - The key is to remember sine and cosine can
be at most 1 and at least -1. - Example
- f(x) (2x)cosx