For each translation of the point (

1
Bell Ringer
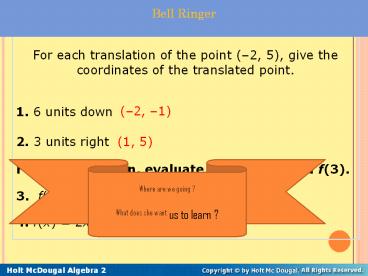
For each translation of the point (2, 5), give
the coordinates of the translated point.
(2, 1)
1. 6 units down
2. 3 units right
(1, 5)
For each function, evaluate f(2), f(0), and f(3).
Where are we going ? What does she want us to
learn ?
3. f(x) x2 2x 6
6 6 21
4. f(x) 2x2 5x 1
19 1 4
2
Horizontal translation

• f(x) a(x h) k

2
reflection across the x-axis and / or a
vertical stretch or compression.
Vertical translation
negative
3
Transformations Quadratic Functions
Objectives F-IF.4, F-IF.6, F-IF.7a
Transform quadratic functions. Describe the
effects of changes in the coefficients of y a(x
h)2 k.
up down
Define, identify, and graph quadratic
functions. Identify and use maximums and
minimums of quadratic functions to solve
problems.
left right
always negative part of formula
(-) (-) (-) ()
4
Transformations Quadratic Functions
Vocabulary
Reference in your textbook
Quadratic Function Parabola
Vertex of a Parabola
Standard Form Vertex Form
Slope Intercept Form Maximum Value vs.
Minimum Value
Due test day September 9, 2014 Test 2 Term 1
5

• Teaching note
• Watch 2-1 video, part 1
• 2) Copy Lab Activities softbook page 8, due
  next class

6
Exit Question
You either need to copy question or answer using
complete sentences. If you copy question, you
may use bullets to answer. Describe the path
of a football that is kicked into the air.
Why? Will the h or k be negative? Hint
creating a graph might be helpful
7
Write Slope Intercept Form of an
Equation Vertex Form of an Equation Standard
Form of an Equation

• Bell Ringer

Challenge yourself to do without notes!
8
2-1 video, part 2, do again today with
pausing
Teaching note
Pause(s) .22, pointing out this is given .36
and ask students how he knows h -1 (negative in
equation and (-)(-) given 1) 2.01 so
students have the option to write down new
function 2.25 and ask students why he added a
in front of K? (part of formula)
9
(No Transcript)
10
Example Translating Quadratic Functions
Use the graph of f(x) x2 as a guide, describe
the transformations and then graph each function.
g(x) (x 2)2 4
Identify h and k.
g(x) (x 2)2 4
h 2, the graph is translated 2 units right. k
4, the graph is translated 4 units up. g is f
translated 2 units right and 4 units up.
11
Example Translating Quadratic Functions
Use the graph of f(x) x2 as a guide, describe
the transformations and then graph each function.
g(x) (x 2)2 3
Identify h and k.
g(x) (x (2))2 (3)
Because h 2, the graph is translated 2 units
left. Because k 3, the graph is translated 3
units down. Therefore, g is f translated 2 units
left and 4 units down.
12
Teaching note

• On next slide point out the 5 is not squared with
  (), so it cannot be the h

13
Example
Using the graph of f(x) x2 as a guide, describe
the transformations and then graph each function.
g(x) x2 5
Identify h and k.
g(x) x2 5
Because h 0, the graph is not translated
horizontally. Because k 5, the graph is
translated 5 units down. Therefore, g is f is
translated 5 units down.
14
Bell Ringer

• Using complete sentence(s), what does each
  indicate about parabola?

2
f(x) a(x h) k
15
Example
Using the graph of f(x) x2 as a guide, describe
the transformations and then graph each function.
g(x) x2 5
Identify h and k.
g(x) x2 5
Because h 0, the graph is not translated
horizontally. Because k 5, the graph is
translated 5 units down. Therefore, g is f is
translated 5 units down.
16
Lets Use a Table, example 1
Evaluate g(x) x2 6x 8 by using a table.

x g(x) x2 6x 8 (x, g(x))
1
1
3
5
7
17
example 1 cont.
Evaluate g(x) x2 6x 8 by using a table,
and calculate the Slope(s).
18
Vertexwhat is it?Its Formula?
Y f
X - b 2a
-b 2a

• Open your textbooks to page 246 and follow along.

19
Y x -2x 3
(1, -4)

• Vertex example, 1

2
Y f(x)
x -b 2a
2
Y (1) 2(1) - 3
x - (-2) 2(1)
Y -4
X 1
20
Y 2x -11x 8
(2.75, -7.12)

• Vertex example, 2

2
Y f(x)
x -b 2a
2
Y 2(11/4) 11(11/4) 8
x - (-11) 2(2)
Y -57 8
X 11 4
21
Y -5x 3x 4
(0.3, -3.55)

• Vertex example, 3

2
Y f(x)
x -b 2a
2
Y -5(3/10) 3(3/10) - 4
x - (3) 2(-5)
Y -71/20
X 3 10
22
Example 1 cont.
Evaluate g(x) x2 6x 8 by using a table,
and calculate the Slope(s), and Vertex.
23
Example 2, Lets Use a Table
Evaluate g(x) x2 3x 11 by using a table.

2
x g(x) x 3x 11 (x, g(x))
3
-1
-0
2
4
24
Example 2 cont.
Evaluate g(x) x2 3x 11 by using a table,
and calculate the Slope(s).
25
Example 2 cont.
Evaluate g(x) x2 3x 11 by using a table,
and calculate the Slope(s), Vertex.
26
Teaching note
Application Activity You are welcome to work
with your peers, but each of you will turn in
your own paper.
MUST COPY soft Common Core workbook pages
40-45. This is not homework will continue to
work on packet tomorrow in class.
27
Exit Question
For each function, evaluate f(2), f(0), and
f(3). Must show work in a table format for
credit.
1. f(x) x2 2x 6
6 6 21
2. f(x) 2x2 5x 1
19 1 4
28
Bell Ringer
Evaluate g(x) -x2 2x 4 by using a table,
and calculate the slope, and Vertex.
x g(x) -x2 2x 4 (x, g(x))
2
-1
0
1
2
29
(No Transcript)
30
Example Reflecting, Stretching, and Compressing
Quadratic Functions
Using the graph of f(x) x2 as a guide, describe
the transformations and then graph each function.
1
(
)
-
2
g x
x
4
Because a is negative, g is a reflection of f
across the x-axis.
31
Example Reflecting, Stretching, and Compressing
Quadratic Functions
Using the graph of f(x) x2 as a guide, describe
the transformations and then graph each function.
g(x) (3x)2
32
Teaching notenext slide

• Students already copied next slide, now they need
  to understand it
• Students need to be able to know if it is the a
  or b, being changed.
• Ask students how do they know if it is the a or b
  being changed?
• They should see it is an a value when x only
  squared it is a b value when there are ( )
  squared.

33
(No Transcript)
34
Activity Group practicefinish pages 40-45
packetdue next class
35
Exit Question
Using the graph of f(x) x2 as a guide, describe
the transformations, and then graph g(x) (x
1)2.
-1 5
g is f reflected across x-axis, vertically
compressed by a factor of , and translated 1
unit left.
1 5