Rough Sets Theory

1
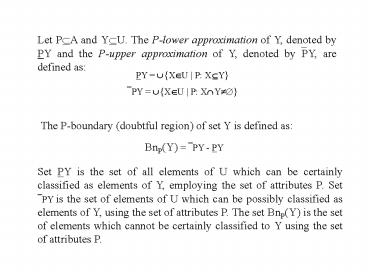
Let P?A and Y?U. The P-lower approximation of Y,
denoted by PY and the P-upper approximation of Y,
denoted by?PY, are defined as
PY ??X?U P X?Y? ?PY ??X?U P X?Y???
The P-boundary (doubtful region) of set Y is
defined as
BnP(Y) ?PY - PY
Set PY is the set of all elements of U which can
be certainly classified as elements of Y,
employing the set of attributes P. Set ?PY is the
set of elements of U which can be possibly
classified as elements of Y, using the set of
attributes P. The set BnP(Y) is the set of
elements which cannot be certainly classified to
Y using the set of attributes P.
2
Undefinable sets

• Set Y is definable in P iff PY ?PY, otherwise
  set Y is undefinable in P.

• If PY ? ? and ?PY ?U, Y will be called roughly
  definable in P.

• If PY ? ? and ?PY U, Y will be called
  externally undefinable in P.

• If PY ? and ?PY ? U, Y will be called
  internally undefinable in P.

• If PY ? and ?PY U, Y will be called totally
  undefinable in P.

3
With every set Y?U, we can associate an accuracy
of approximation defined as
Subsets Yi , i1,...,n are categories of
partition Y. By P-lower (P-upper) approximation
of Y in S we mean sets PY?PY1, PY2, ..., PYn?
and PY??PY1,?PY2, ...,?PYn?, respectively.
The coefficient
is called the quality of approximation of
partition Y by a set of attributes P (quality of
sorting).
4
Name Education Descision Joe High
School No Mary High School Yes Peter Elementary
No Paul University Yes Cathy Doctorate Yes
O Mary, Paul, Cathy A Education R(A)
Joe, Mary, Peter, Paul,
Cathy POS(O) LOWER(O) Paul,
Cathy NEG(O) Peter BND(O) Joe,
Mary UPPER(O) POS(O) BND(O) Paul, Cathy,
Joe, Mary
(Education, University) or (Education, Doctorate)
--gt Good prospects (Education, Elementary) --gt
No good prospects (Education, High School) gt
Good prospects (i.e. possibly)
5
Reducts in Information Systems
Set of attributes P is independent if for every
proper subset Q of P, that is Q ? P,
IND(P) ? IND(Q), otherwise P is dependent (in S).
A subset P ? Q ? A is a reduct of Q (in S) if P
is independent subset of Q and IND(P)  IND(Q).
For every P ? A, RED(P) ? ?, and if P is
independent then RED(P)  ?P?.
An element p ? P is said to be dispensable for P
if IND(P)  IND(P-?p?), otherwise an element p
is indispensable.
The set of all indispensable elements for P is
said to be a core of P and denoted by CORE(P).
6
Let U consist of five elements denoted by t1...t5
and let A  ?a, b, c, d, e?, Va  ?0, 1?,
Vb  ?0, 2?, Vc  ?1, 2, 3?, Vd  ?1, 3?,
Ve  ?0, 1, 2, 3?.
All partitions in this system are ?  ?t1, t2, t
3, t4, t5? a  ??t1, t5?, ?t2, t3, t4?? b   ??t
1, t3?, ?t2, t4, t5?? c   ??t1, t3?, ?t2, t4?, ?
t5?? d  ??t1, t3, t5?, ?t2, t4?? e  ??t1, t5?,
 ?t2?, ?t3?, ?t4?? (ab)  ??t2, t4?, ?t1?, ?t3?, 
?t5?? (ad)  ??t2, t4?, ?t1, t5?, ?t3?? (be)  ?
t1?, ?t2?, ?t3?, ?t4?, ?t5??
The table gives an information function
U a b c d e t1 0 2 1 3 1 t2 1 0 2 1 2 t3 1 2 1 3 3
t4 1 0 2 1 0 t5 0 0 3 3 1
7
This information system has nine different
indiscernibility relations IND(?), IND(a), IND(b)
, IND(c) ( IND(bc)  IND(bd)  IND(cd) 
IND(bcd)), IND(d), IND(e) ( IND(ae)  IND(de)  I
ND(ade)), IND(ab) ( IND(ac)  IND(abc)  IND(abd)
  IND(acd)  IND(abdc)), IND(ad), IND(A) IND(be)
  IND(ce)  IND(abc)  IND(ace)  IND(bce)  
IND(cde) IND(abce) IND(bcde)
IND(acde) IND(abde))
8
To remember

• A rough set is a set defined only by its lower
  and upper approximation. A set, O, whose boundary
  is empty is exactly definable.

• If a subset of attributes, A, is sufficient to
  create a partition R(A) which exactly defines
  set of objects, then we say that A is a reduct.

• The intersection of all reducts is known as the
  core.

9

• Articles
• Rough Classification. Z. Pawlak, Polish Academy
  of Sciences (1983)
• Reducts in Information Systems. C. M. Rauszer,
  Uof Warsaw (1991)
• Variable Precision Extension of Rough Sets. J. D.
  Katzberg, W. Ziarko, University of Regina,
  Saskatchewan, Canada (1996)
• Rough Concept Analysis a Synthesis of Rough Sets
  and Formal Concept Analysis. R. E. Kent,
  University of Arkansas at Little Rock, USA (1996)

http//www-idss.cs.put.poznan.pl/research/rough_se
ts/index.html http//www.cs.uregina.ca/roughset/