Chapter 4 Continuous Models

1
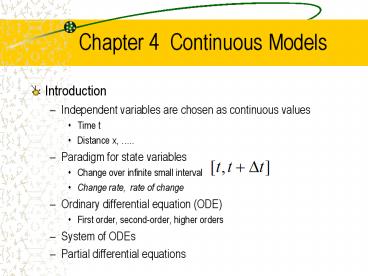
Chapter 4 Continuous Models

• Introduction
• Independent variables are chosen as continuous
  values
• Time t
• Distance x, ..
• Paradigm for state variables
• Change over infinite small interval
• Change rate, rate of change
• Ordinary differential equation (ODE)
• First order, second-order, higher orders
• System of ODEs
• Partial differential equations

2
Malthuss Model

• Thomas R. Malthus (1766-1834) Father of
  population model
• In 1798, An Essay on the Principle of
  population
• Profoundly impact on evolution theory of Charles
  Darwin (1809-1882)
• Malthus's observation was that, unchecked by
  environmental or social constraints, it appeared
  that human populations doubled every twenty-five
  years, regardless of the initial population size.
  Said another way, he posited that populations
  increased by a fixed proportion over a given
  period of time and that, absent constraints, this
  proportion was not affected by the size of the
  population.

3
Malthuss Model

• By way of example, according to Malthus, if a
  population of 100 individuals increased to a
  population 135 individuals over the course of,
  say, five years, then a population of 1000
  individuals would increase to 1350 individuals
  over the same period of time.
• Let
• t time
• N(t) the number of population at time t
• Balance equation

4
Malthuss model

• Consider the time interval

5
Malthuss model

• Malthuss assumption
• Unlimited resource no migration
• Birth rate and death rate are both constants
• The equation

6
Malthuss model

• Phenomena
• Population explosion story of Prof. Yanchu
  Ma
• Population distinction
• No change
• World population

7
The logistic model

• Assumption (Verhulst, 1836)
• Limited resource, no migration death rate is
  constant
• Birth rate decreases with increasing population

8
The logistic model

• The solution
• Phenomena
• Population distinction
• Equilibrium
• Carrying capacity K
• N(t) simply increase monotonically
  to K
• Form a sigmoid
  character slow-fast-slow change
• fast-slow
  change
• N(t) decreases monotonically to K
  

9
(No Transcript)
10
Equilibrium stability

• Consider autonomous ODE
• Equilibrium
• Asymptotically stable
• Conditions
• Stable
• Unstable

11
Equilibrium stability

• Reasons
• For Malthuss model N0
• Stable
• Unstable
• For the logistic model N0 or K
• Stable NK
• Unstable N0

12
Population with Harvesting

• Some examples
• Population of Singapore or USA immigration
• Fish in a pound
• Whale in the ocean
• Big environmental problems
• Fishing grounds collapsed under over-fishing
• Some animals are in danger of extinction due to
  indiscriminate hunting
• Big question harvesting of renewable resources
• Optimal harvest without ruining the
  resource!!!!!

13
Harvest logistic model (I)

• Assumption For fish, plants, etc
• Limited resource death rate is constant
• Harvest depends linearly on the population
• Birth rate decreases with increasing population

14
Harvest logistic model (I)

• We can find the solution, but here we are NOT so
  much interested in the value of N at a specific
  time instant t!!
• We are rather interested in
• The terminal value of N when t goes to infinity??
• ecologists who guard against extinction of animal
  or botanical species
• Scientists in agriculture who have to control
  pests
• Scientists in calculating fishing quotas,
  determine E!!!
• Whether the population will die out in a finite
  period of time??
• Will N tend to a limit value when t goes to
  infinity??
• What is the optimal harvest strategy
• Almost optimal harvest the population can self
  renewable

15
Growth of f(N)
16
Harvest logistic model (I)

• The solution
• Phenomena
• Equilibrium
• Yield or harvest is
• Maximum harvest

17
Harvest logistic model (I)

• Time scale of recovery after harvesting
• No harvest recovery time
• With harvest 0ltEltr
• For fixed r, E increases, the recovery time
  increases
• Since the yield Y that is recorded, express T in
  the yield Y

18
Harvest logistic model (I)

• Optimal harvest strategy

19
Harvest logistic model (II)

• Assumption
• Limited resource death rate is constant
• Harvest fixed amount H per unit time
• Birth rate decreases with increasing population
• With

20
Harvest logistic model (II)

• We are rather interested in
• The terminal value of N when t goes to infinity??
• ecologists who guard against extinction of animal
  or botanical species
• Scientists in agriculture who have to control
  pests
• Scientists in calculating fishing quotas,
  scientist try to choose E in such a way that the
  annual catch is as large as possible without
  diminishing the stock (the maximum sustainable
  yield). Of course, the terminal value depends on
  E !!!

21
Harvest logistic model (II)

• Suppose the limit of N(t) exists,
• Plug into the equation
• It is equivalent
• Solution

22
Harvest logistic model (II)

• When , there
  exists a limit
• When , no
  limit!!
• Population will extinct in a finite time T.

23
Harvest logistic model (II)

• F is a critical value in the sense that a
  harvesting rate which exceeds F must leads to the
  collapse of the stock
• Qualitative analysis
• Two different limiting values

24
Harvest logistic model (II)

• Re-write the equation
• Qualitative graph of the solution
• Case 1 ,
  N(t) decreases near t0 and remains decreasing as
  long as .
• Case 2
• Case 3
  , N(t) increases near t0 and remains increasing
  as long as .
• Case 4
• Case 5
  , N(t) decreases near t0. N(t) must be zero
  within a finite time T (extinction time)

25
Harvest logistic model (II)
26
Harvest logistic model (II)

• Example The sandhill crane Grus canadensis in
  North American
• It was protected since 1916 because it was on the
  endangered list
• Repeated complaints of crop damage in USA and
  Canada led to hunting seasons since 1961.
• These birds will not breed until they are 4 years
  old and normally will have a maximum life span of
  25 years.
• Two USA ecologists, R. Miller D. Botkin studied
  it by constructing a simulation model with ten
  parameters to investigate the effect of different
  rates of hunting on the sandhill crane.

27
Harvest logistic model (II)

• Use our logistic model II and their data,
• Critical hunting rate is F4800 birds per year
• Take the initial value N(0)194,600 which
  is the limit of the logistic model when E0.
• Comparison with Miller Botkin
• Case 1 EgtF

28
Harvest logistic model (II)

• Case 2 EltF
• Our model is more optimistic than the prediction
  of Miller Botkin, and surprisingly near to it!!
• Due to illegal hunters, Miller Botkin plead for
  stricter control on indiscriminate hunting and
  for smaller quotas!!